Assuming that a person of normal sight can read print to such distance that the letters subtend an angle of 5’ at his eye, find the height of the letters that he can read at a distance of 12 metres.
Convert 5' into degrees
=560 degrees.
Now replace it in the equation tan(560)=PQ12
PQ=12⋅tan(112)
=12⋅0.00145m
=0.0174m
=1.7cm
Topper's Solved these Questions
Similar Questions
Assuming that a person of normal sight can read print to such distance that the letters subtend an angle of 5’ at his eye, find the height of the letters that he can read at a distance of 12 metres.
View SolutionAssuming that a person of normal sight can read print at such a distance that the letters subtend an angle of 5' at his eye. Find what is the height of the letters that he can read at a distance of 756 meteres ?
View Solution
Knowledge Check
A person cannot see distinctly at the distance less than one metre. Calculate the power of the lens that he should use to read a book at a distance of 25 cm.
A+ 3.0.DB+ 0.125 DC-3.0 DD+ 4.0 DA tree of height 100 feet subtends a right angle at the top of another tree.If the height of the other tree is 64 metres then the distance between the two trees is
A48 mB36 mC54 mD72 mA short sighted person can see objects most distinctly at a distance of 16 cm. If he wears spectacles at a distance of 1 cm from the eye, then their focal length to see distinctly at a distance of 26 cm
A25 cm, convexB25 cm, concaveC37.5 cm, convexD37.5 cm, concave
Find the length which at a distance of 5280 m will subtend an angle of 1' at the eye.
View SolutionA flagstaff stands vertically on a pillar, the height of the flagstaff being double the height of the pillar. A man on the ground at a distance finds that both the pillar and the flagstaff subtend equal angles at his eyes. The ratio of the height of the pillar and the distance of the man from the pillar is
View SolutionAt what distance does a man 512 ft. in height, subtend an angle of 15" ?
View SolutionA normal human cye can see an object making an angle of 1.8∘ at the eye. What is the

approximate height of object which can be seen by an eye placed at a distance of 1 m from the object.
View SolutionRead the graph and find the height of Tarun when he was of 5 years?
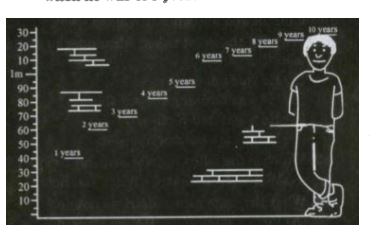
View Solution
