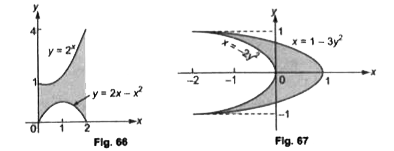
Compute the area of the figure bounded by the parabolas x=−2y2,x=1−3y2
Text Solution
The correct Answer is:43
|
Topper's Solved these Questions
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Areas with Parametrically Represented Boundaries
7 Videos
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - The Area of a Curvilinear Sector in Polar Coordinates
5 Videos
- APPLICATIONS OF THE DEFINITE INTEGRALBOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Finding Average Values of a Function
15 Videos
- APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSBOOK - IA MARONCHAPTER - APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSEXERCISE - ADDITIONAL PROBLEMS
9 Videos
- BASIC CLASSES OF INTEGRABLE FUNCTIONS BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS
5 Videos
Similar Questions
Knowledge Check
Find the area of the figure bounded by the parabolas x=−2y2,x=1−3y2.
A83B63C43D23
Find the area of the figure bounded by the parabolas x=−2y2,x=1−3y2.
View SolutionThe area of the region bounded by the parabolas x=−2y2 and x=1−3y2 is
View SolutionCompute the volume of the solid generated by revolving about the y-axis the figure bounded by the parabolas y=x2and8x=y2
View SolutionFind the area bounded by the parabola y=x² and y2=x.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region bounded by the parabola y=x2 and y=|x| .
View SolutionFind the area of the figure bounded by the parabola y=−x2+2x+3, the line tangent to it at the point M (2,−5) and the y-axis
View Solution
