Consider a family of straight lines . Find the equation of the straight line belonging to this family that is farthest from Solution in Tamil
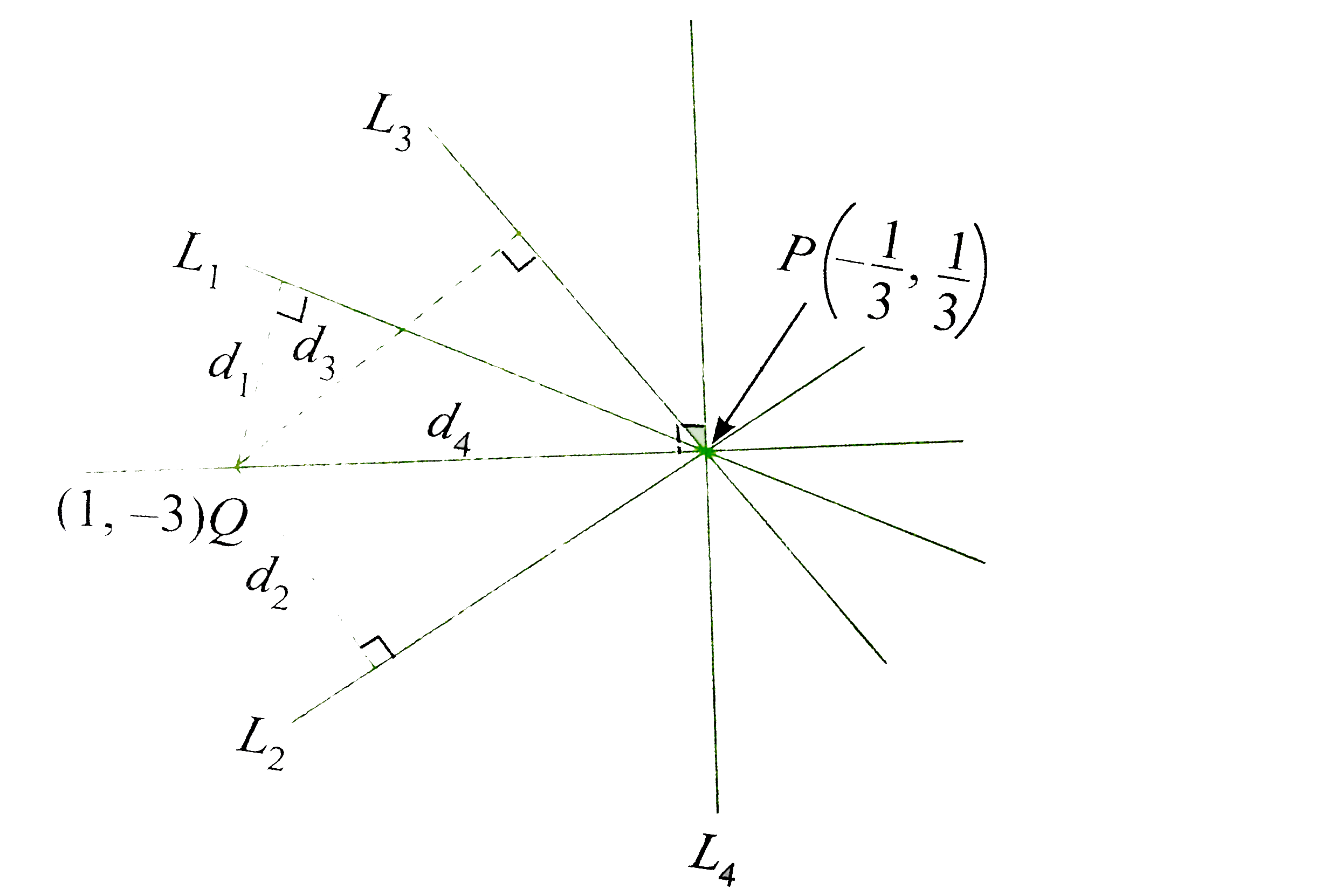
Given lines are concurrent at point of intersection of lines .
We require a line through point P which is farthest from point Q(1,-3), i.e., line whose perpendicular distance from point P is the greatest.
We observe that perpendicular distances ..... of the lines ....., respectively, from point Q are less than distance of the line Q. Line is perpendicular to the segment joining P and Q. Line is the greatest distance from point Q.
Therefore, equation of the required line is
or 6x-15y+7 = 0
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.1
23 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
Find the equation of the right bisector of the line segment joining the points (3,4) and (−1,2).
View SolutionFind the points on the line x + y = 1 that lie at a distance 3 units from the line 5x+12y = 3.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionConsider a family of straight lines (x+y)+λ(2x−y+1)=0 . Find the equation of the straight line belonging to this family that is farthest from (1,−3).
View SolutionConsider a family of straight lines (x+y)+λ(2x−y+1)=0 . Find the equation of straight line belonging to this family that is farthest from (1;-3)
View SolutionConsider the family of lines 5x+3y−2+λ1(3x−y−4)=0 and x−y+1+λ2(2x−y−2)=0. Find the equation of a straight line that belongs to both the families.
View SolutionConsider the family of lines 5x+3y−2+λ(3x−y−4)=0 and x−y+1+μ(2x−y−2)=0 Equation of straight line that belong to both families is ax+by−7=0 then a+b is
View SolutionConsider the family of line (x+y−1)+λ(2x+3y−5)=0 and (3x+2y−4)+μ(x+2y−6)=0 Equation of a straight line that belongs to both the families is:
View SolutionConsider the family of lines 5x+3y−2+λ(3x−y−4)=0andx−y+1+μ(2x−y−2)=0. The equation of a straight line that belonges to both the families is
View SolutionConsider a family of straight lines (x+y)+λ(2x−y+1)=0 . Find the equation of the straight line belonging to this family that is farthest from (1,−3).
View SolutionConsider a family of straight lines (x+y)+λ(2x−y+1)=0 . Find the equation of the straight line belonging to this family that is farthest from (1,−3).
View SolutionConsider the family of lines 5x+3y−2+λ1(3x−y−4)=0 and x−y+1+λ2(2x−y−2)=0. Find the equation of a straight line that belongs to both the families.
View SolutionConsider the family of lines 5x+3y−2+λ1(3x−y−4)=0andx−y+1+λ2(2x−y−2)=0. Equation of a straight line that belong to both families is
View SolutionIf a family of straight line (x+y)+λ(2x−y+1)=0. Find the equation of the staright line belonging to this family that is farthest from (1,-3).
View SolutionConsider the family of lines (x+y−1)+λ(2x+3y−5)=0 and (3x+2y-4)+μ(x+2y−6)=0, equation of a straight line that belongs to both the families is
View Solution
