Consider the following figure.
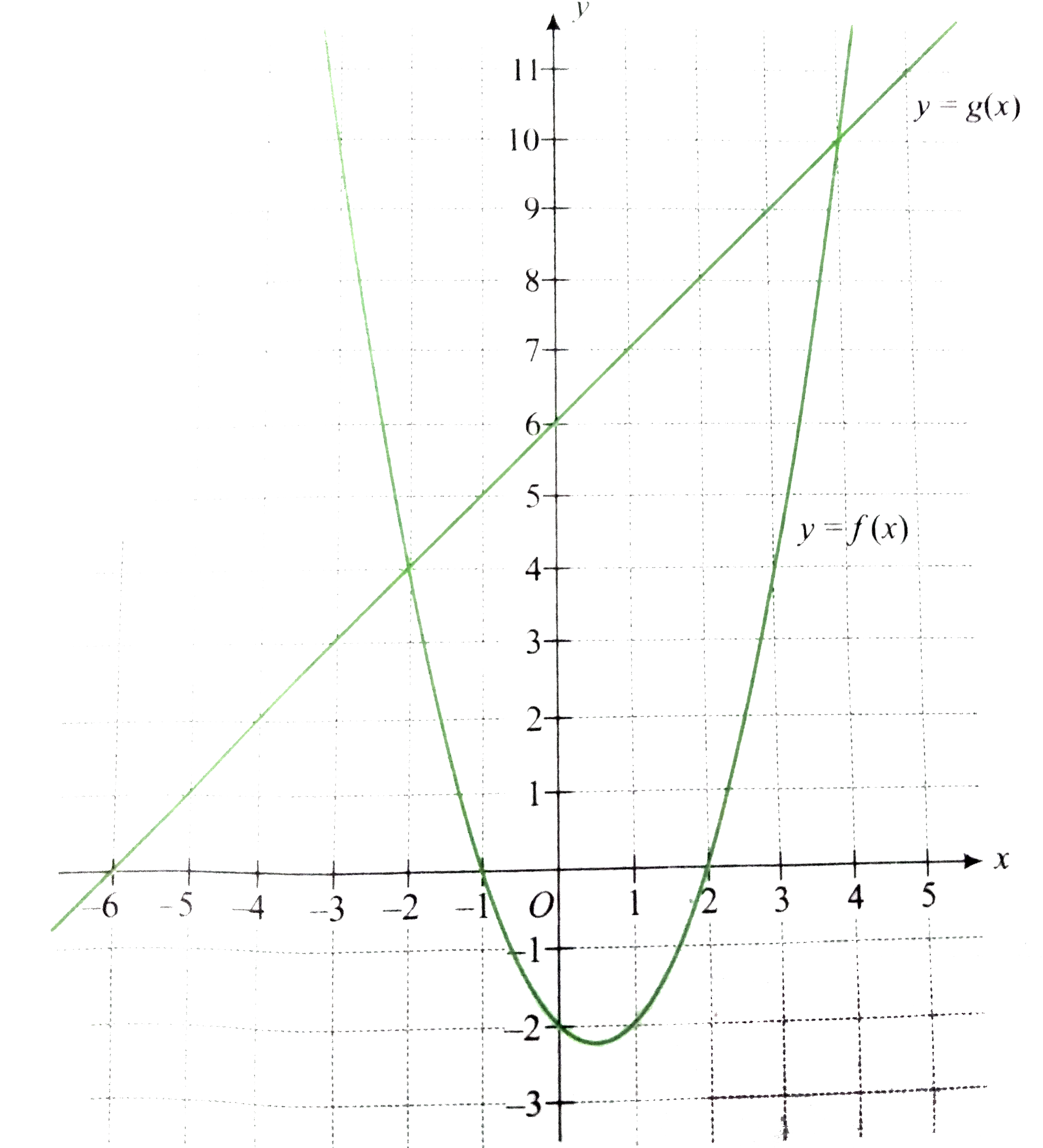
Answer the following questions
(i) What are the roots of the f(x) = 0?
(ii) What are the roots of the f(x) = 4?
(iii)What are the roots of the f(x) = g (x)?
(i) For the roots of the equation f(x) = 0, we check the points of intersection of y = f(x) with x -axis at x = - 1 and x = 2, which are roots of the equation f(x) = 0 .
(ii) For the roors of the equation f(x) = 4, we check the points of intersection of the graphs y = f(x) and y = 4 ( a line parallel to x-axis at height 4 units above it)
The x -values of the poinst of intersection of graphs is x = -2 and x = 3 , which are roots of the equation f(x) = 4.
(iii) For the roots of the equation f(x) = g(x), we check the points of intersection of the graphs y = f(x) and y = g(x).
The x -velues of the points of intersection of graphs are x = -2 and x = 4, which are roots of the equation f(x) = g(x)
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - SOLVED EXAMPLES14videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - CONCEPT APPLICATION EXERCISE 2.13videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - Multiple Correct Answer6videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)1videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions291videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
Consider the graph of y = f(x) as shown in the following figure.
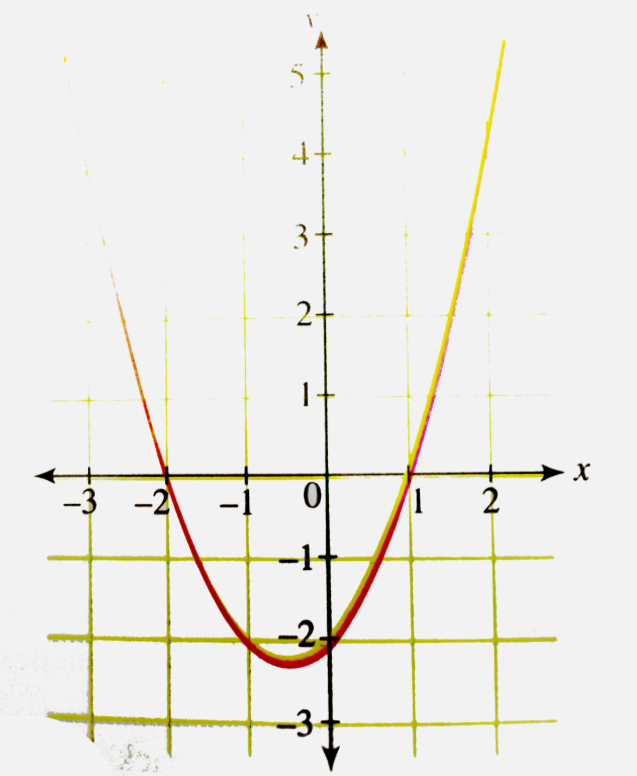
(i) Find the sum of the roots of the equation f (x) = 0.
(ii) Find the product of the roots of the equation f(x) = 4.
(iii) Find the absolute value of the difference of the roots of the equation f(x) = x+2 .View SolutionConsider the graph of y = f(x) as shown in the following figure.

(i) Find the sum of the roots of the equation f (x) = 0.
(ii) Find the product of the roots of the equation f(x) = 4.
(iii) Find the absolute value of the difference of the roots of the equation f(x) = x+2 .View Solution
Knowledge Check
- Question 1 - Select One
The two roots of the equation f(x)=f(x+8x−1) are:
A2,-2B4,-2C−4,−2D2,4 - Question 2 - Select One
Let f(x)=x2+2x−5 and g(x)=5x+30
What are the roots of the equation g [f(x)]=0A1, -1B−1,−1C1, 1D0,1 - Question 3 - Select One
The two roots of the equation f(x)=f(x+8x−1) are :
A2,−2B4,−2C−4,−2D2,4
If f(x) is a quadratic expression such that f(1)+f(2)=0, and −1 is a root of f(x)=0, then the other root of f(x)=0 is :
View SolutionGraph of y=f(x) is as shown in the following figure.
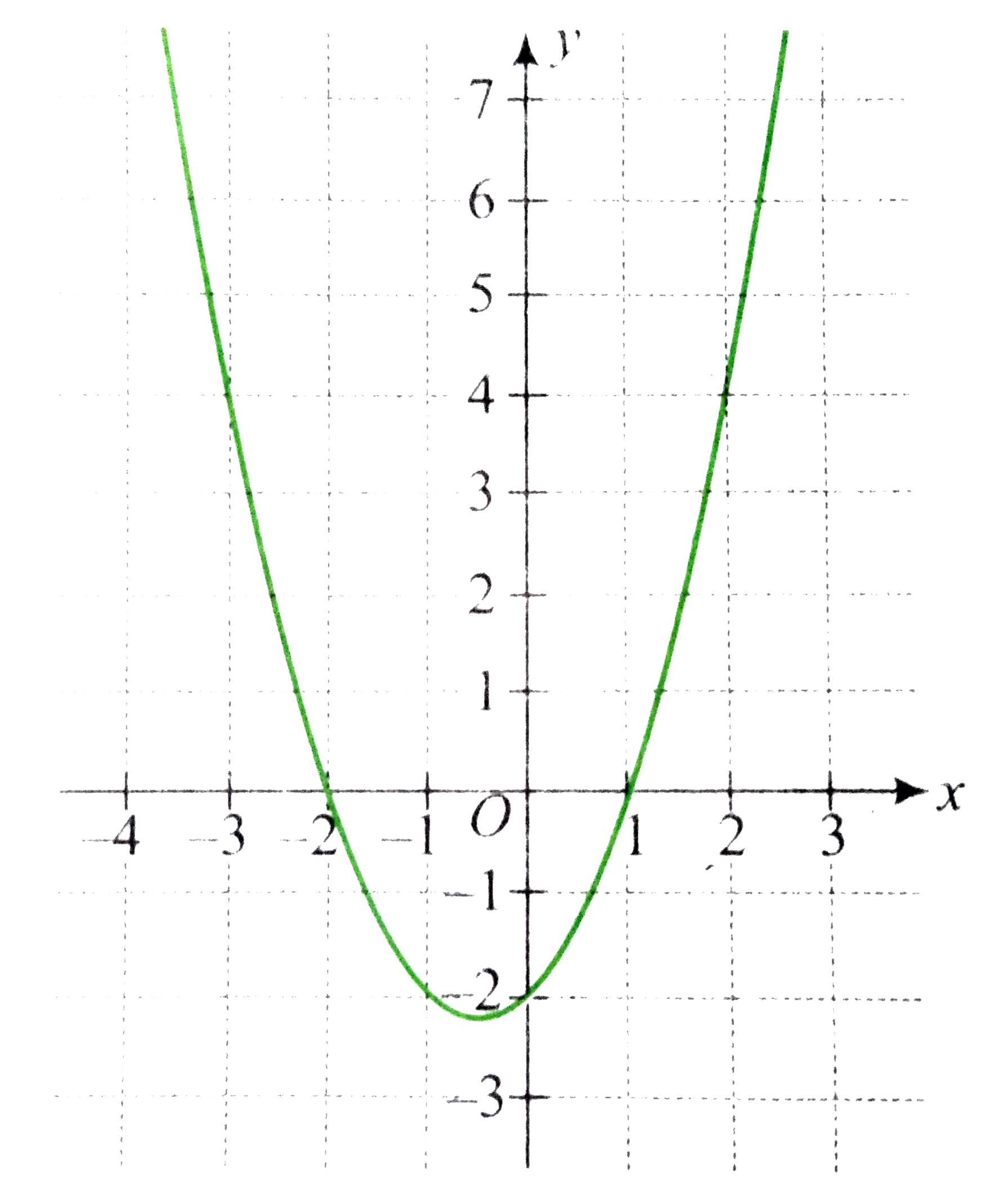
Find the roots of the following equations
f(x)=0
f(x)=4
f(x)=x+2View SolutionGraph of y=f(x) is as shown in the following figure.

Find the roots of the following equations
f(x)=0
f(x)=4
f(x)=x+2View SolutionFollowing is the graph of y = f(x).
<imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/CEN_GRA_C01_S01_005_Q01.pngwh=80%mx-autosty≤=background:white;><br>F∈dthes√oftheequation f(x) = 0, f(x) = 4 and f(x) = 10.View SolutionLet f(x) be a quadratic polynomial satisfying f(2) + f(4) = 0.
If unity is one root of f(x) = 0 then find the other root.View Solution

