Question

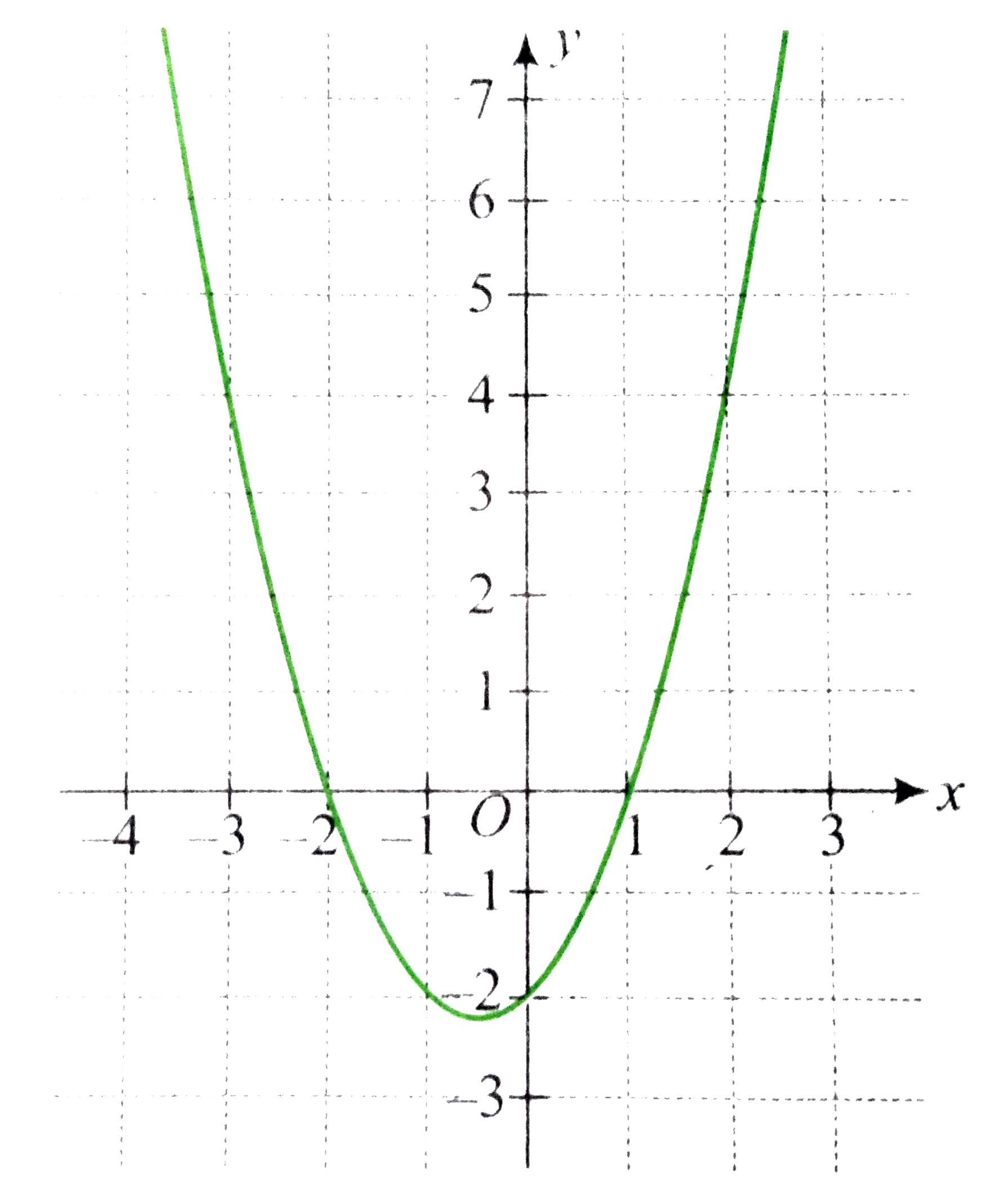
Consider the graph of y = f(x) as shown in the following figure.

(i) Find the sum of the roots of the equation f (x) = 0.
(ii) Find the product of the roots of the equation f(x) = 4.
(iii) Find the absolute value of the difference of the roots of the equation f(x) = x+2 .

Explanation

(i) Roots of the equation f(x) = 0 occur where the graph of y = f(x) and y = 0 intersect.
From the diagram, the points of intersection of intersection are x =- 2 and x= 1. Hence sum of the roots is - 1.
(ii) Roots of the equation f(x) = 4 occur where the graph of y = f(x) and y = 4 intersect. From the diagram, the points of intersection are x =- 3 and x = 2. Hence the product of the roots is -6.
(iii) Roots of the equation f(x) = x+2 occur where the graph of y = f(x) and y = x+2 intersect. From the diagram, the points of intersection are x =- 2 and x = 2. Hence the difference of the roots is 4.