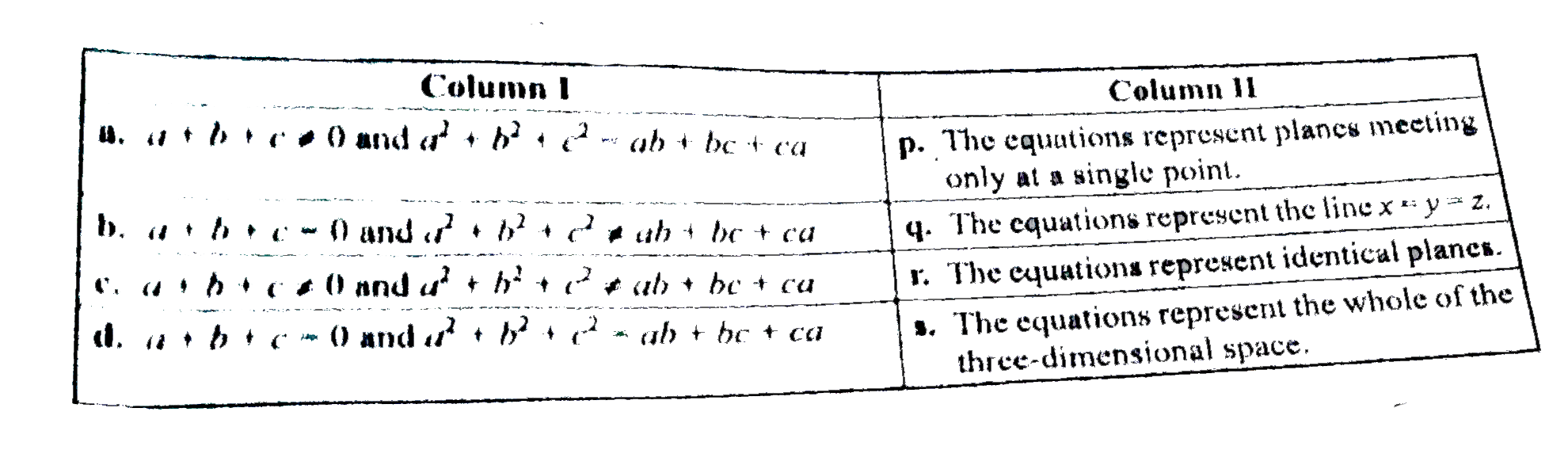
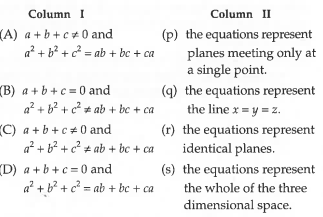
Consider the following linear equations
$a x + b y + c z = 0$
$b x + c y + a z = 0$
$c x + a y + b z = 0$
Match the conditions/expressions in Column-I with statements in Column-II.

Text Solution

Verified by Experts

The correct Answer is: $(d) \to (s)$

|

Updated on:21/07/2023

Class 12 MATHS COORDINATE GEOMETRY

Topper's Solved these Questions

BOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - PROBLEM SET (4)
19videos
CO-ORDINATE GEOMETRY OF THREE DIMENSION
BOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - MISCELLANEOUS EXERCISE(TRUE AND FALSE)
27videos
CO-ORDINATE GEOMETRY OF THREE DIMENSION
BOOK - ML KHANNACHAPTER - CO-ORDINATE GEOMETRY OF THREE DIMENSIONEXERCISE - PROBLEM SET (2)
12videos
CO-ORDINATE GEOMETRY OF THREE DIMENSION
BOOK - ML KHANNACHAPTER - BINOMIAL THEOREM AND MATHEMATICAL INDUCTION EXERCISE - Self Assessment Test
35videos
BINOMIAL THEOREM AND MATHEMATICAL INDUCTION
BOOK - ML KHANNACHAPTER - COMPLEX NUMBERS EXERCISE - Assertion / Reason
2videos
COMPLEX NUMBERS

Similar Questions

Knowledge Check

Question 1 - Select One or More
Three lines $p x + q y + r = 0$ , $q x + r y + p = 0$ and $r x + p y + q = 0$ are concurrent , if
A $Σ a^{3} = 3 a b c$
B $σ a = 0$
C $Σ a^{2} = Σ a b$
DNone
Question 2 - Select One
The three lines $a x + b y + c = 0$ ,
$b x + c y + a = 0, c x + a y + b = 0$
are concurrent only when
A $a + b + c = 0$
B $a^{2} + b^{2} + c^{2} = a b + b c + c a$
C $a^{3} + b^{3} + c^{3} = 3 a b$
DNone of these
Question 3 - Select One or More
If the equations $a x + b y + c z = 0, b x + c y + a z = 0 and c x + a y + b z = 0$ have a non-zero 'solution, then which one of the following is true
A $a + b + c = 0$
B $a = b = 0$
C $(a - b) + {(b - c)}^{2} + {(c - a)}^{2} = 0$
Dnone of these

ML KHANNA-CO-ORDINATE GEOMETRY OF THREE DIMENSION-PROBLEM SET (3)

Home
Ask Doubt
Profile