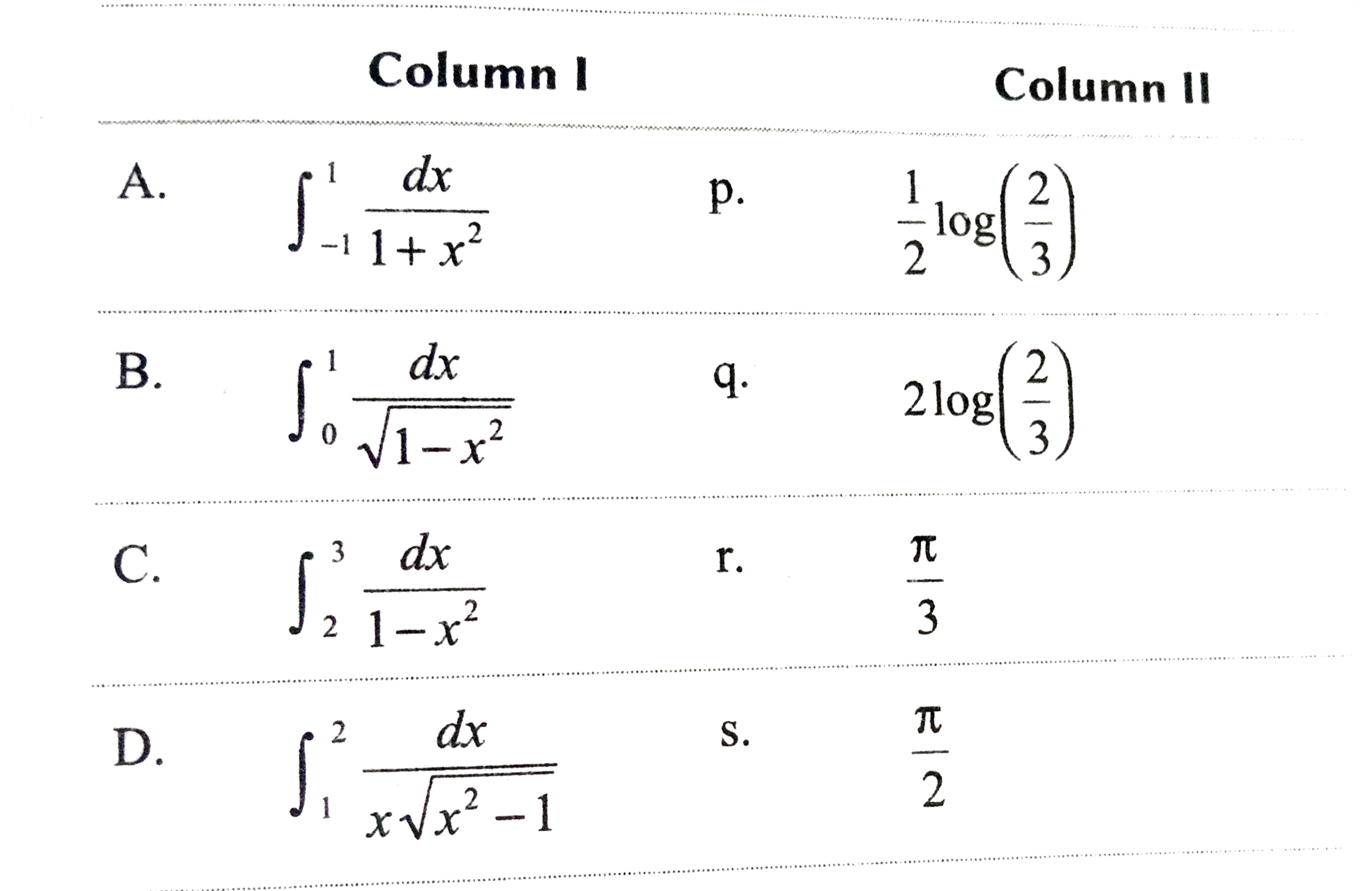
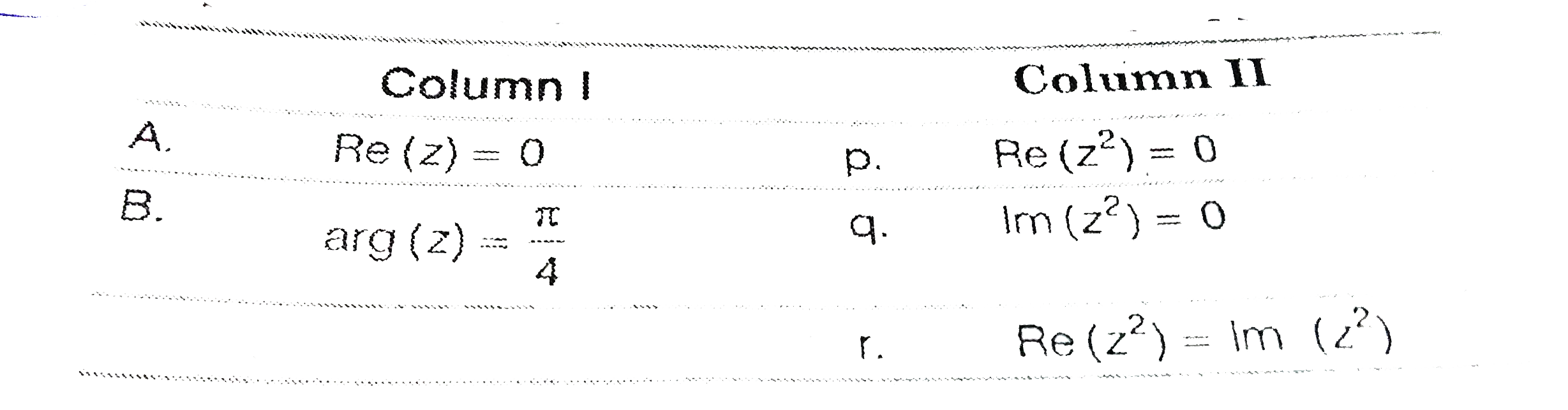
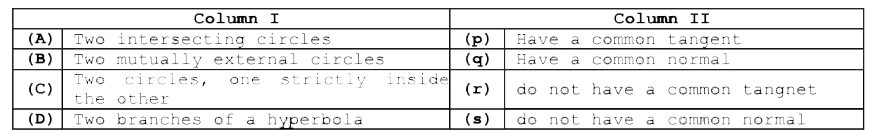Match the conditions /expression n Column I with statement in Column II (z≠0 is a complex number )
Video Solution
Text Solution
The correct Answer is:A→q;B→p
Let z=a+ ib
Given Re(z)=0⇒a=0
Then z=ib⇒z2=−b2orlm(z2)=0
Therefore sdsA→q
Also, given ,arg (z)=π2
Let z=r(cosπ4−Isinπ4)
Then z2r2(cos2π4−sin2Lπ4)+2ir3π4sinπ4
=ir2sinπ/2=ir2
Therefore , Re(z2)=0⇒B⇒p.
⇒a=b=2−√3 [∵a,b←(0,1)]
|
Topper's Solved these Questions
- COMPLEX NUMBERSBOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 3 ARGUMENT OF A COMPLEX NUMBER (OBJECTIVE QUESTIONS II)()
1 Video
- COMPLEX NUMBERSBOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 3 ARGUMENT OF A COMPLEX NUMBER (OBJECTIVE QUESTIONS II)(ANALYTICAL & DESCRIPTIVE QUESTIONS )
1 Video
- COMPLEX NUMBERSBOOK - IIT JEE PREVIOUS YEARCHAPTER - COMPLEX NUMBERSEXERCISE - TOPIC 3 ARGUMENT OF A COMPLEX NUMBER (OBJECTIVE QUESTIONS II)(ONE OR MORE THAN ONE CORRECT OPTION)
2 Videos
- CIRCLEBOOK - IIT JEE PREVIOUS YEARCHAPTER - CIRCLEEXERCISE - Topic 5 Integer Answer type Question
1 Video
- DEFINITE INTEGRATIONBOOK - IIT JEE PREVIOUS YEARCHAPTER - DEFINITE INTEGRATIONEXERCISE - LIMITS AS THE SUM
6 Videos
Similar Questions
Knowledge Check
Match the items in Column I with the relevant item in column II.
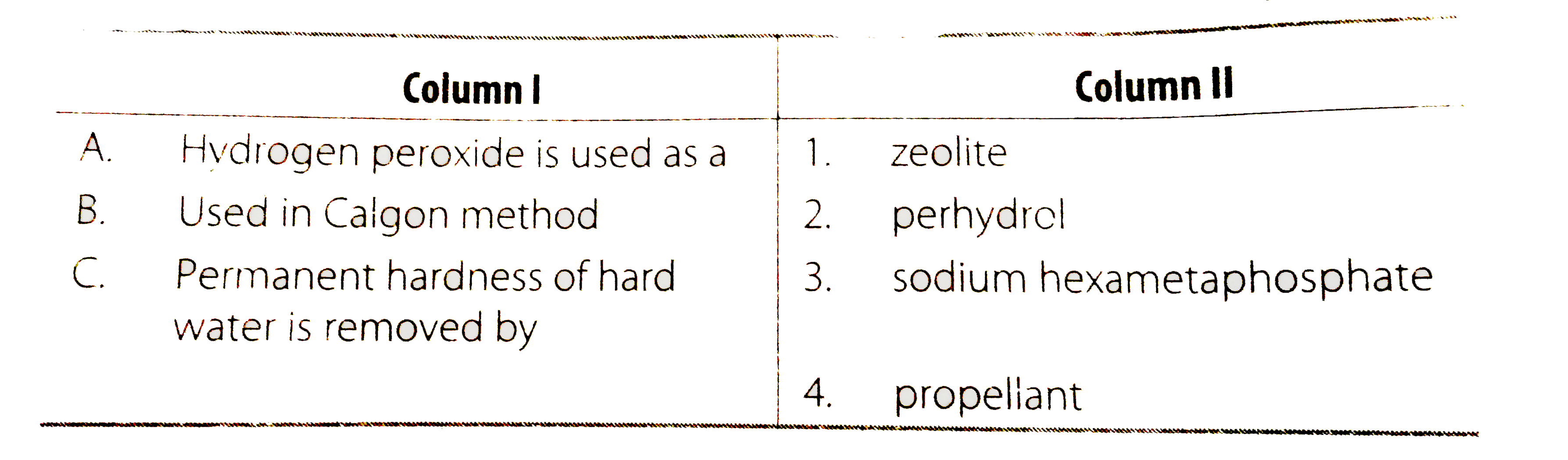 AP (p,r,s), Q(q, s), R(q,s), S(p, r, s)BP(p,r), Q(q), R(q, s), S(r,s)CP(p,s), Q(s), R(q), S(p, r, s)DP(p), Q(q ,s), R(s), S(p,s)
AP (p,r,s), Q(q, s), R(q,s), S(p, r, s)BP(p,r), Q(q), R(q, s), S(r,s)CP(p,s), Q(s), R(q), S(p, r, s)DP(p), Q(q ,s), R(s), S(p,s)
Match the terms given in Column I with the statements given in Column II

View SolutionMatch the reactions given in Column I with the statements given in Column II
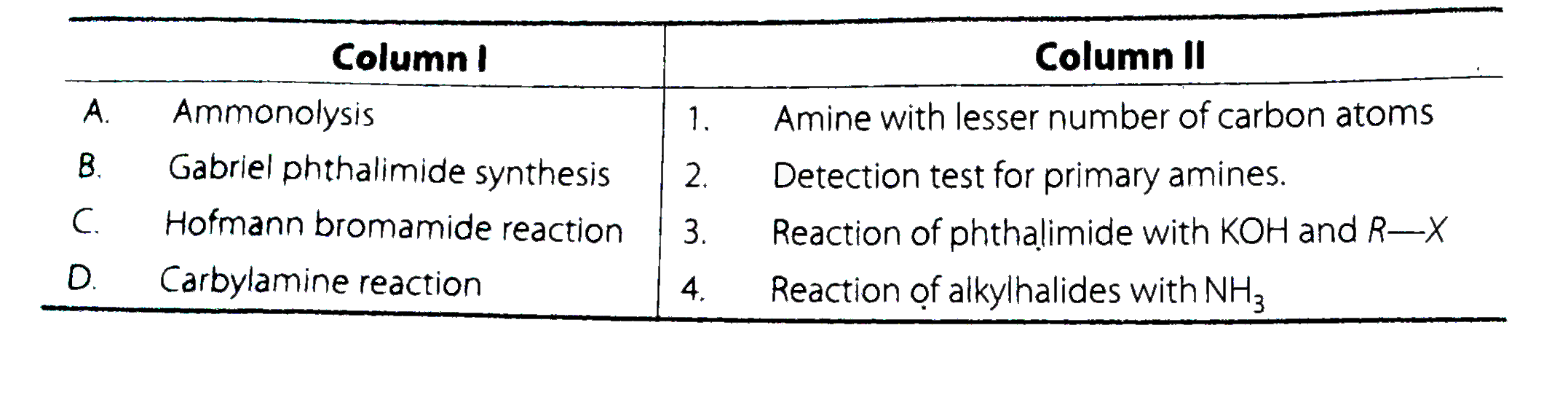
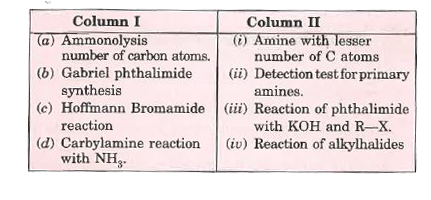
View SolutionMatch the reactions given in Column I with the statements given in Column II
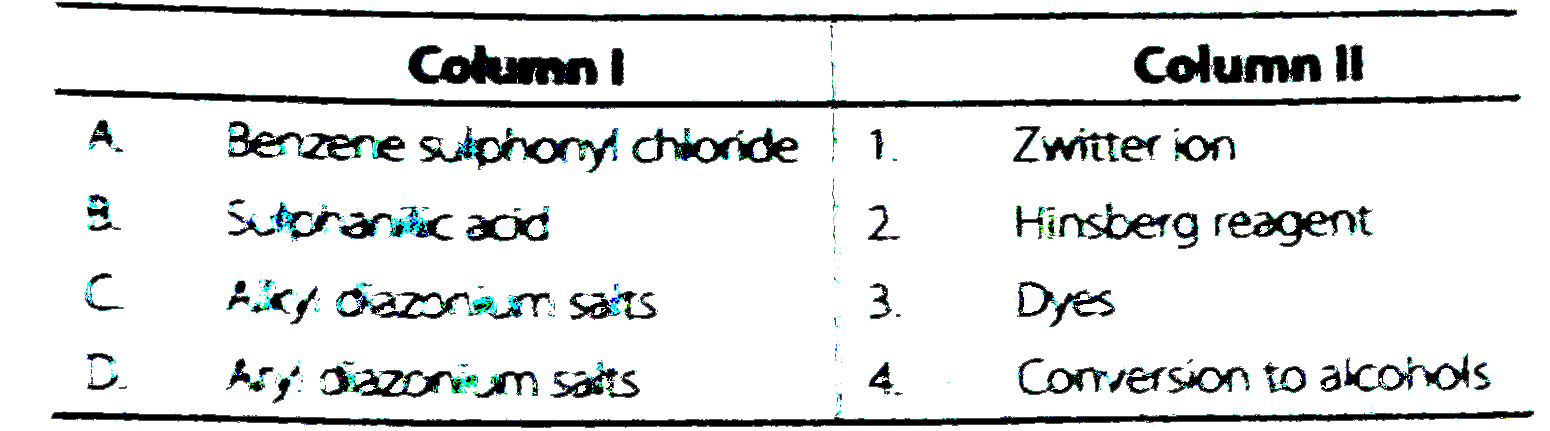
View SolutionMatch the reactions given in Column I with the statements given in Column II.
View SolutionMatch the conics in column I with statements/ex- pressions in Column II.

View SolutionMatch the conditions/expressions in Column I with statement in Column Ⅱ. (A). sin(π[x]) (B) sin{π(x−[x])}
View SolutionMatch the defects given in Column I with the statements in given Column II.

View Solution