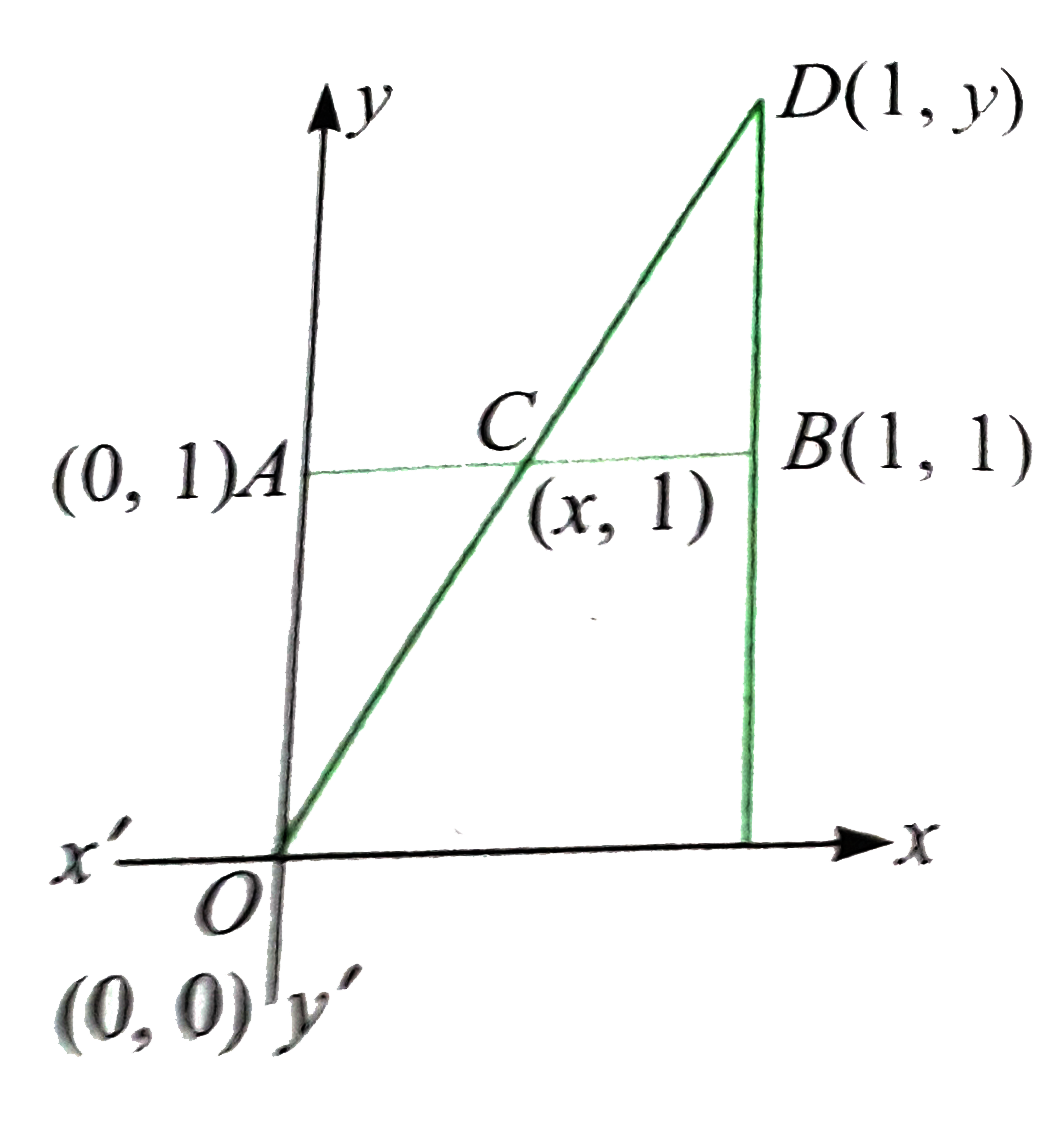
Consider the points O(0,0),A(0,1), and B(1,1) in the x-y plane. Suppose that points C(x,1)andD(1,y) are chosen such that 0<x<1. And such that O,C,andD are collinear. Let the sum of the area of triangles OAC and BCD be denoted by S. Then which of the following is/are correct?. Solution in Bengali
Minimum value of S is irrational lying in (1/3,1/2) .
Minimum value of S is irrational in (2/3,1).
The value of x for the minimum value of S lies in (2/3,1).
The value of x for the minimum values of S lies in (1/3,1/2).
The correct Answer is:A, C
S=Area ofΔOAC+Area ofΔBCD
=1.x2+(1−x)y−12,0<x<1
∴S=x2−(x−1)y−12 (1)
Now,Δ's CBD and OCA are similar. Therfore,
y−11=1−xx
∴S=x2−(x−1){(1/x)−1}2
=x2+(x−1)22x
=x2+(x−1)12x=(2x2−2x+1)2x
=x+12x−1=(√x−1√2x)2−1+√2
Therefore, A is minimum if √x=1/√2x. therefore , x=1/√2
which lies in (2/3,1) and Amin=√2 which lies in (1/3,1/2).
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Linked
10 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Matrix match type
4 Videos
- COORDINATE SYSYEM BOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSYEM EXERCISE - Exercises
59 Videos
- COORDINATE SYSTEMBOOK - CENGAGE PUBLICATIONCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE PUBLICATIONCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
For points P=(x1,y1)and Q=(x2,y2) of the co-ordinate plane a new distance d(P,Q)=|x1−x2|+|y1−y2| is defined .Let O(0,0)and A(3,2). The set of points in first quadrant which are equidistant from O and A is
View SolutionConsider the lines represented by equation (x2+xy−x)×(x−y)=0 forming a triangle. Then match the following lists:

View SolutionABC is an isosceles triangle. If the coordinates of the base are B(1,3) and C(−2,7) , the coordinates of vertex A
View SolutionCoordinates of points on curve 5x2−6xy+5y2−4=0 which are nearest to origin are
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionConsider the points O(0,0),A(0,1) , and B(1,1) in the x,y plane. Suppose that points C(x,1) and D(1,y) are chosen such that 0<x<1 and such that O,C and D are collinear. Let the sum of the area of triangles OAC and BCD be denoted by S . Then which of the following is/are correct? Minimum value of S is irrational lying in (13,12). Minimum value of S is irrational in (23,1) The value of x for the minimum value of S lies in (23,1). The value of x for the minimum value of S lies in (13,12).
View SolutionA point O inside a rectangle ABCD is joined to the vertices. Prove that the sum of the areas of a pair of opposite triangles so formed is equal to the sum of the other pair of triangles. Given: A rectangle ABCDandO is a point inside it. OA,OB,OCandOD have been joined. To Prove: ar(AOD)+ar(BOC)=ar(AOB)+ar(COD)
View SolutionA point equidistant from the line 4x+3y+2=0, 5x−12y+26=0 and 7x+24y−50=0 is (1,−1) b. (1,1) c. (0,0) d. (0,1)
View SolutionConsider the points O(0,0),A(0,1), and B(1,1) in the x-y plane. Suppose that points C(x,1)andD(1,y) are chosen such that 0<x<1. And such that O,C,andD are collinear. Let the sum of the area of triangles OAC and BCD be denoted by S. Then which of the following is/are correct?.
View SolutionIf A, B, C, D are four non-collinear points in the plane such that ¯¯¯¯¯¯AD+¯¯¯¯¯¯BD+¯¯¯¯¯¯CD=¯¯¯O, then the point D is the … of triangle ABC.
View SolutionThe equation of the tangent to the curve y=secx at the point (0,1) is
(a) y=0
(b) x=0
(c) y=1
(d) x=1
View SolutionThe curve given by x+y=exy has a tangent parallel to the y-axis at the point
View SolutionConsider the points O(0,0),A(0,1), and B(1,1) in the x-y plane. Suppose that points C(x,1)andD(1,y) are chosen such that 0 < x < 1. And such that O,C,andD are collinear. Let the sum of the area of triangles OAC and BCD be denoted by S. Then which of the following is/are correct?.
View SolutionConsider the points O(0,0),A(0,1), and B(1,1) in the x-y plane. Suppose that points C(x,1)andD(1,y) are chosen such that 0<x<1. And such that O,C,andD are collinear. Let the sum of the area of triangles OAC and BCD be denoted by S. Then which of the following is/are correct?. (a) Minimum value of S is irrational lying in ( 1 / 3 , 1 / 2 ) (b) Minimum value of S is irrational in ( 2 / 3 , 1 ) . (c) The value of x for the minimum value of S lies in ( 2 / 3 , 1 ) . (d) The value of x for the minimum values of S lies in ( 1 / 3 , 1 / 2 ) .
View SolutionThe curve given by x+y=exy has a tangent parallel to the y−aξs at the point (0,1) (b) (1,0) (c)(1,1) (d) none of these
View SolutionA point O inside a rectangle ABCD is joined to the vertices. Prove that the sum of the areas of a pair of opposite triangles so formed is equal to the sum of the other pair of triangles. Given: A rectangle ABCD and O is a point inside it. OA, OB, OC and OD have been joined. To Prove: ar (AOD)+ ar (BOC)= ar (AOB)+ ar(COD)
View Solution
