Consider two lines L1:2x+y=4 and L2:(2x−y=2).Find the angle between L1 and L2.Solution in Malayalam
Step by step video & image solution for Consider two lines L_1:2x+y=4 and L_2:(2x-y=2).Find the angle between L1 and L2. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Find the sum to n terms of the sequence
4+44+444+…….
View SolutionIf A={x<x is a natural number,x<5 and
x>7},then n(A) is
View SolutionThe combined equation of the lines L1and L2 is 2x2+6xy+y2=0, and that of the lines L3 and L4 is 4x2+18xy+y2=0. If the angle between L1 and L4 be α , then the angle between L1andL3 will be .
View SolutionThe shortest distance between the two lines L1:x=k1,y=k2andL2:x=k3,y=k4 is equal to
View SolutionLet L1 be a tangent to the parabola y2=4(x+1)andL2 be a tangent to the parabola y2=8(x+2) such that L1andL2 intersect at right angles. Then L1andL2 meet on the straight line :
View SolutionL1=2x+2y−8=0 మరియు L2=x+y−4=0 ల మరియు L1=KL2 గా రాస్తే k విలువ ..........
View SolutionThe equations of bisectors of two lines L1&L2 are 2x−16y−5=0 and 64x+8y+35=0. lf the line L1 passes through (−11,4), the equation of acute angle bisector of L1 & L2 is:
View SolutionConsider the line
L1=x+13=y+21=z+12
L2=x−21=y+22=z−33
The shortest distance between L1 and L2 is
View SolutionL1andL2 are two intersecting lines. If the image of L1 w.r.t. L2 and that of L2 w.r.t. L1 concide, then the angle between L1andL2 is
View SolutionConsider two points A and B on L1 and a line L2 as shown in figure. Find the foot of the perpendicular drawn from (2,3,4) on L1 to the line L2.
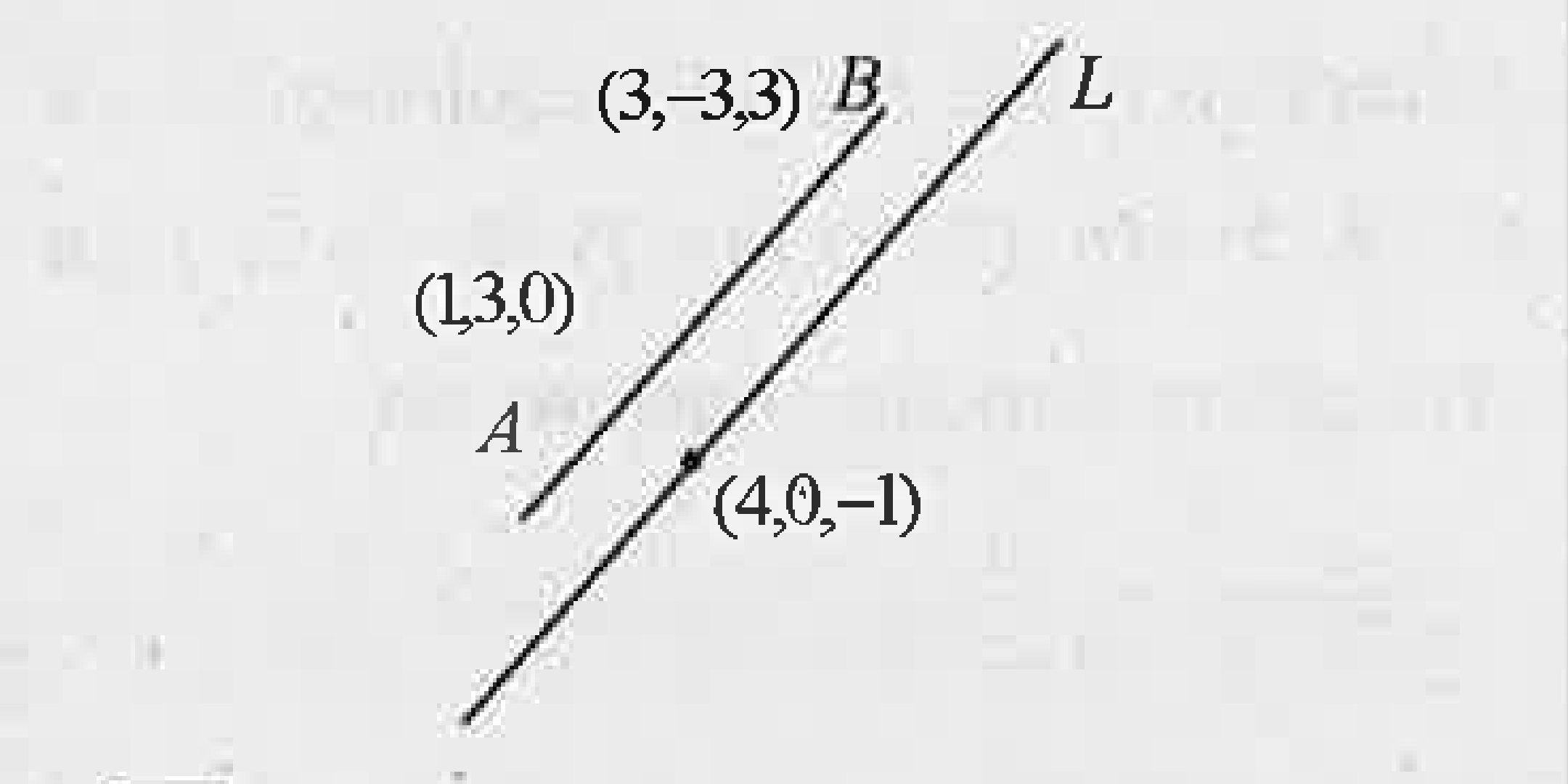
View SolutionConsider two lines L1:2x+y=4 and
L2:(2x−y=2)
Find the equation of the line passing through the intersection of L1 and L2 which makes an angle 45° with the positive direction of x−aξs.
View SolutionConsider two lines L1:2x+y=4 and
L2:(2x−y=2)
Find the equation of the line passing through the intersection of L1 and L2 which makes an angle 45° with the positive direction of x−aξs.
Find the x and y intercepts of the third line obtained.
View SolutionConsider the lines
l1:x−12=y−1−1=z1
l2:x−23=y−1−5=z+11
Find The shortest distance between the lines l1 and l2
View SolutionConsider the lines: L1:x−21=y−17=z+2−5,L2:x−4=y+3=−z Then which of the following is/are correct ? (A) Point of intersection of L1andL2is(1,−6,3)
View SolutionGiven equation of line L1 is y=4
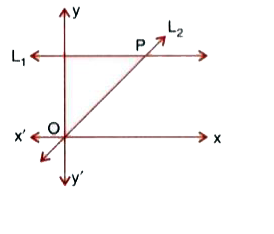
Write the slope of line L1 if L2 is the bisector of angle O.
View Solution
