D,E,F are three points on the sides BC,CA,AB, respectively, such that ∠ADB=∠BEC=∠CFA=θ. A′,B'C' are the points of intersections of the lines AD,BE,CF inside the triangle. Show that are of A′B′C′=4cos2θ, where is the area of ABC.Solution in Bengali
From ΔAB'CAB'sin(π−(A+θ))=ACsin(π−B)
![ocr_image]()
⇒AB'=2Rsin(A+θ)
From ΔAC'B,AC'sin(θ−A)=ABsin(π−C)
⇒AC'=2Rsin(θ−A)
∴B'C'=2R(sin(A+θ)−sin(θ−A))
=4RcosθsinA=2acosθ
Similarly, C'A'=2bcosθ
∴ Area of ΔA'B'C'=12(B'C')(A'C')sin∠B'C'A'
=12(2acosθ)(2bcosθ)sinC
=4cos2θ×Δ
Topper's Solved these Questions
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE PUBLICATIONCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Illustration
86 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE PUBLICATIONCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Concept application exercise 5.1
12 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE PUBLICATIONCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE )
8 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - All Questions
1119 Videos
Similar Questions
If an a triangle ABC,a=3band B−A=900, then find the value of tanA
View SolutionFind the value of a2+b2+c2R2 in any right-angled triangle.
View SolutionLet Sk,k=1,2,….100 denote the sum of the infinite geometric series whose first term is k−1K! and the common
ration is 1k then the value of (100)2100!+Σ100k=1|(k^2-3k+1)S_k| is ____________
View SolutionIf the function f:[1,∞)→[1,∞) is defined by f(x)=2x(x−1), then find f−1(x)
View SolutionIf f(x)<0, x∈(a,b) then at the point C(c, f(c)) on y=f(x) for whichF(c) is a maximum, f′(c) is given by a.f′(c)=f(b)−f(a)b−1 b. f′(c)=f(b)−f(a)a−b c.f′(c)=2(f(b)−f(a))b−a d. f′(c)=0
View SolutionD, E and F are the points on sides BC, CA and AB respectively of ABC such that AD bisects ∠A, BE bisects ∠B and CF bisects ∠C . If AB=5cm, BC=8cm and CA=4cm , determine AF, CE and BD .
View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
View SolutionD, E, F are the mid-point of the sides BC, CA and AB respectively of ABC. Then DEF is congruent to triangle. ABC (b) AEF (c) BFD, CDE (d) AFE, BFD, CDE
Given : D, E, F are the mid-point of the sides BC, CA and AB .
View SolutionIn Figure, AB⊥BEandFE⊥BE.If BC=DEandAB=EF, then ABD is congruent to:
(a)EFC
(b) ECF
(c) CEF
(d) FEC
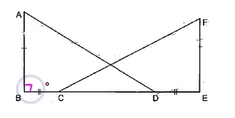
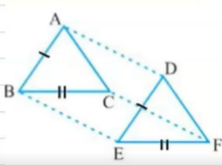
View SolutionIf ABC and DEF are two triangles such that AB, BC are respectively equal and parallel to DE, EF, then show that Quadrilateral ABED is a parallelogram Quadrilateral BCFE is a parallelogram AC=DF (iv) ABC ≅ DEF
View SolutionIf D,EandF are three points on the sides BC,CAandAB, respectively, of a triangle ABC show that the BDCD=CEAE=AFBF=−1
View SolutionThe sides BC,CA and AB of a triangle ABC, are produced in order, forming exterior angles ∠ACD,∠BAE and ∠CBF . Show that ∠ACD+∠BAE+∠CBF=3600
View SolutionThe diagonal BD of a parallelogram ABCD intersects the segment AE at the point F, where E is any point on the side BC . Prove that DF⋅EF=FB⋅FA.
View SolutionIn a ABC,D and E are points on sides ABandAC respectively such that BD=CE. If ∠B=∠C, show that DE∣∣BC.
View SolutionO is any point inside a triangle ABC . The bisector of ∠AOB,∠BOC and ∠COA meet the sides AB,BC and CA in point D,EandF respectively. Show that AD⋅BE⋅CF=DB⋅EC⋅FA
View SolutionD, E and F are the points on sides BC, CA and AB respectively of ABC such that AD bisects ∠A, BE bisects ∠B and CF bisects ∠C . If AB=5cm, BC=8cm and CA=4cm , determine AF, CE and BD .
View Solution
