Determine the ratio in which the line $3 x + y - 9 = 0$ divides the segment joining the points (1,3) and $(2, 7) .$

A

3:4 externally

B

3:4 iternally

C

4:5 internally

D

5:6 externally

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

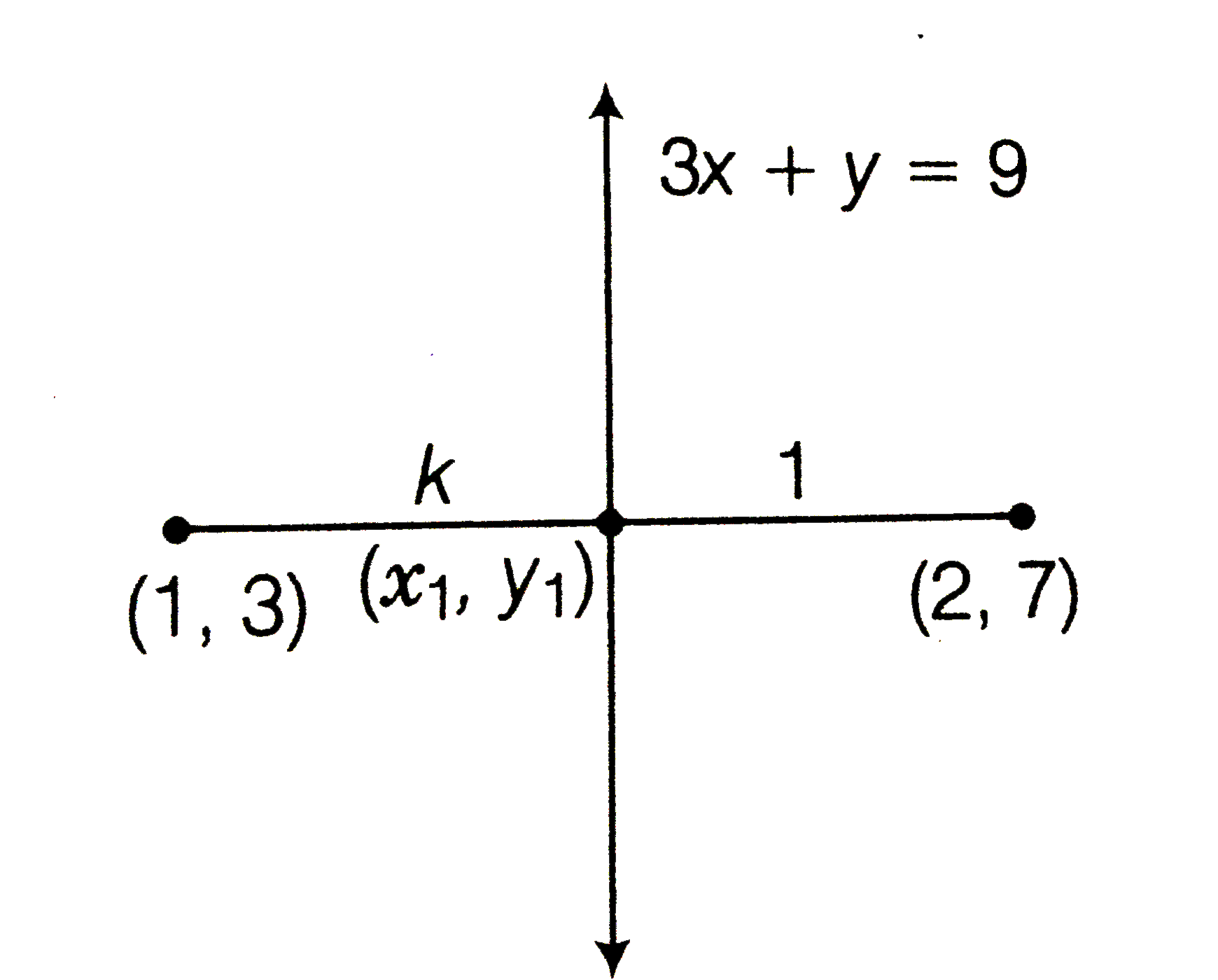
Clearly , $x_{1} = \frac{2 k + 1}{k + 1} and y_{1} = \frac{7 k + 3}{k + 1}$
Since ( $x_{1}, y_{1})$ satisfies the equation 3x +y =9
$3 . (\frac{2 k + 1}{k + 1}) + (\frac{7 k + 3}{k + 1}) = 9$
$\Rightarrow 6 k + 3 + 7 k + 3 = 9 k + 9$
$\Rightarrow 13 k - 9 k = 9 - 6$
$\Rightarrow 4 k - 3 \Rightarrow k = \frac{3}{4}$
Thus, line 3x+y=9 divides the line segement in the ratio $\frac{3}{4} : 1$ i.e, 3 : 4 internally.

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

The ratio in which the line $3 x + y = 9$ divides the line sequent joining the points (1, 3) and (2, 7) is given by
A $4 : 3$
B $3 : 4$
C $2 : 3$
D $3 : 2$

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-STRAIGHT LINE -EXERCISE 2(MISCELLANEOUS PROBLEMS)

Home
Ask Doubt
Profile