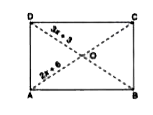
Diagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA= 3 cm and OD = 2 cm, determine the lengths of AC and BD.
To solve the problem, we need to determine the lengths of the diagonals AC and BD of the parallelogram ABCD, given that the diagonals intersect at point O. We know that OA = 3 cm and OD = 2 cm.
1. Understanding the Properties of Parallelograms:
In a parallelogram, the diagonals bisect each other. This means that the point of intersection (O) divides each diagonal into two equal parts. Therefore, we have:
- AO = OC
- BO = OD
2. Finding OC:
Since OA = 3 cm, and because O is the midpoint of diagonal AC, we have:
OC=OA=3 cm
3. Calculating the Length of Diagonal AC:
The total length of diagonal AC can be calculated as:
AC=AO+OC=OA+OC=3 cm+3 cm=6 cm
4. Finding OB:
Given that OD = 2 cm, and because O is the midpoint of diagonal BD, we have:
OB=OD=2 cm
5. Calculating the Length of Diagonal BD:
The total length of diagonal BD can be calculated as:
BD=OB+OD=OB+OD=2 cm+2 cm=4 cm
Final Results:
- The length of diagonal AC is 6 cm.
- The length of diagonal BD is 4 cm.
In a parallelogram, the diagonals bisect each other. This means that the point of intersection (O) divides each diagonal into two equal parts. Therefore, we have:
- AO = OC
- BO = OD
Since OA = 3 cm, and because O is the midpoint of diagonal AC, we have:
OC=OA=3 cm
The total length of diagonal AC can be calculated as:
AC=AO+OC=OA+OC=3 cm+3 cm=6 cm
Given that OD = 2 cm, and because O is the midpoint of diagonal BD, we have:
OB=OD=2 cm
The total length of diagonal BD can be calculated as:
BD=OB+OD=OB+OD=2 cm+2 cm=4 cm
- The length of diagonal AC is 6 cm.
- The length of diagonal BD is 4 cm.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - QUADRILATERALS EXERCISE - SHORT ANSWER TYPE QUESTIONS10videos
QUADRILATERALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - QUADRILATERALS EXERCISE - LONG ANSWER TYPE QUESTIONS18videos
QUADRILATERALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - QUADRILATERALS EXERCISE - LONG ANSWER TYPE QUESTIONS18videos
QUADRILATERALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - POLYNOMIALSEXERCISE - EXERCISE 2.4 Long Answer type Questions9videos
POLYNOMIALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS12videos
STATISTICS AND PROBABILITY
Similar Questions
In the given figure, the diagonals AC and BD of a rectangle ABCD intersect each other at point O.
If OA=2x+6 and OD=3x+3, find the value of x.View SolutionIn rhombus ABCD, diagonals AC and BD intersect each other at point O.
If cosine of angle CAB is 0.6 and OB = 8 cm, find the lengths of the side and the diagonals of the rhombus.View Solution
Knowledge Check
- Question 1 - Select One

The given figure shows a parallelogram ABCD whose diagonals intersect each other at point O. if OB=6 cm and AC is 6 cm more than BD, find OC.A12cmB10cmC9cmD11cm
Diagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that ar (APB) × ar (CPD) = ar (APD) × ar (BPC).
View SolutionDiagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using a similarity criterion for two triangles, show that OAOC=OBOD
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way thatar(AOD)=ar(BOC).Prove that ABCD is a trapezium.
View SolutionIn the figure, given below, straight lines AB and CD intersect at P, and AC//BD.
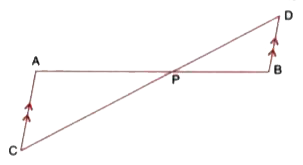
If BD = 2.4 cm, AC = 3.6 cm, PD = 4.0 cm and PB = 3.2 cm, find the lengths of PA and PC.View SolutionFind the area of a parallelogram ABCD in which AB = 8 cm, BC = 15 cm and diagonal AC = 17 cm.
View SolutionIn quadrilateral ABCD, the diagonals AC and BD intersect each other at point O.
If AO = 2CO and BO = 2DO, show that:
ΔAOB is similar to ΔCOD.View SolutionIf ABCD is a parallelogram, then −−→AC−−−→BD=
View Solution
