Draw a quadrilateral in the Cartesian plane, whose vertices are (−4,5), (0,7), (5,−5)and (−4,−2). Also, find its area.
In the figure the vertices A = (-4,5), B = (0,7), C = (5,-5) and D = (-4,-2) of the quadrilateral are plotted on the graph.
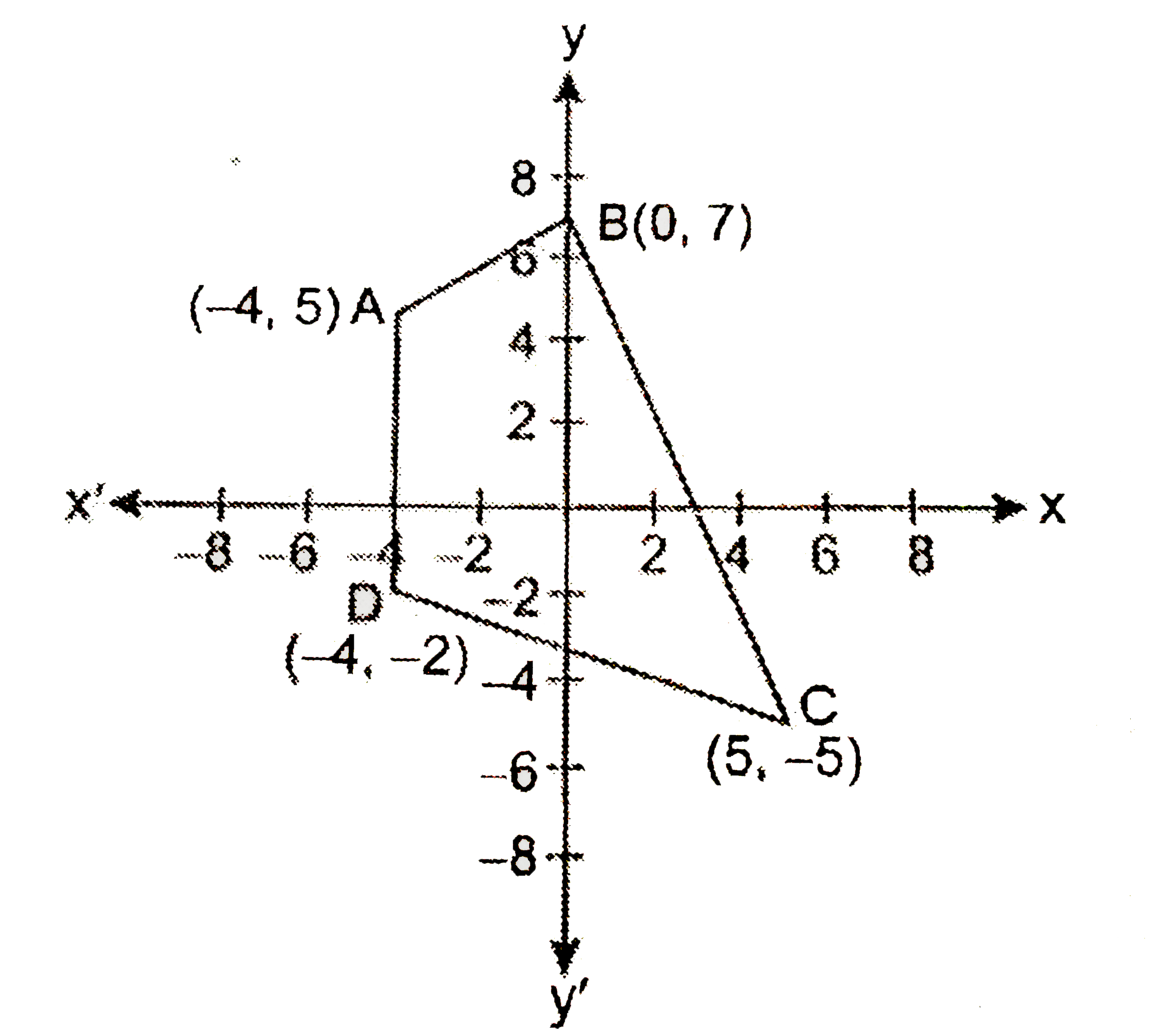
From the given vertices,
x1=−4,y1=5
x2=0,y2=7
x3=5,y3=−5
x4=−4,y4=−2
Join the diagonal AC.
Area of ΔABC,
=12[(x1y2+x2y3+x3y1)−(y1x2+y2x3+y3x1)]
=12[{−4×7+0×(−5)+5×5}−{5×0+7×5+(−5)×(−4)}]
=12[(−28+0+25)−(0+35+20)]
=12[(−3)−(55)]
=12(−58)=29 (neglecting negative sign)
Area of ΔACD
=12[(x1y3+x3y4+x4y1)−(y1x3+y3x4+y4x1)]
=12[{−4×−5+5×(−2)+5×(−4)}−{5×5+(−5)×(−4)+(−2)×(−4)}]
=12[(20−10−20)−(25+20+8)]
=12[(−10)−(53)]
=12[−63]=632 (neglecting negative sign)
=31.5
Therefore, are of quadrilateral ABCD
= Area of ΔABC + Area of ΔACD
=29+31.5=60.5 units
Therefore, are of quadrilateral ABCD = 60.5 units.
Topper's Solved these Questions
- STRAIGHT LINESBook:NAGEEN PRAKASHAN ENGLISHChapter:STRAIGHT LINESExercise:Exercise
Explore 207 Videos
- STATISTICSBook:NAGEEN PRAKASHAN ENGLISHChapter:STATISTICSExercise:MISCELLANEOUS EXERCISE
Explore 7 Videos
- TRIGNOMETRIC FUNCTIONSBook:NAGEEN PRAKASHAN ENGLISHChapter:TRIGNOMETRIC FUNCTIONSExercise:MISCELLANEOUS EXERCISE
Explore 10 Videos
Similar Questions
Find the area of the quadrilateral whose vertices are : (−4,5),(0,7),(5,−5)and(−4,−2)
View SolutionFind the area of the pentagon whose vertices are (4,3),(−5,6),(0,−7),(3,−6),(−7,−2)
View SolutionFind the area of the triangle whose vertices are : (3,-4), (7, 5), (-1, 10)
View SolutionFind the area of the triangle whose vertices are: (−2,4),(2,−6),(5,4)
View SolutionFind the area of the triangle whose vertices are (3,8), (-4,2) and (5, -1).
View SolutionFind the area of the triangle whose vertices are: (−3,2),(5,4),(7,−6)
View SolutionFind the area of the triangle whose vertices are (3,8),(−4,2)and (5,1).
View Solutionfind the area of triangle whose vertices are (3,8),(-4,2)and (5,1)
View SolutionFind the area of the triangle whose vertices are: (1,4),(2,3),(−5,−3)
View SolutionFind the area of triangle, whose vertices are (2,3), (7,5) and (-7,-5).
View Solution