The base f an equilateral triangle with side 2a lies along the y-axis such that the mid point of the base is at the origin. Find the vertices of the triangle.

Let ABC is an equilateral triangle, each of whose sids AB, B and CA is 2a units.
∵ The base AB of triangle is along y-axis.
∴ The x-coordinates of points A and B will be zero
And origin is the mid-point of AB and AB=2aunits.
∴OA=a and OB=a
Now, coordinates of point A=(0,a)
Coordinates of point B=(0,−a)
Now, let coordinates of point C be (x,0)
Then AC=√(x−0)2+(0−a)2
⇒AC2=x2+a2.......(1)
But AC=2a⇒AC2=4a2........(2)
Then from equation (1) and (2),
x2+a2=4a2
∴x2=4a2−a2=3a2
∴x=√3a2⇒x=±a√3
The coordinates of point C=(±a√3,0)
Therefore, the vertices of triangle are A(0,a), B(0,−a), C(asqrt(3),0)orA(0,a),B(0,-a),C(-asqrt(3),0)
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Exercise207videos
STRAIGHT LINES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - MISCELLANEOUS EXERCISE7videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIGNOMETRIC FUNCTIONSEXERCISE - MISCELLANEOUS EXERCISE10videos
TRIGNOMETRIC FUNCTIONS
Similar Questions
The base of an equilateral triangle with side 2a lies along the y-axis such that the mid point of the base is at the origin. Find the vertices of the triangle.
View SolutionThe base of an equilateral triangle with side 2a lies along the y–axis such that the mid–point of the base is at the origin. Find vertices of the triangle.
View Solution
Knowledge Check
- Question 1 - Select One
What is the area of equilateral triangle if the base BC = 6 ?
 A9√3B18√3C26√3D30√3
A9√3B18√3C26√3D30√3
The base of an equilateral triangle with side 20cm lies along x-axis such that the mid-point of the base is at the origin. Find the vertices of the triangle
View SolutionTwo uniform spheres A (Hollow) and B (solid) of same radius R (√125GmPr, where P is _____
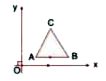
View SolutionThe base AB of two equilateral triangles ABC and ABC' with side 2a lies along the X -axis such that the mid-point of AB is at the origin as shown in . Find the coordinates of the vertices C and C' of the triangles.
View SolutionLet the point P lies in the interior of an equilateral triangle with side lengths 2 units, each. Find the sum of the shortest distances from P to the sides of the triangle.
View SolutionEquation of the base of an equilateral triangle is 3x+4y=9 and its vertex is at point (1,2) .Find the equations of the other sides and the length of each side of the triangle .
View SolutionIf (-4,3) and (4,3) are two vertices of an equilateral triangle, then find the coordinates of the third vertex, given that the origin lies in the interior of the triangle.
View SolutionThe side AB of an equilateral triangle ABC is parallel to the x-axis. Find the slopes of all its sides.
 View Solution
View Solution

