Draw the graph of y=tan2x.
Video Solution
Text Solution
We have y=f(x)=tan2x
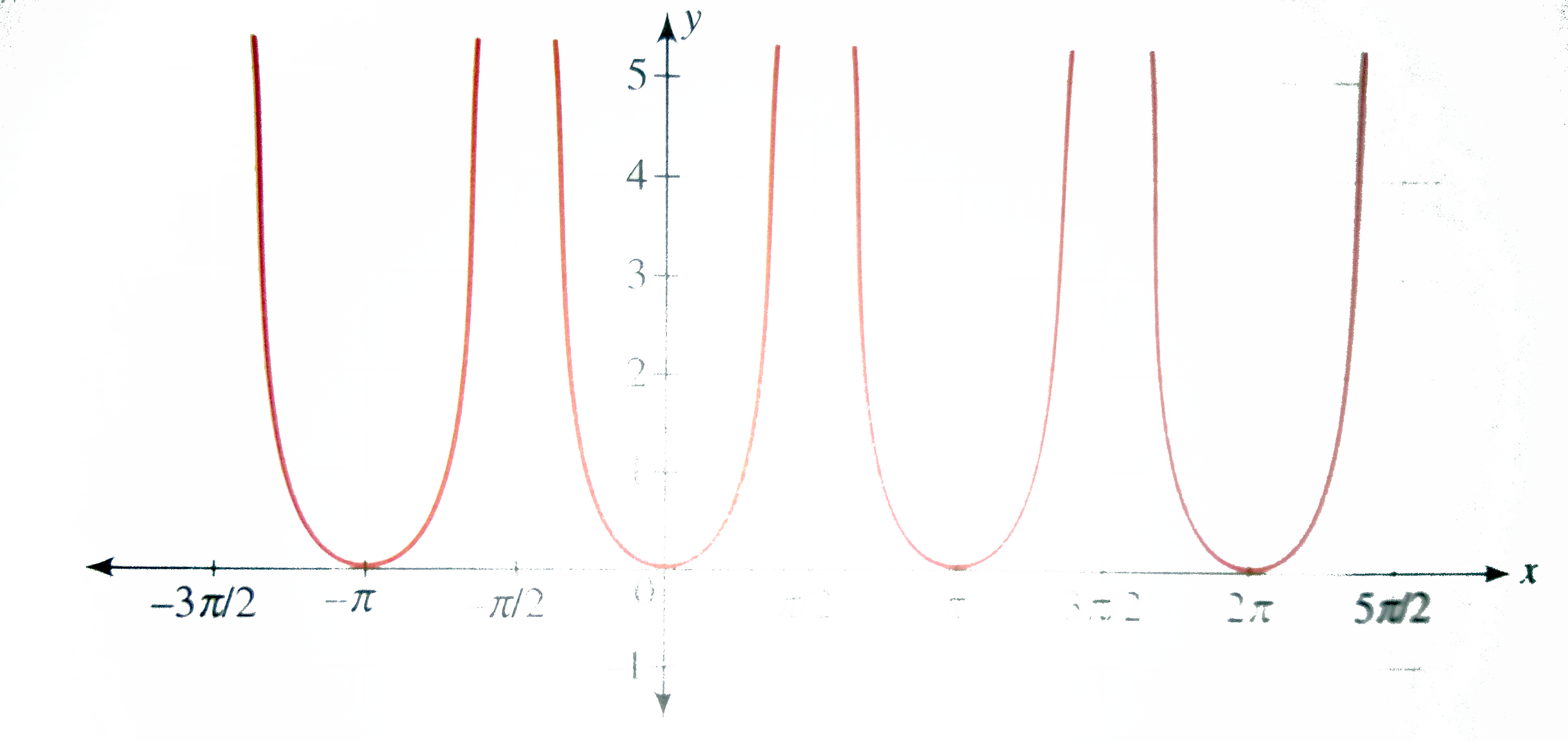
Period of the function is π.
Function is even, so the graph is symmetrical above the x - axis.
Let us draw the graph for 0<x<π/2.
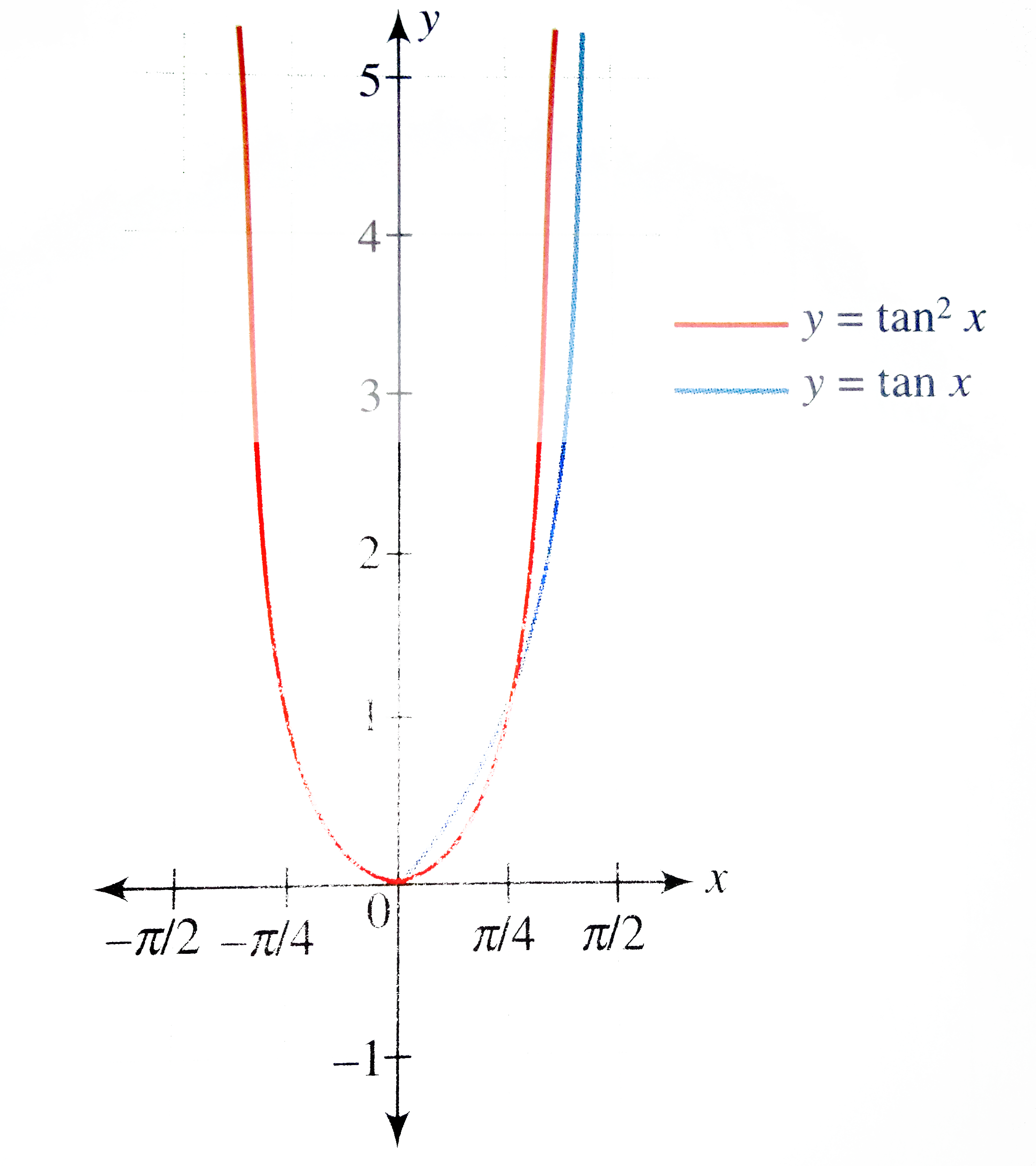
For 0<x<π/4,0<tanx<1
∴ tan2x<tanx
For x>π/4
tanx>1
∴ tan2x>tanx
Hence the graph of y=tan2x lies below and above the graph of y = tan x for x∈(0,π/4) and x∈(π/4,π/2).
So the graph of the function for x∈(−π/2,π/2) is as shown in the following figure.
Since the function has period π , the graph of the function is as shown in the following figure.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives3videos
HIGHT AND DISTANCE
Similar Questions
Draw the graph of y=2x.
View SolutionDraw the graph of y=|x|
View SolutionDraw the graph of y=sin2x.
View SolutionDraw th graph of y=−2x
View SolutionDraw the graph of y=cos2x.
View SolutionDraw the graph of y=tan−1(tanx)
View SolutionDraw the graph of y=tan(3x).
View SolutionDraw the graph of y=2x−1
View SolutionDraw the graph of y=tan−1|x|.
View SolutionDraw the graph of y=sinxcos2x
View Solution

