Draw two concentric circles of radii 3 cm and 5 cm. Taking a point on outer circle, construct the pair of tangents to the other. Measure the length of a tangent and verify is by actual calculation.
To solve the problem of drawing two concentric circles of radii 3 cm and 5 cm, and constructing the pair of tangents from a point on the outer circle to the inner circle, follow these steps:
1. Draw the Concentric Circles:
- Start by drawing a point O, which will be the center of both circles.
- Using a compass, draw a circle with a radius of 3 cm (this is Circle C1).
- Without changing the compass width, draw another circle with a radius of 5 cm (this is Circle C2). Both circles should share the same center O.
2. Choose a Point on the Outer Circle:
- Select a point P on the circumference of the outer circle (Circle C2). This point will be used to construct the tangents.
3. Construct a Circle with Diameter OP:
- With point P as one endpoint, draw a line segment from O to P.
- Find the midpoint M of the line segment OP. You can do this by measuring the length of OP and marking the halfway point.
- Using M as the center, draw a circle (Circle C3) with a radius equal to the length of OM (which is equal to OP/2).
4. Identify Points of Intersection:
- Circle C3 will intersect Circle C1 at two points. Label these points as Q1 and Q2.
5. Draw Tangents from Point P:
- Draw lines from point P to points Q1 and Q2. These lines are the required tangents to Circle C1 from point P.
6. Measure the Length of the Tangents:
- Use a ruler to measure the length of one of the tangents (let's say PQ1). Record this measurement.
7. Verify the Length of the Tangents:
- To verify the length of the tangent mathematically, use the Pythagorean theorem. In triangle OPQ1:
- OP is the radius of the outer circle (5 cm).
- OQ1 is the radius of the inner circle (3 cm).
- The tangent length PQ1 can be calculated using the formula:
PQ1=√OP2−OQ12
- Substitute the values:
PQ1=√52−32=√25−9=√16=4 cm
- Start by drawing a point O, which will be the center of both circles.
- Using a compass, draw a circle with a radius of 3 cm (this is Circle C1).
- Without changing the compass width, draw another circle with a radius of 5 cm (this is Circle C2). Both circles should share the same center O.
- Select a point P on the circumference of the outer circle (Circle C2). This point will be used to construct the tangents.
- With point P as one endpoint, draw a line segment from O to P.
- Find the midpoint M of the line segment OP. You can do this by measuring the length of OP and marking the halfway point.
- Using M as the center, draw a circle (Circle C3) with a radius equal to the length of OM (which is equal to OP/2).
- Circle C3 will intersect Circle C1 at two points. Label these points as Q1 and Q2.
- Draw lines from point P to points Q1 and Q2. These lines are the required tangents to Circle C1 from point P.
- Use a ruler to measure the length of one of the tangents (let's say PQ1). Record this measurement.
- To verify the length of the tangent mathematically, use the Pythagorean theorem. In triangle OPQ1:
- OP is the radius of the outer circle (5 cm).
- OQ1 is the radius of the inner circle (3 cm).
- The tangent length PQ1 can be calculated using the formula:
PQ1=√OP2−OQ12
- Substitute the values:
PQ1=√52−32=√25−9=√16=4 cm
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 11 A13videos
CONSTRUCTIONS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 11 B10videos
CONSTRUCTIONS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Construction of Tangents to a Circle5videos
CONSTRUCTIONS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions10videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions5videos
INTRODUCTION TO TRIGONOMETRY
Similar Questions
Draw two concentric circles of radii 3 cm and 5 cm . Taking a point on outer circle construct the pair of tangents to the other. Measure the length of a tangent and verify it by actual calculation .
View SolutionDraw two concentric circles with radii 4 cm and 6 cm. Taking a point on the outer circle, construct a pair of tangents to inner circle. By measuring the lengths of both the tangents, show that they are equal to each other.
View SolutionDraw a circle of radius 6cm. From a point 10cm away from its centre, construct the pair of tangents to the circle and measure their lengths.
View SolutionDraw a circle of radius 4 cm. From the point 7 cm away from its centre, construct the pair of tangents to the circle.
View SolutionDraw a circle of radius 5.0 cm. Take a point P on it. Without using the centre of the circle construct a tangent at the point P.
View SolutionIf radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
View SolutionDraw a circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this points to the circle.
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
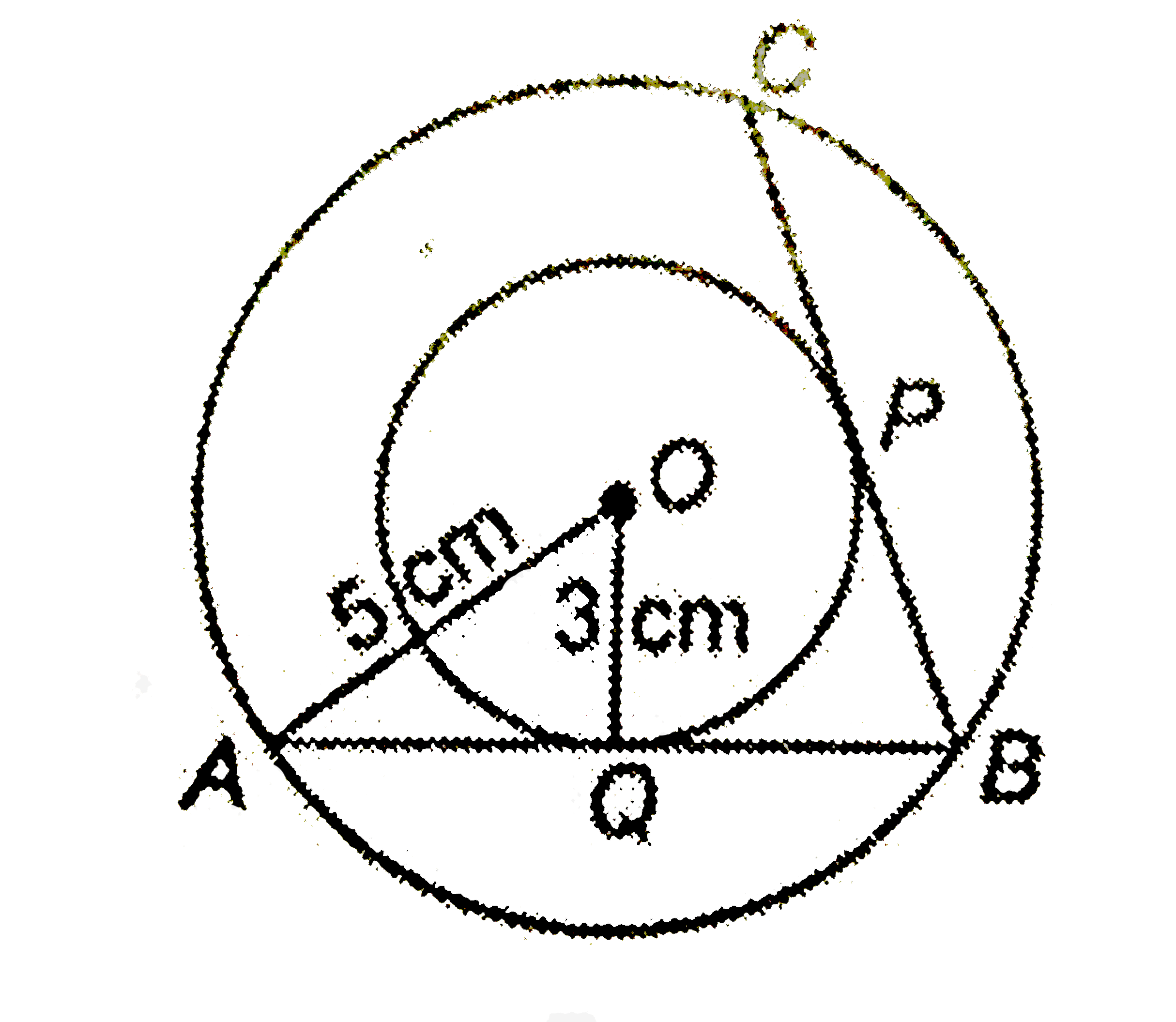 View Solution
View SolutionDraw a circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this point to the circle.
View SolutionTwo concentric circles are of radii 13 cm and 5 cm. Find the length of the chord of the larger circle which touches the inner circle.
View Solution
