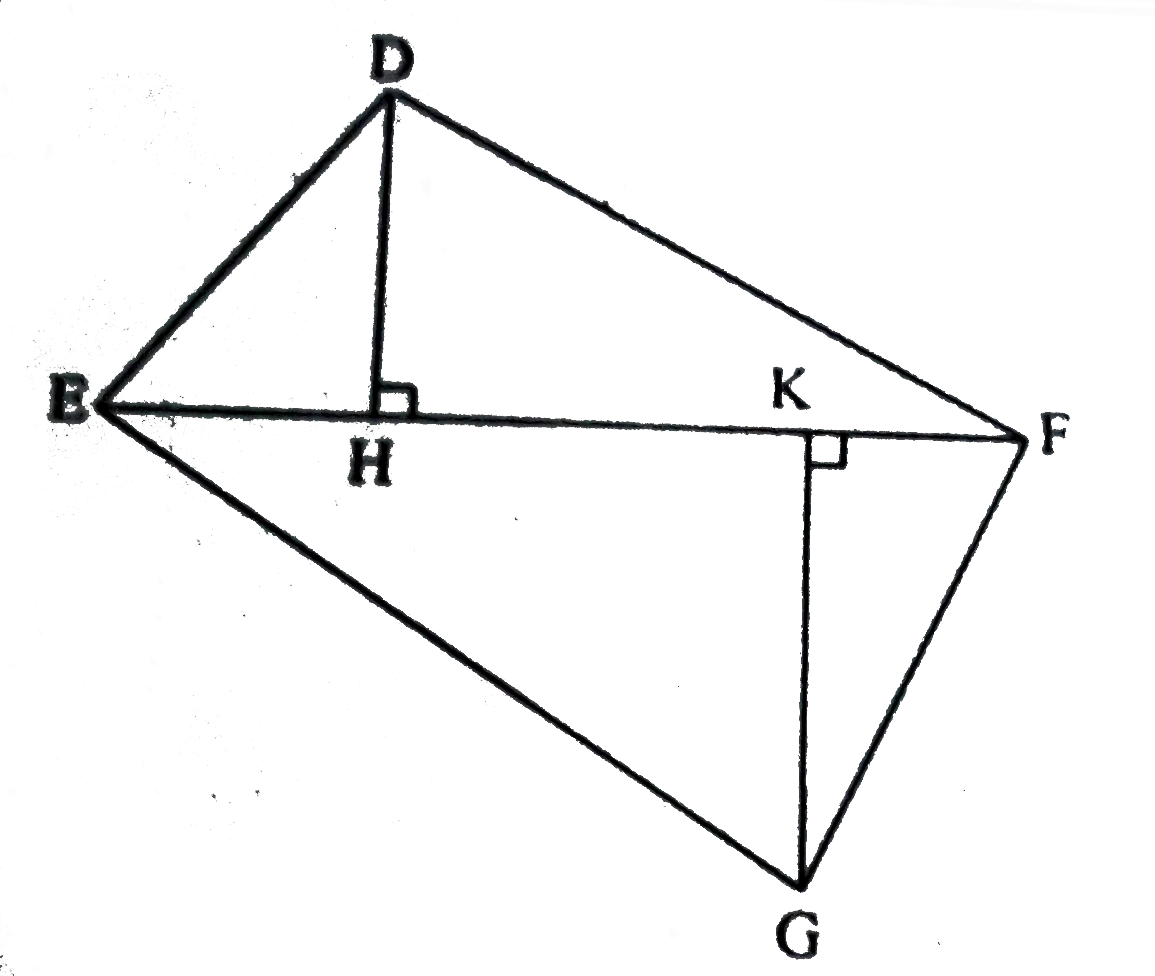
E is a point on hypotenuse dF of ΔDFH, such that seg HE⊥segDF,segEG⊥segFHandsegEK⊥segDH prove that,
(i) EG2=FG×EK
(ii) EK2=DK×EG
 Solution in Tamil
Solution in Tamil
The correct Answer is:x = 5, y = 2 sqrt 5 , z = 3
Step by step video & image solution for E is a point on hypotenuse dF of Delta DFH, such that seg HE bot seg DF, seg EG bot seg FH and seg EK bot seg DH prove that, (i) EG^(2) = FG xx EK (ii) EK^(2) = DK xx EG by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - CHETAN PUBLICATIONCHAPTER - PYTHAGORAS THEOREMEXERCISE - ASSIGNMENT -28videos
PYTHAGORAS THEOREM
- BOOK - CHETAN PUBLICATIONCHAPTER - PYTHAGORAS THEOREMEXERCISE - ASSIGNMENT -31videos
PYTHAGORAS THEOREM
- BOOK - CHETAN PUBLICATIONCHAPTER - PYTHAGORAS THEOREMEXERCISE - Additional MCQ.s10videos
PYTHAGORAS THEOREM
- BOOK - CHETAN PUBLICATIONCHAPTER - MODEL ACTIVITY SHEET EXERCISE - QUESTION29videos
MODEL ACTIVITY SHEET
- BOOK - CHETAN PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - ASSIGNMENT12videos
QUADRATIC EQUATIONS
Similar Questions
Solve the following sub questions:
Is 28,21 and 35 a pythagorean triplet?03:15View SolutionSolve the following sub questions :
State and prove 'Pythagoras theorem'05:46View SolutionIn ΔRST,∠s=90∘,RT=12m,ST=8 m then RS=
02:37View SolutionWhat is the ratio of 1mm 1cm ?
02:24View SolutionIf α and β are the roots of quadratic equation 2x2+4x+3=0, then the value of α+β=
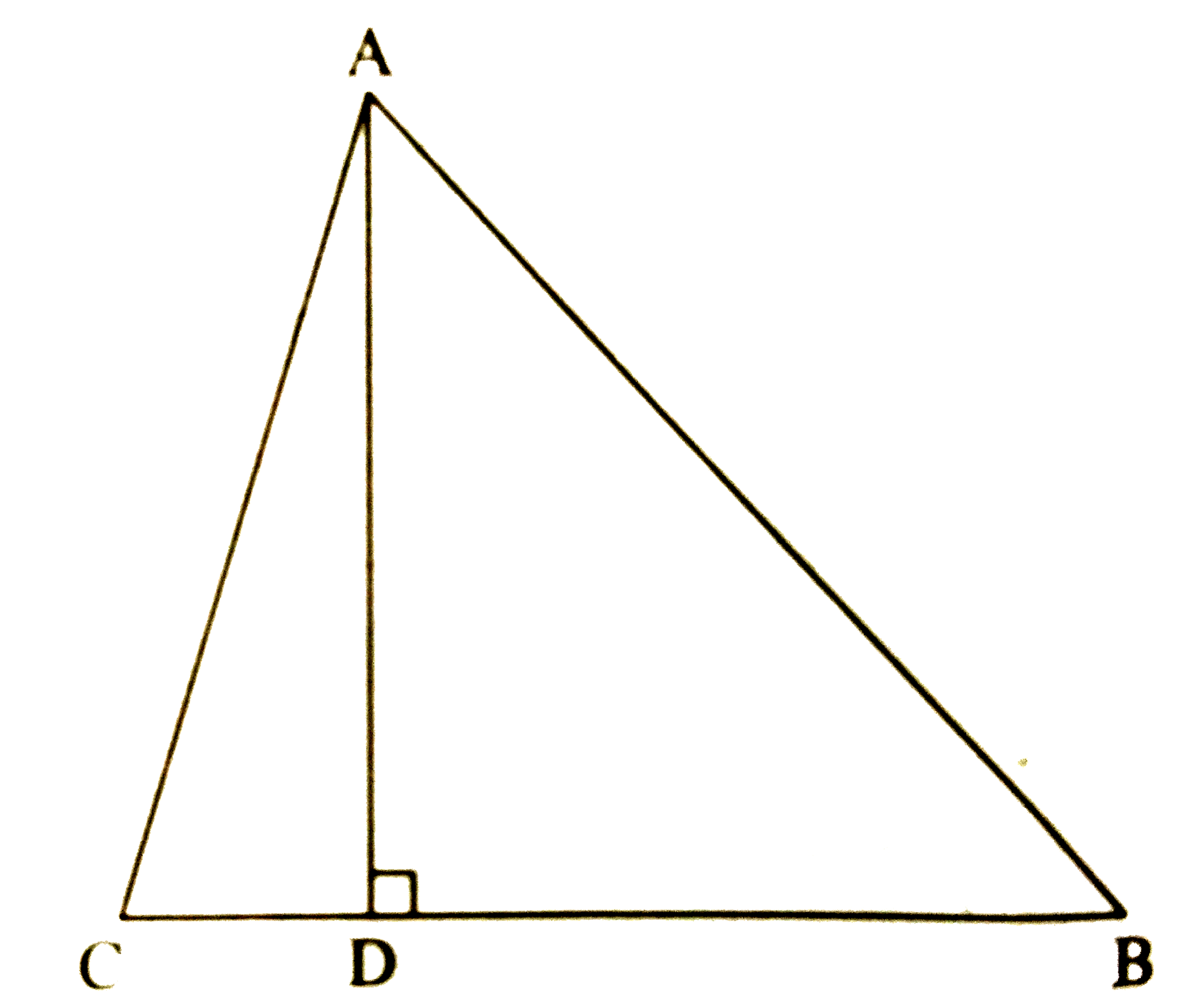
01:58View SolutionIn ΔABC,
seg AD⊥ seg BC,
DB=3CD.
Prove that 2AB2=2AC2+BC2.
 05:33View Solution
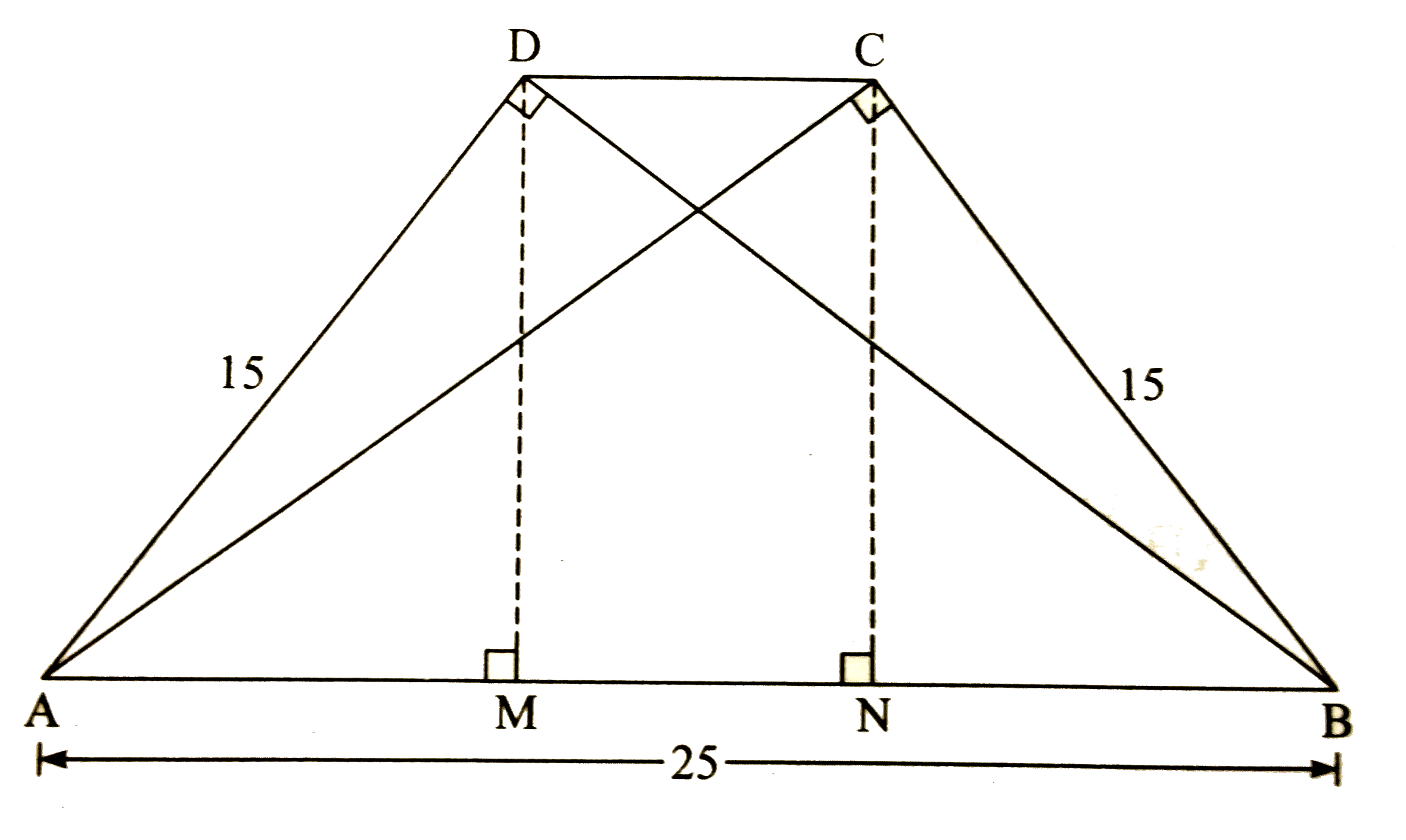
05:33View SolutionIn a trapezium ABCD, seg AB∣∣ seg DC, seg BD⊥ seg AD, seg AC⊥ seg BC, if AD=15, BC=15 and AB=25. Find A(□ABCD).
 03:40View Solution
03:40View SolutionIn the following figure,segDH⊥segEFandsegGK⊥segEF.IfDH=6cm,GK=10cmandA(ΔDEF)=150cm2, then find :
i.EF
ii.A(ΔGEF)
iii.A(□DFGE).
 05:00View Solution
05:00View SolutionIn given figure, seg AE ⊥ seg BC, seg DF ⊥ line BC,
AE = 4, DF = 6, then find A(ΔABC)A(ΔDBC).
 06:40View Solution
06:40View SolutionIn the given figure, seg BE ⊥ seg AB and seg BA ⊥ seg AD. If BE = 6 and AD = 9, then find A(ΔABE)A(ΔBAD).
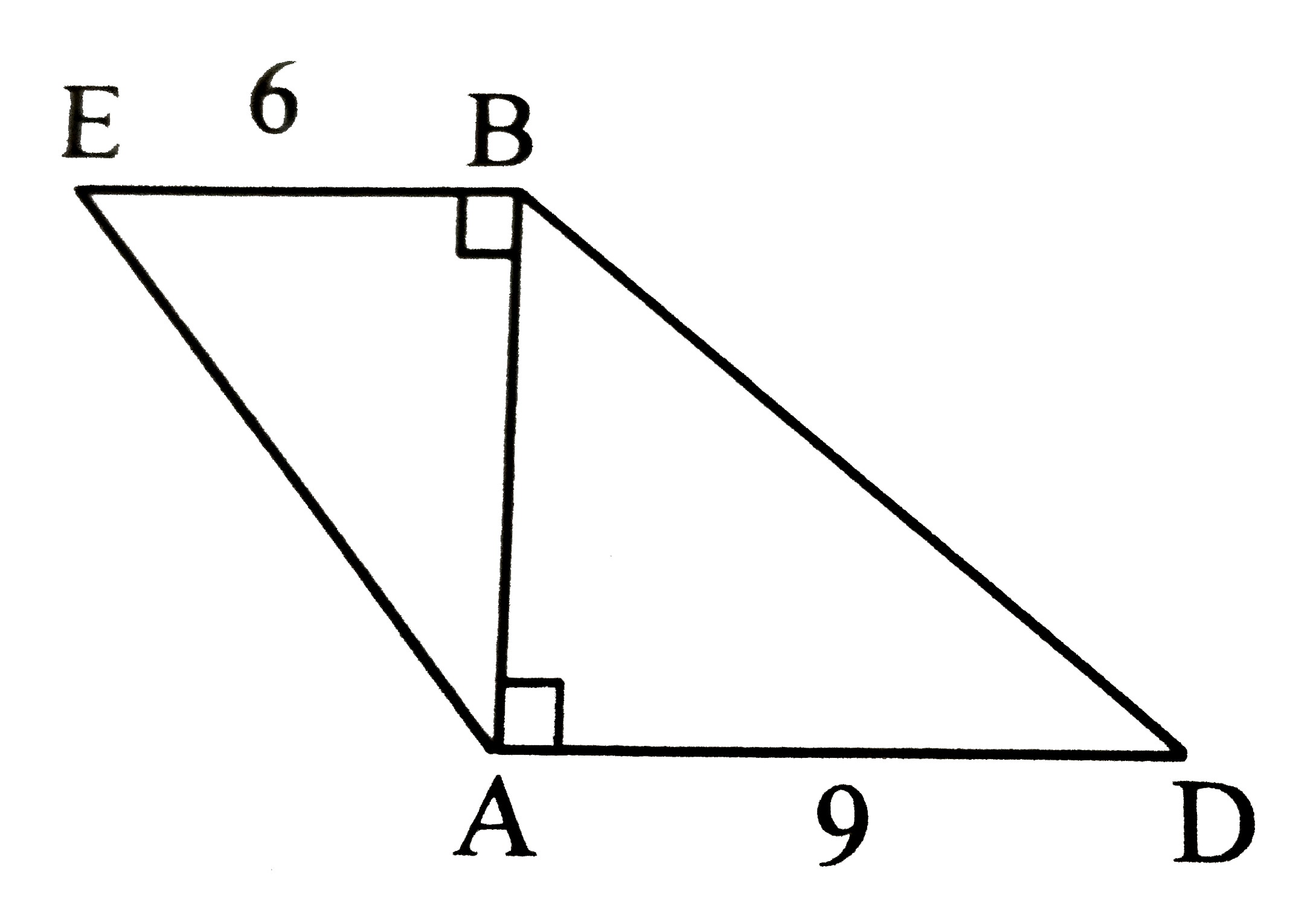 06:23View Solution
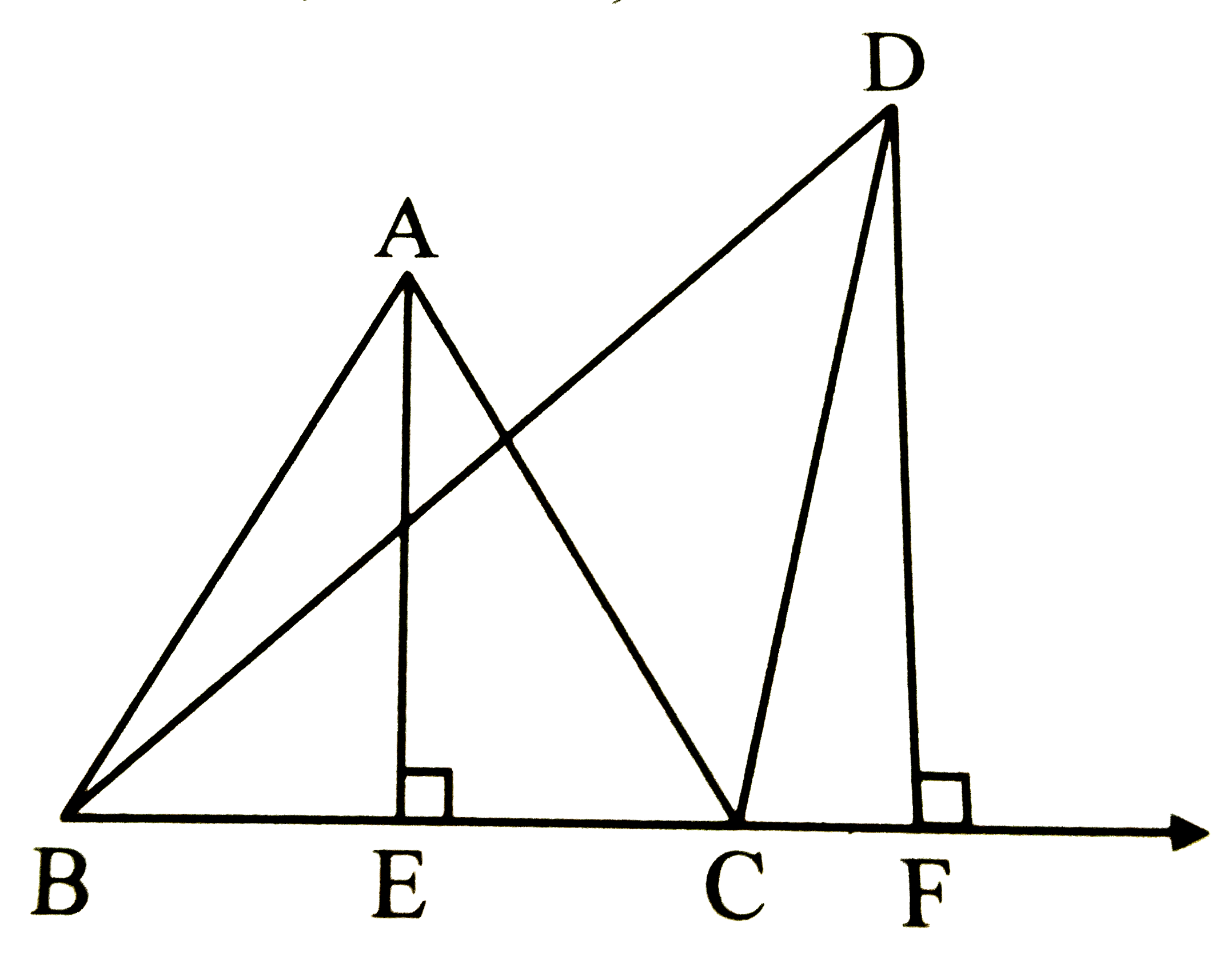
06:23View SolutionIn the given figure, seg AE⊥ seg BC and seg DF ⊥seg BC. Find
i.A(ΔABC)A(ΔDBC)
ii. A(ΔDBF)A(ΔDFC)
iii. A(ΔAEC)A(ΔDBF)
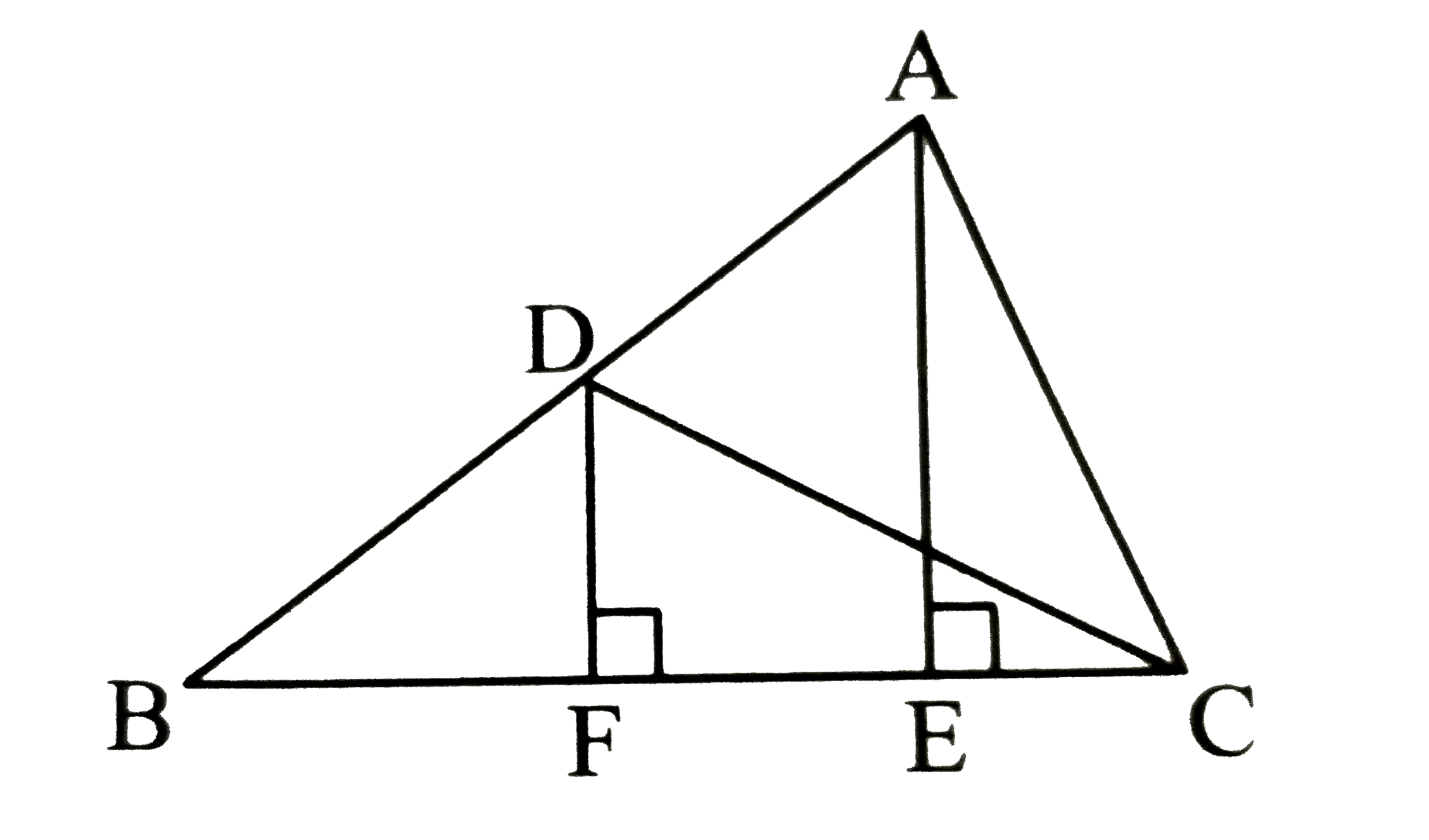 06:40View Solution
06:40View SolutionIn the following figure, seg DH⊥seg EF and seg GK⊥EF. If DH = 6 cm, GK = 10 cm and A(ΔDEF) = 150cm2, then find:
(i) EF
(ii) A(ΔGEF)
(iii) A(□DFGE)
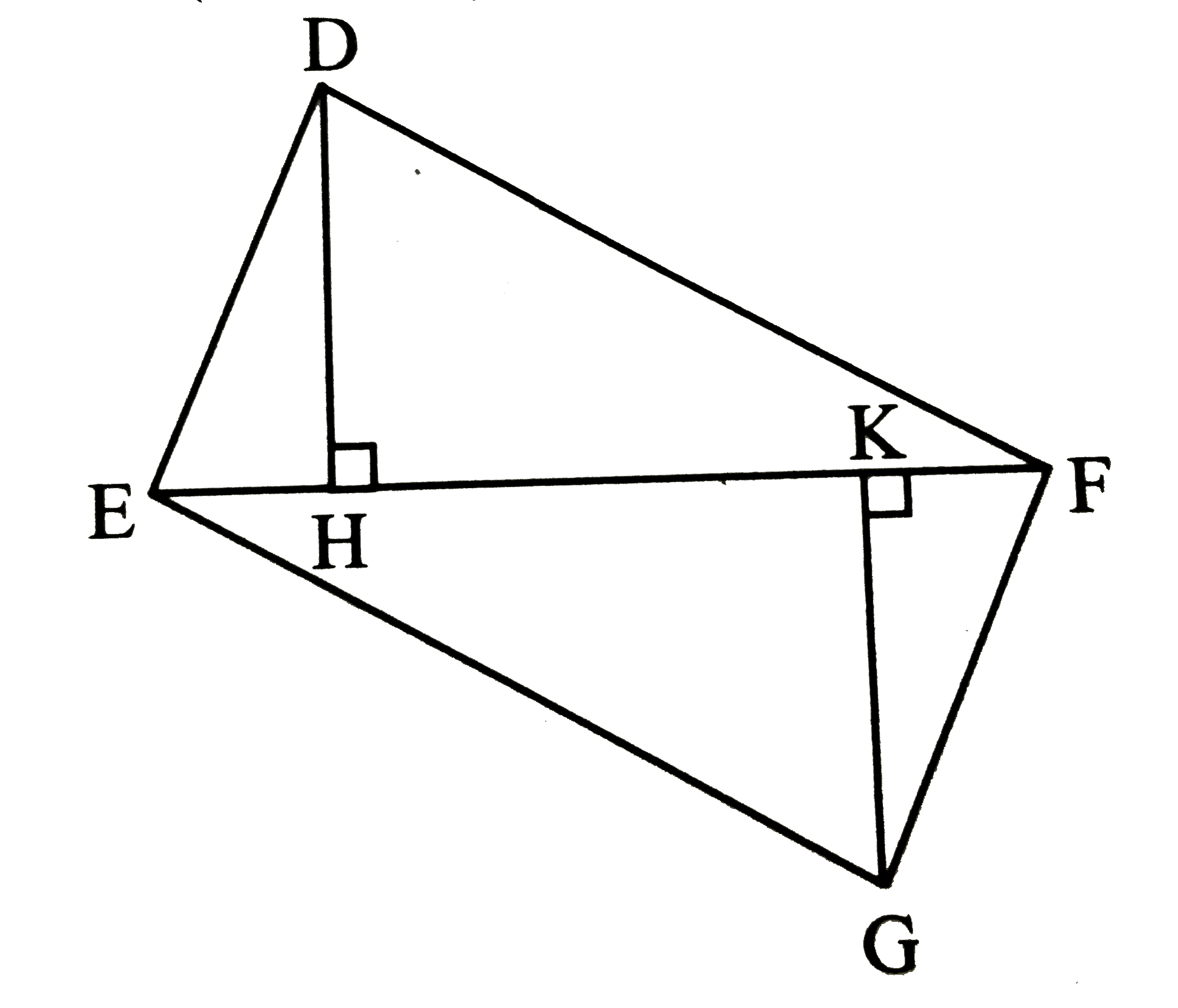 04:04View Solution
04:04View SolutionIn ΔABC,∠ACB=90∘
seg CD ⊥ seg AB
seg DE ⊥ seg CB.
Show that: CD2×AC=AD×AB×DE
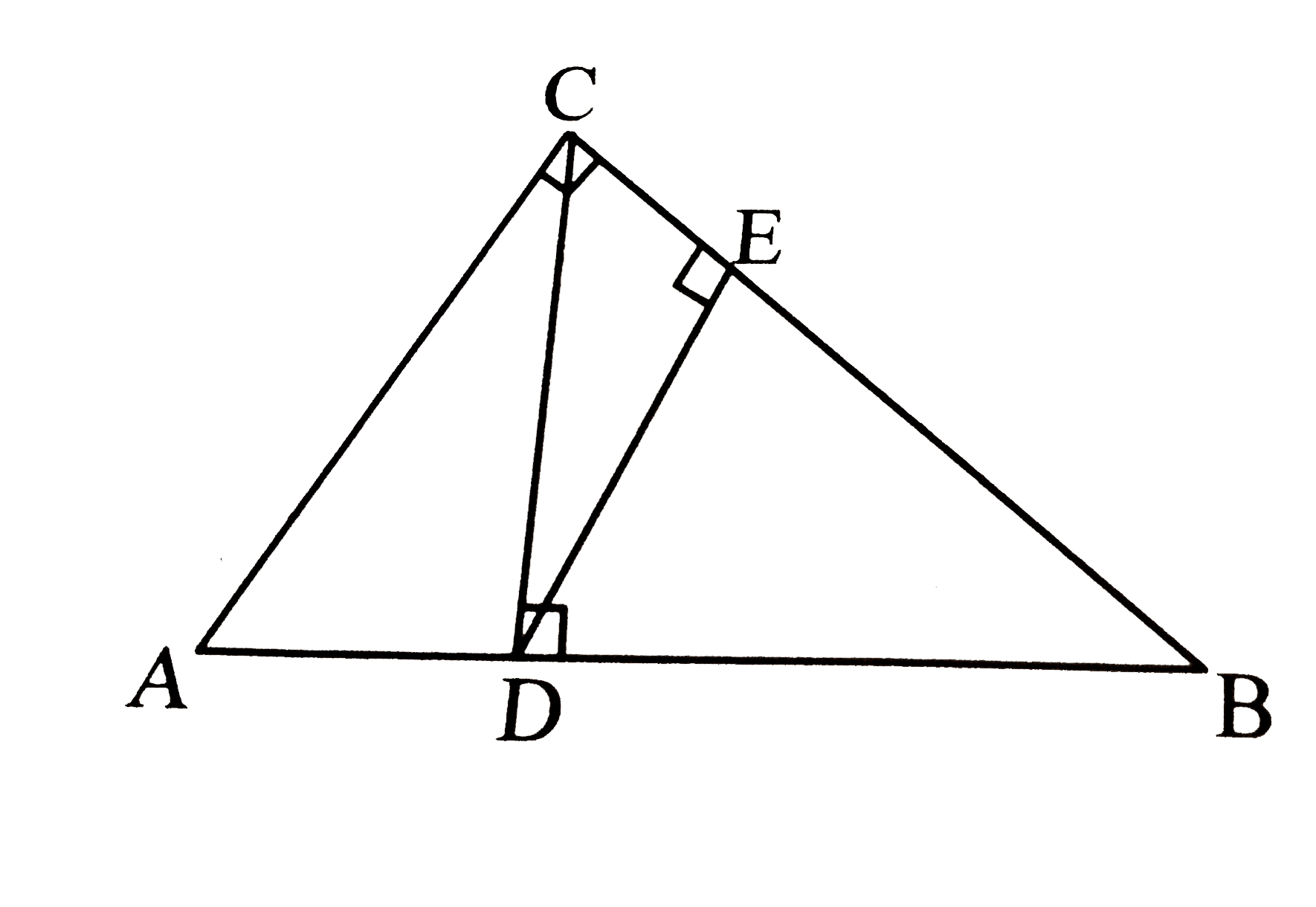 04:52View Solution
04:52View SolutionIn the adjoining figure X id any point in interior of triangle . Point X is joined to vertices of traingle. seg PQ∣∣DE,
seg QR∣∣segEF . Then fill in the blanks to prove that , seg PR∣∣seg DF.
 08:17View Solution
08:17View SolutionIn trapezium ABCD, seg AB ||seg DC. Seg BD bot seg AD, seg AC bot seg BC. If AD = 15, BC = 15 and AB = 25, then find A ( square ABCD)
07:45View Solution

