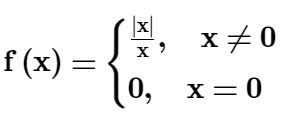Evaluate limx→0f(x), where :
f(x)={x|x|,x≠00,x=0
The correct Answer is:does not exist
To evaluate the limit limx→0f(x), where
f(x)={x|x|if x≠00if x=0
we will analyze the left-hand limit (LHL) and the right-hand limit (RHL) as x approaches 0.
Step 1: Calculate the Left-Hand Limit (LHL)
The left-hand limit is defined as:
limx→0−f(x)
For x<0, we have |x|=−x. Thus,
f(x)=x|x|=x−x=−1
Therefore,
limx→0−f(x)=−1
Step 2: Calculate the Right-Hand Limit (RHL)
The right-hand limit is defined as:
limx→0+f(x)
For x>0, we have |x|=x. Thus,
f(x)=x|x|=xx=1
Therefore,
limx→0+f(x)=1
Step 3: Compare LHL and RHL
Now we compare the two limits:
- LHL as x→0− is −1
- RHL as x→0+ is 1
Since the left-hand limit and the right-hand limit are not equal:
limx→0−f(x)≠limx→0+f(x)
Conclusion
The limit limx→0f(x) does not exist because the left-hand limit and the right-hand limit are not equal.
Thus, the final answer is:
limx→0f(x) does not exist.
---
- RHL as x→0+ is 1
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - LIMITS AND DERIVATIVESEXERCISE - EXERCISE 13 (b)59videos
LIMITS AND DERIVATIVES
- BOOK - MODERN PUBLICATIONCHAPTER - LIMITS AND DERIVATIVESEXERCISE - EXERCISE 13 (c)47videos
LIMITS AND DERIVATIVES
- BOOK - MODERN PUBLICATIONCHAPTER - LIMITS AND DERIVATIVESEXERCISE - FREQUENTLY ASKED QUESTIONS43videos
LIMITS AND DERIVATIVES
- BOOK - MODERN PUBLICATIONCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - CHAPTER TEST12videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
- BOOK - MODERN PUBLICATIONCHAPTER - LINEAR INEQUATIONSEXERCISE - CHAPTER TEST12videos
LINEAR INEQUATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One or More
Let g(x)=xf(x), where f(x)={x2sin.1x:x≠00:x=0. At x=0,
Ag is differentiable but g' is not continuousBg is differentiable while f is not differentiableCboth f and g are differentiableDg is differentiable but g' is continuous
Evaluate : limx→0f(x)={|x|x,x≠0 0,x=0.
View SolutionEvaluate (lim)x→0f(x), where f(x)={|x|x, x≠00, x=0
View SolutionFind limx→0f(x), where f(x)=|x|−5.
View SolutionEvaluate (lim)x→0f(x), where f(x)=[|x|x,x≠0,0,x=0
View SolutionFind limx→0f(x) and limx→1f(x), where
f(x)={2x+3,x≤03(x+1),x>0View SolutionCalculate limx→0f(x), where f(x)=1x2 for x>0
View SolutionFind limx→0f(x), where f(x)=⎧⎪⎨⎪⎩x−1x<00x=0x=1x>0
View Solution