Find the area bounded by the curve y=2cosx and the X-axis from x = 0 to x=2π.
The correct Answer is:N/a
Required area of shaded region =∫2π02cosxdx
=∫π/202cosxdx+∣∣∣∫3π/2π/22cosxdx∣∣∣+∫2π3π/22cosxdx
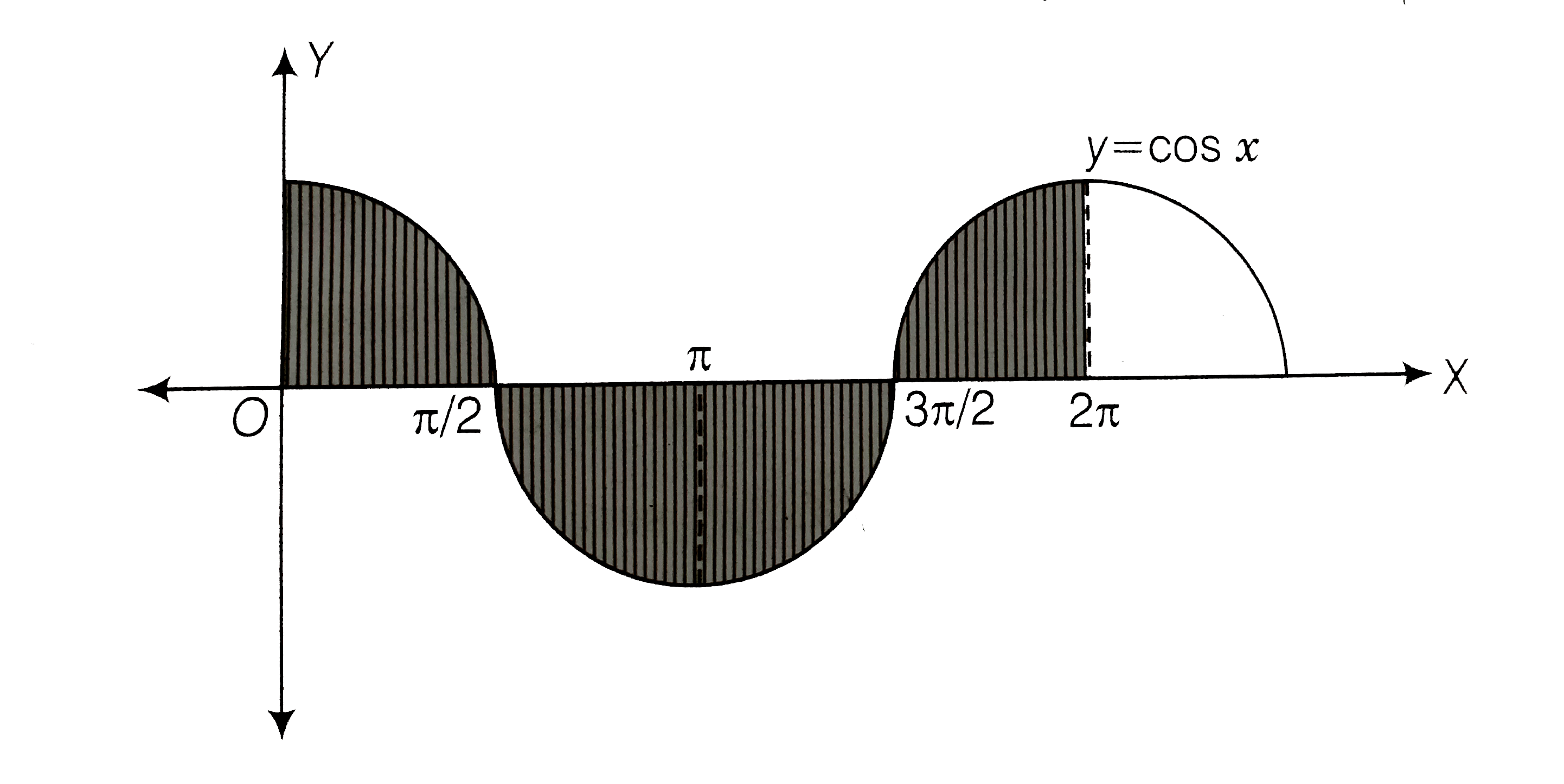
=2[sinx]π/20+∣∣2(sinx)3π/2π/2∣∣+2[sinx]2π3π/2
=2+4+2=8 sq units
Topper's Solved these Questions
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions
22 Videos
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions
22 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers
5 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - True/False
10 Videos
Similar Questions
Find the area bounded by the curve y=x3−3x2+2x and the x-axis.
View SolutionThe area bounded by the curve y=4x−x2 and the x-axis is:
View SolutionFind the area bounded by the curve y=x2,x=1,x=2 and x-axis .
View SolutionFind the area bounded by the curve y=x(x−1)(x−2) and the x-axis.
View SolutionThe area bounded by the curve y=sin−1(sinx) and the x - axis from x=0 to x=4π is equal to the area bounded by the curve y=cos−1(cosx) and the x - axis from x=−π to x=a, then the value of a is equal to
View SolutionFind the area bounded by the curves x+2|y|=1 and x=0 .
View SolutionFind the area bounded by the curves x+2|y|=1 and x=0 .
View SolutionFind the area bounded by the curve |x|+y=1 and axis of x.
View SolutionThe area bounded by the curve xy2=a2(a−x) and y-axis is
View SolutionFind the area bounded by the curve y=e−x the X-axis and the Y-axis.
View Solution
