Find the area bounded by y=∣∣∣sinx−12∣∣∣andy=1 for x∈[0,π]Solution in Bengali
The correct Answer is:(π2+2)sq. units
y=∣∣∣sinx−12∣∣∣=⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩12−sinx,0≤x≤π6sinx−12,π6<x<5π612−sinx,5π6≤x≤π
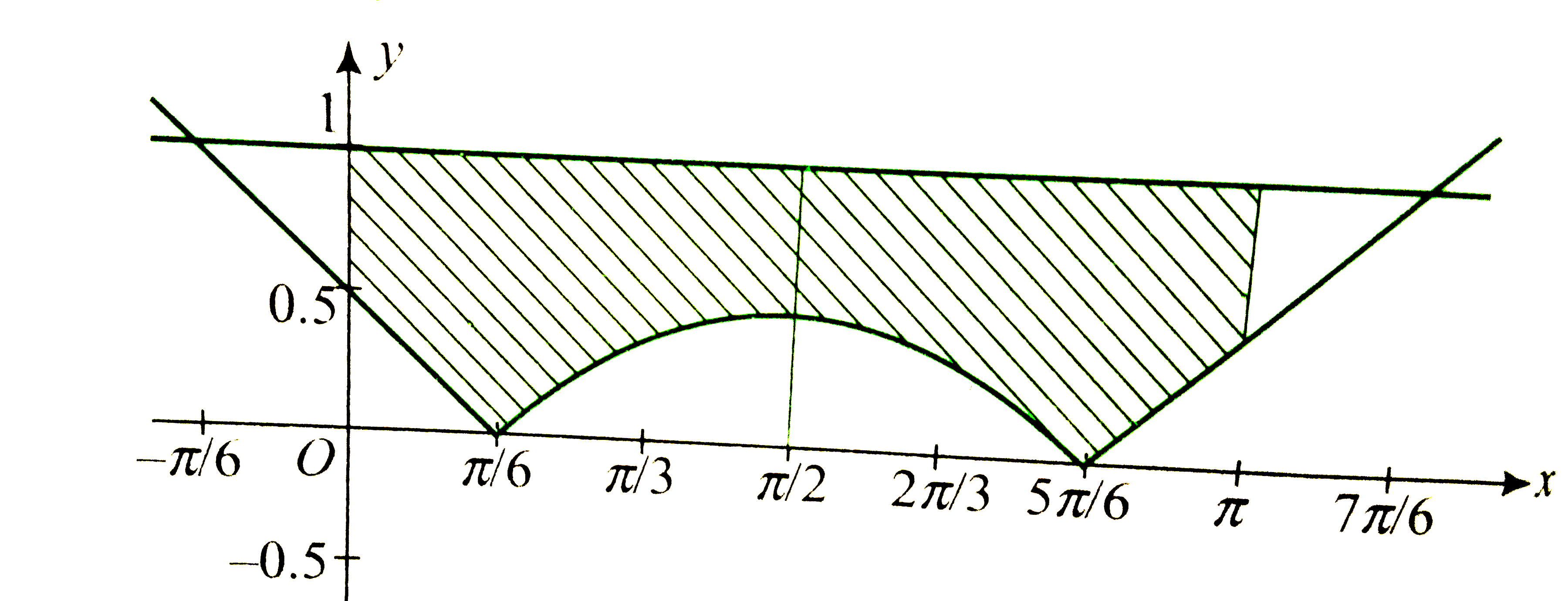
∴ Required area is
A=π−2⎛⎜
⎜
⎜⎝π2∫0(12−sinx)dx+π2∫0(sinx−12)dx⎞⎟
⎟
⎟⎠
π−2(12x+cosx)π/60+2(−cosx−12)π/2π/6
=π2+2
Topper's Solved these Questions
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Concept Application Exercise 9.2
14 Videos
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Concept Application Exercise 9.3
7 Videos
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Solved Examples
10 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGE PUBLICATIONCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
Similar Questions
Find the area lying in the first quadrant and bounded by the curve y=x3 and the line y=4x.
View SolutionFind the continuous function f where (x4−4x2)≤f(x)≤(2x2−x3) such that the area bounded by y=f(x),y=x4−4x2. then y-axis, and the line x=t, where (0≤t≤2) is k times the area bounded by y=f(x),y=2x2−x3,y-axis , and line x=t(where0≤t≤2).
View SolutionFind the area bounded by the curve x2=y,x2=−yandy2=4x−3
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionFind the area bounded by the y-axis, y=cosx,andy=sinxwhen0≤x≤π2.
View SolutionFind the area bounded by the curve y=sin−1x and the line x=0,|y|=π2.
View SolutionFind the area bounded by the curves y=(sin^(-1)(sin x)+cos^(-1)(cos x)) and y=(sin^(-1)(sin x)+cos^(-1)(cos x))^(2) for 0<=x<=2 pi
View SolutionFind the area bounded by y=∣∣∣sinx−12∣∣∣andy=1 for x∈[0,π]
View SolutionFind the area bounded by y = sin x and axis of x in (0,π)
View SolutionFind the area bounded by y=1+2sin2x,X-axis,X=0 and x=π.
View SolutionThe area bounded by y=cos−1 (Sin x) and y=sin−1 (sin x) on the interval (0, π) is
View SolutionFind the area bounded by the curve y=sin−1x and the line x=0,|y|=π2.
View SolutionFind the area bounded by the curve y=sin−1x and the line x=0,|y|=π2.
View SolutionFind the area bounded by y=∣∣∣sinx−12∣∣∣andy=1 for x∈[0,π]
View SolutionFind the area of the region bounded by
y= sin x and y= cos x between x=0 and x=π2
View Solution
