Find the area of circle $4 x^{2} + 4 y^{2} = 9$ which is interior to the parabola $x^{2} = 4 y$

Video Solution

Text Solution

Verified by Experts

Given equation of circle is $4 x^{2} + 4 y^{2} = 9$ and equation of parabola is $x^{2} = 4 y$ .

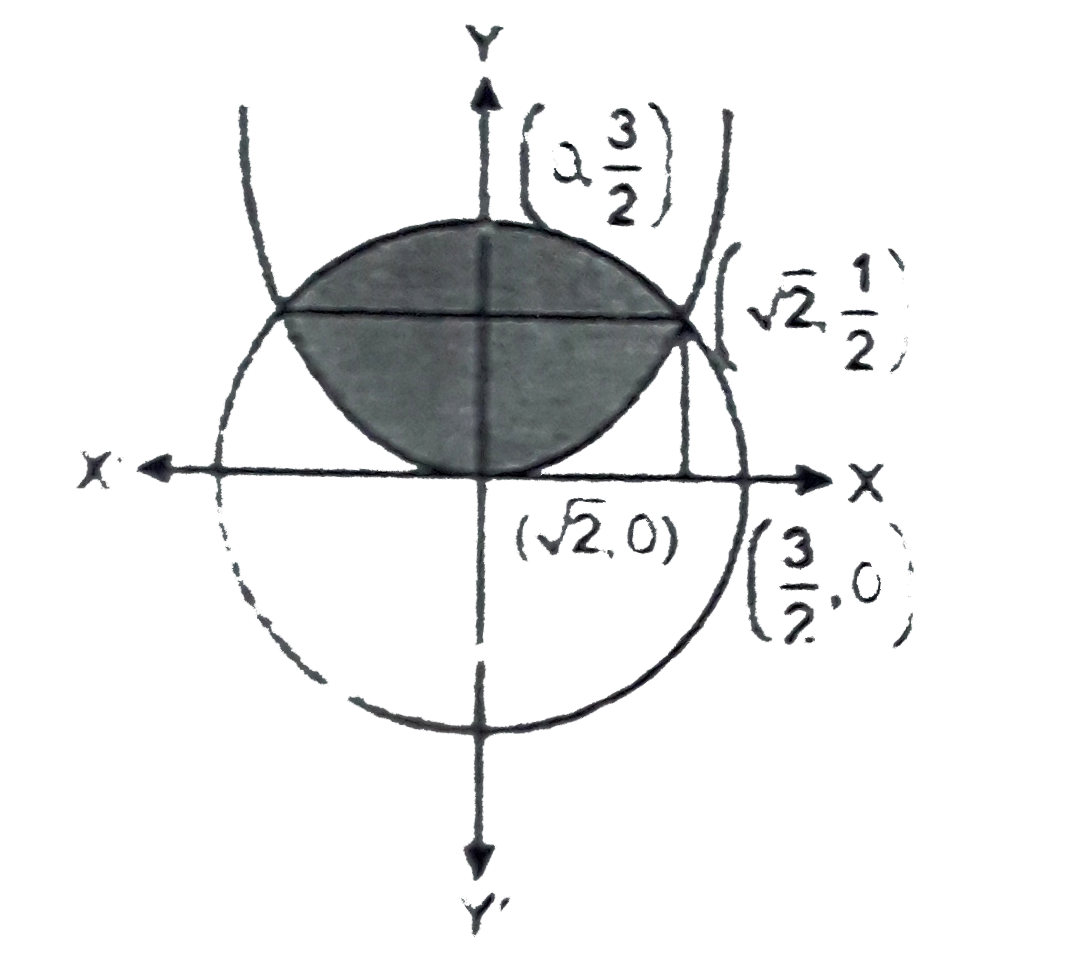
Now, solving the equations of two curves,
$4 (4 y) + 4 y^{2} = 9$
$\Rightarrow 4 y^{2} + 16 y - 9 = 0$
$\Rightarrow y = \frac{- 16 \pm \sqrt{256 + 144}}{2 \times 4}$
$= \frac{- 16 \pm \sqrt{400}}{8}$
$= \frac{- 16 \pm 20}{8} = \frac{1}{2}, - \frac{9}{2}$
but y gt 0, so two curves will meet when $y = \frac{1}{2}$ ,
$∴ x^{2} = 4 \times \frac{1}{2} = 2$
$\Rightarrow x = \pm \sqrt{2}$
$∴ Required area = 2 \int_{0}^{\sqrt{2}} (y_{2} - y_{1}) d x$
$= {\int_{0}^{\sqrt{2}} \sqrt{\frac{9 - 4 x^{2}}{4}} d x - \int_{0}^{\sqrt{2}} \frac{x^{2}}{4} d x}$
$= 2 \int_{0}^{\sqrt{2}} \sqrt{{(\frac{3}{2})}^{2} - x^{2}} d x - \frac{2}{4} {[\frac{x^{3}}{3}]}_{0}^{\sqrt{2}}$
$= 2 {⎡ ⎣ \frac{x}{2} \sqrt{{(\frac{3}{2})}^{2} - x^{2}} + \frac{{(3 / 2)}^{2}}{2} {sin}^{- 1} (\frac{x}{3 / 2}) ⎤ ⎦}_{0}^{- 1} - \frac{1}{6} [{(\sqrt{2})}^{3} - 0]$
$= {[x \sqrt{\frac{9}{4} - x^{2}} + \frac{9}{4} {sin}^{- 1} (\frac{2 x}{3})]}_{0}^{- 1} - \frac{2 \sqrt{2}}{6}$
$= [\sqrt{2} \sqrt{\frac{9}{4} - 2} + \frac{9}{4} {sin}^{- 1} (\frac{2 \sqrt{2}}{3})] - {0 - 0} - \frac{2 \sqrt{2}}{6}$
$= \frac{\sqrt{2}}{2} + \frac{9}{4} {sin}^{- 1} (\frac{2 \sqrt{2}}{3}) - \frac{2 \sqrt{2}}{6}$
$= (\frac{\sqrt{2}}{2} - \frac{\sqrt{2}}{3}) + \frac{9}{4} {sin}^{- 1} (\frac{2 \sqrt{2}}{3})$
$= \frac{\sqrt{2}}{6} + \frac{9}{4} {sin}^{- 1} (\frac{2 \sqrt{2}}{3})$ sq. units.

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-APPLICATIONS OF INTEGRALS-Exercise 8.2

Home
Ask Doubt
Profile