Find the area of the region enclosed by y=−5x−x2andy=x on interval [−1,5]
Video Solution
Text Solution
Solving the given curves we get
5x−x2=x
∴ x2−4x=0
⇒ x=0,4
y=x(5−x) is parabola which is concave downward and intersecting x-axis at x=0 and x=5.
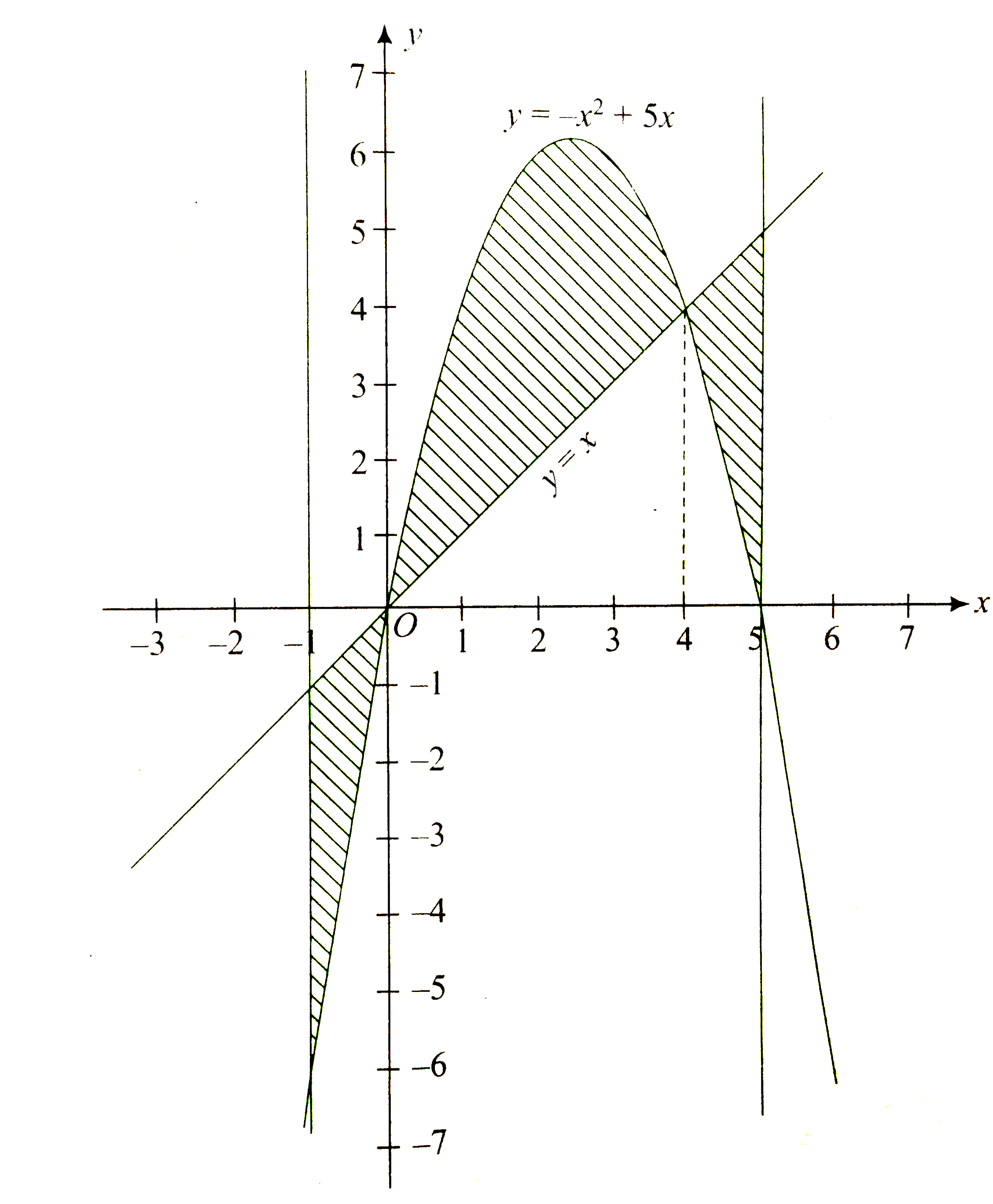
Required area, =5∫−1∣∣x−(5x−x2)∣∣dx
=5∫−1|x−4x|dx
=0∫−1(x2−4x)dx+4∫0(4x−x2)dx+5∫4(x2−4x)dx
=[x33−2x2]0−1+[2x2−x33]40+[x33−2x2]
=−[−13−2]+[32−643]+[1253−50]−[643−32]
=16−23
463 sq. units.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.19videos
AREA
- BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.214videos
AREA
- BOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Question Bank29videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank20videos
AREA UNDER CURVES
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of the region enclosed by y=x3−2x2+2andy=3x+2 is
A716B14C293D713 - Question 2 - Select One
What are the area of the region enclosed by y=2|x| and y=4?
A2 sq. unitsB4 sq.unitsC8 sq. unitsD16 sq. units - Question 3 - Select One
What is the area of the region enclosed byy=2|x| and y=4 ?
A2 square unitB4 square unitC8 square unitD16 squre unit
Find the area of the region bounded by the parabola y=x2 and y=|x|.
View SolutionFind the area of the region enclosed by the curves y=xlogxandy=2x−2x2.
View SolutionFind the area of the region enclosed between curves y=|x−1| and y=3−|x|.
View SolutionFind area of region bounded by y=x2–3x+2,x=1,x=2 and y=0.
View SolutionFind the area of the region enclosed by the curve y=x4–2x2andy=2x2.
View Solution

