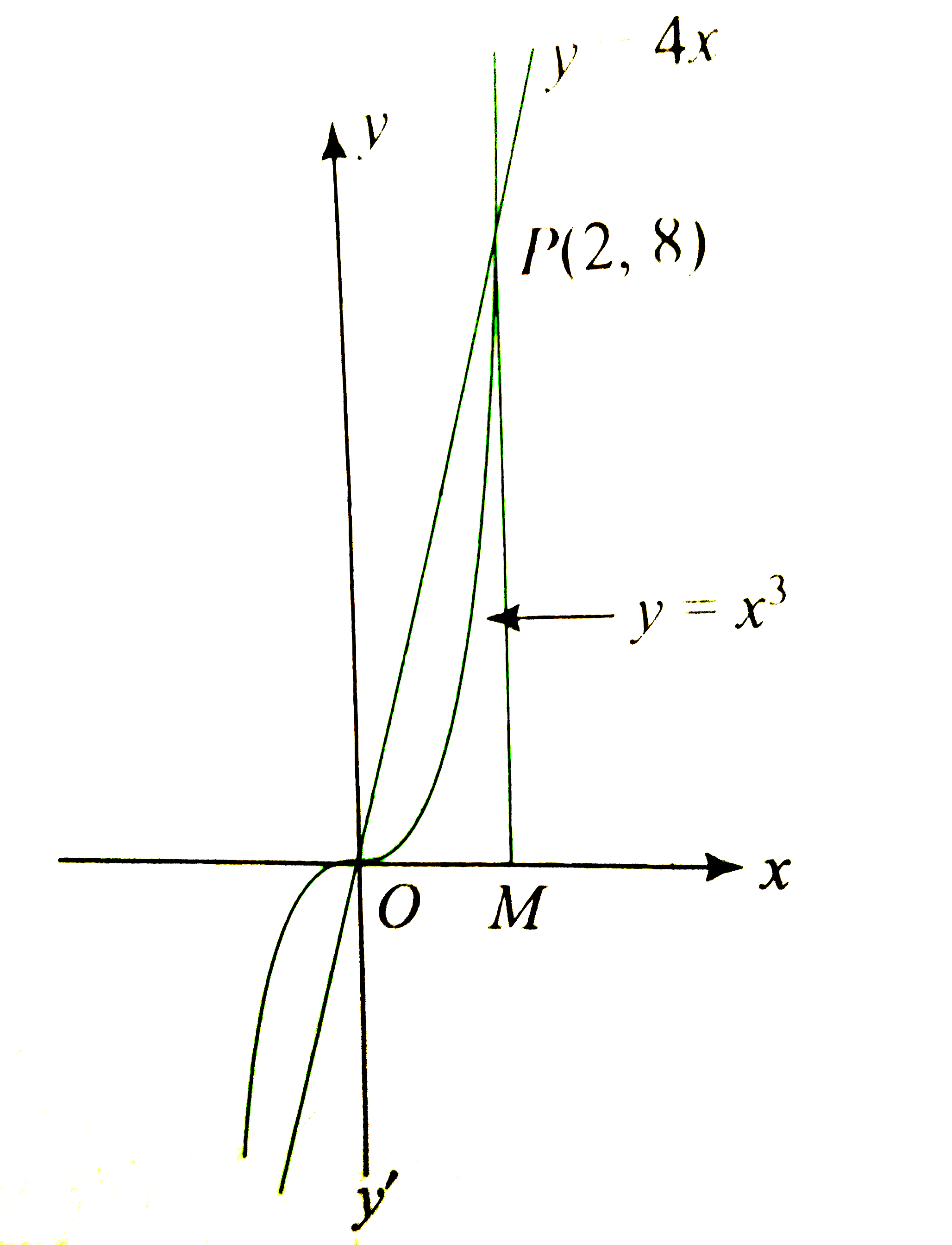
Find the area lying in the first quadrant and bounded by the curve $y = x^{3}$ and the line $y = 4 x .$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4 sq. units

$The line y = 4 x meets y = x^{3} at 4 x = x^{3}$ .
$∴ x = 0, 2, - 2 \Rightarrow y = 0, 8, - 8$
$\Rightarrow A = \int_{0}^{2} (4 x - x^{3}) = {(2 x^{2} - \frac{x^{4}}{4})}_{0}^{2} = 4$ sq. units

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.3
7videos
AREA
BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise (Single)
40videos
AREA
BOOK - CENGAGECHAPTER - AREAEXERCISE - Exercise 9.1
9videos
AREA
BOOK - CENGAGECHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Question Bank
29videos
APPLICATIONS OF DERIVATIVES
BOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
20videos
AREA UNDER CURVES

Similar Questions

Knowledge Check

Question 1 - Select One
Find the area lying in the first quadrant and bounded by the curve $y = x^{3}$ and the line $y = 4 x .$
A2
B3
C4
D5
Question 2 - Select One
The area lying in the first quadrant and bounded by the circle $x^{2} + y^{2} = 4$ and the line $x = \sqrt{3} y$ is :
A $\frac{π}{3}$
B $\frac{π}{2}$
C $π$
D $\frac{π}{5}$
Question 3 - Select One
Area lying in the first quadrant and bounded by the circle $x^{2} + y^{2} = 4$ and the lines $x = 0 and x = 2$ is :
A $π$
B $\frac{π}{2}$
C $\frac{π}{2}$
D $\frac{π}{4}$

CENGAGE-AREA-Exercise 9.2

Home
Ask Doubt
Profile