Question

Find the area region bounded by the triangle whose vertices are $(-1,1),(0,5)\text{and}(3,2),$ using integration.

Explanation

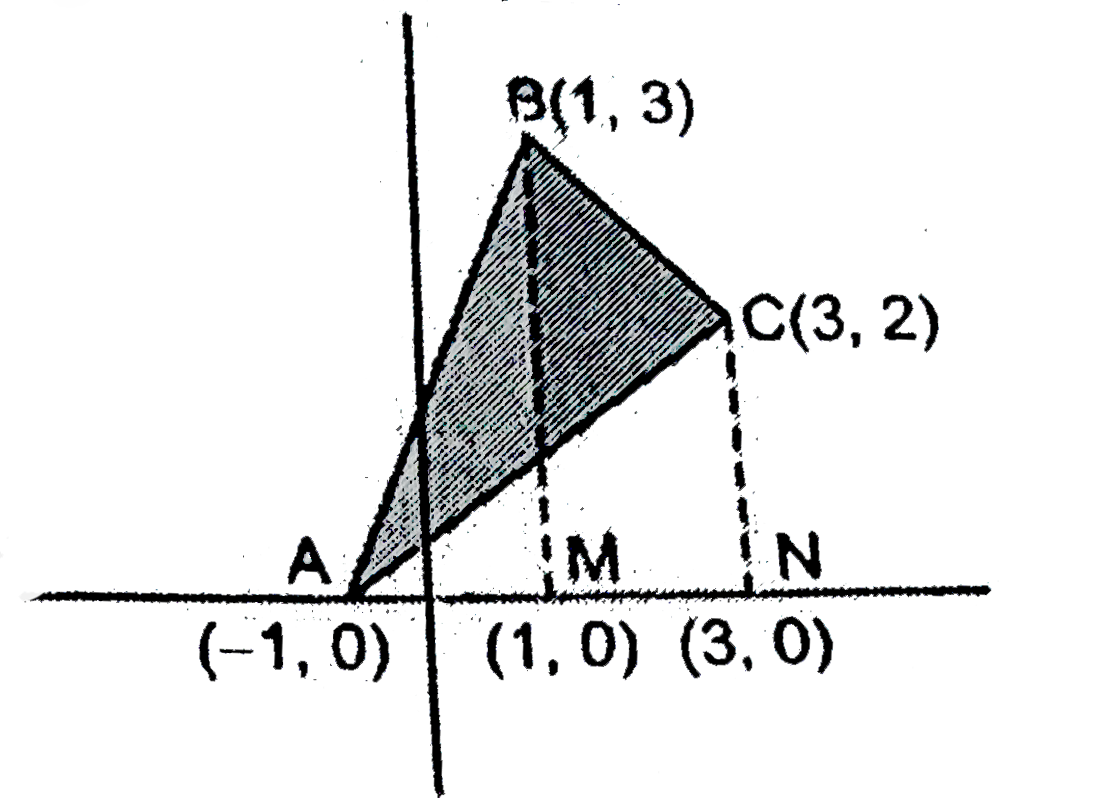
Let the given three points be A (-1, 0), B(1, 3) and C(2, 2).
We know that the equation of a line passing through the points `(x_(1), y_(1))` and `(x_(2), y_(2))` is
`(y-y_(1))=(y_(2)-y_(1))/(x_(2)-x_(1))(x-x_(1)).`
` :. ` Equation of line AB,
`y-0=(3-0)/(1+1)(x+1)impliesy=(3)/(2)(x+1)`
Equation of line BC,
`y-3=(2-3)/(3-1)(x-1)`
`impliesy= -(1)/(2)x+(1)/(2)+3implies y= -(1)/(2)x+(7)/(2)`
and equation of line AC,
`y-0=(2-0)/(3+1)(x+1)impliesy=(1)/(2)x+(1)/(2)`
`ar(triangleABC)=ar(triangle AMB)+ar(squareBMNC)-ar(triangle ANC)`
`=int_(-1)^(1)(3)/(2)(x+1)dx+int_(1)^(3)(-(1)/(2)x+(7)/(2))dx-int_(-1)^(3)((1)/(2)x+(1)/(2))dx`
`=(3)/(2)[(x^(2))/(2)+x]_(-1)^(1)+[-(x^(2))/(4)+(7)/(2)x]_(1)^(3)-[(x^(2))/(4)+(1)/(2)x]_(-1)^(3)`
`=(3)/(2)[(1)/(2)+1-(1)/(2)-(-1)]+(-(9)/(4)+(21)/(2)+(1)/(4)-(7)/(2))-((9)/(4)+(3)/(2)-(1)/(4)+(1)/(2))`
`=(3)/(2)(2)+(7-2)-(2+2)`
`=3+5-4=4` sq. units.