Find the mean, variance and standard deviation for the following data: 227,235,255,269,292,299,312,321,333,348
To find the mean, variance, and standard deviation for the given data set: 227, 235, 255, 269, 292, 299, 312, 321, 333, 348, we will follow these steps:
Step 1: Calculate the Mean
The mean (average) is calculated using the formula:
Mean=Sum of all observationsn
Where n is the number of observations.
1. Sum of observations:
227+235+255+269+292+299+312+321+333+348=2891
2. Number of observations:
n=10
3. Calculate Mean:
Mean=289110=289.1
Step 2: Calculate the Variance
Variance is calculated using the formula:
Variance=1n∑(xi−¯x)2
Where ¯x is the mean.
1. Calculate xi−¯x for each observation:
- 227−289.1=−62.1
- 235−289.1=−54.1
- 255−289.1=−34.1
- 269−289.1=−20.1
- 292−289.1=2.9
- 299−289.1=9.9
- 312−289.1=22.9
- 321−289.1=31.9
- 333−289.1=43.9
- 348−289.1=58.9
2. Square each of these results:
- (−62.1)2=3855.61
- (−54.1)2=2925.81
- (−34.1)2=1169.21
- (−20.1)2=404.01
- (2.9)2=8.41
- (9.9)2=98.01
- (22.9)2=524.41
- (31.9)2=1017.61
- (43.9)2=1924.81
- (58.9)2=3468.81
3. Sum of squared differences:
3855.61+2925.81+1169.21+404.01+8.41+98.01+524.41+1017.61+1924.81+3468.81=8516.5
4. Calculate Variance:
Variance=8516.510=851.65
Step 3: Calculate the Standard Deviation
Standard deviation (SD) is the square root of the variance:
Standard Deviation=√Variance=√851.65≈29.2
Final Results
- Mean: 289.1
- Variance: 851.65
- Standard Deviation: 29.2
The mean (average) is calculated using the formula:
227+235+255+269+292+299+312+321+333+348=2891
n=10
Mean=289110=289.1
Variance is calculated using the formula:
- 227−289.1=−62.1
- 235−289.1=−54.1
- 255−289.1=−34.1
- 269−289.1=−20.1
- 292−289.1=2.9
- 299−289.1=9.9
- 312−289.1=22.9
- 321−289.1=31.9
- 333−289.1=43.9
- 348−289.1=58.9
- (−62.1)2=3855.61
- (−54.1)2=2925.81
- (−34.1)2=1169.21
- (−20.1)2=404.01
- (2.9)2=8.41
- (9.9)2=98.01
- (22.9)2=524.41
- (31.9)2=1017.61
- (43.9)2=1924.81
- (58.9)2=3468.81
3855.61+2925.81+1169.21+404.01+8.41+98.01+524.41+1017.61+1924.81+3468.81=8516.5
Variance=8516.510=851.65
Standard deviation (SD) is the square root of the variance:
- Mean: 289.1
- Variance: 851.65
- Standard Deviation: 29.2
Topper's Solved these Questions
Similar Questions
Find the mean variance and standard deviation for the following data: 2,4,5,6,8,17
View SolutionFind the variance and standard deviation from the following data:
 View Solution
View SolutionFind the mean, variance and standard deviation for the following data: 6,7,10,12,13,4,8,12
View SolutionFind the standard deviation for the following data
 View Solution
View SolutionFind the standard deviation for the following data
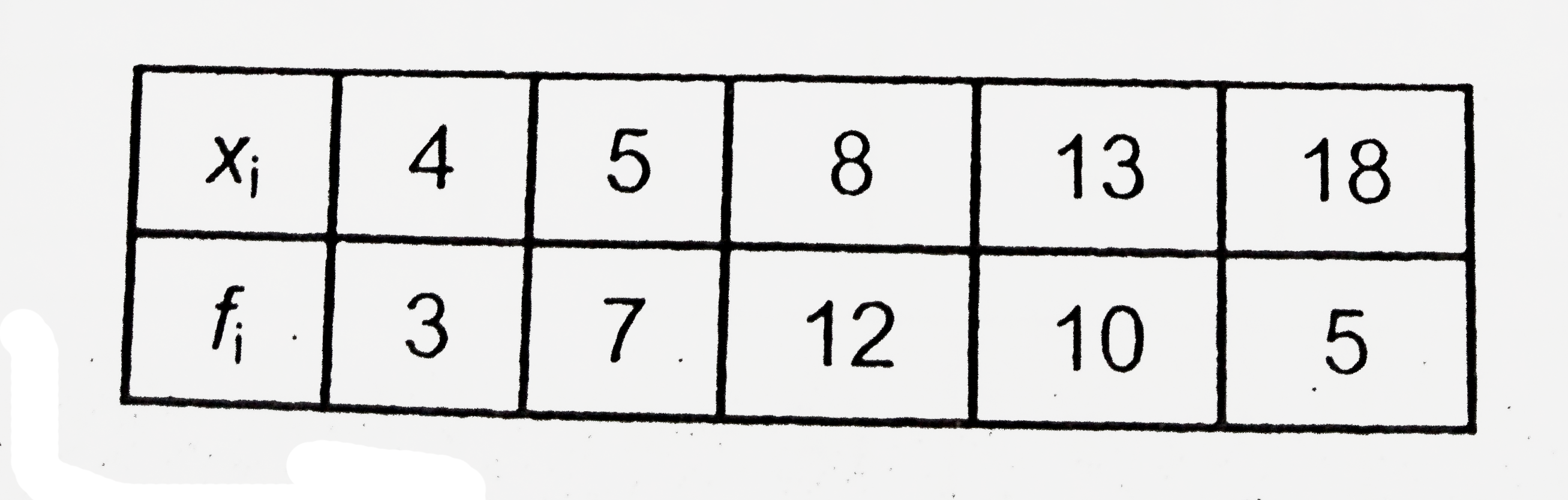 View Solution
View SolutionFind the variance and standard deviation of the following data:
 View Solution
View SolutionCalculate the mean, variance and standard devation for the following data :
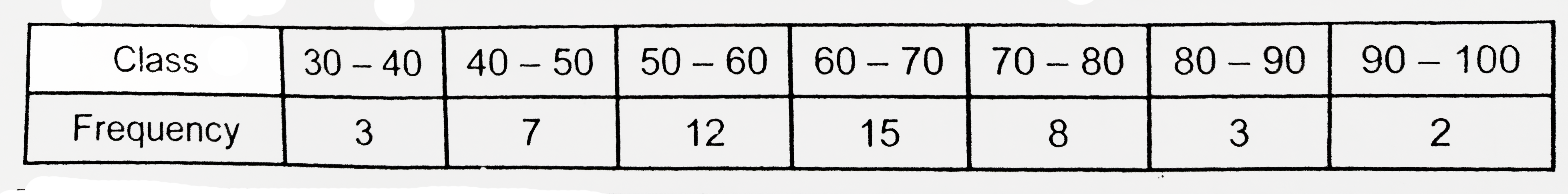 View Solution
View SolutionFind the variance and standard deviation for the following distribution.
 View Solution
View SolutionFind the standard deviation from the following data:
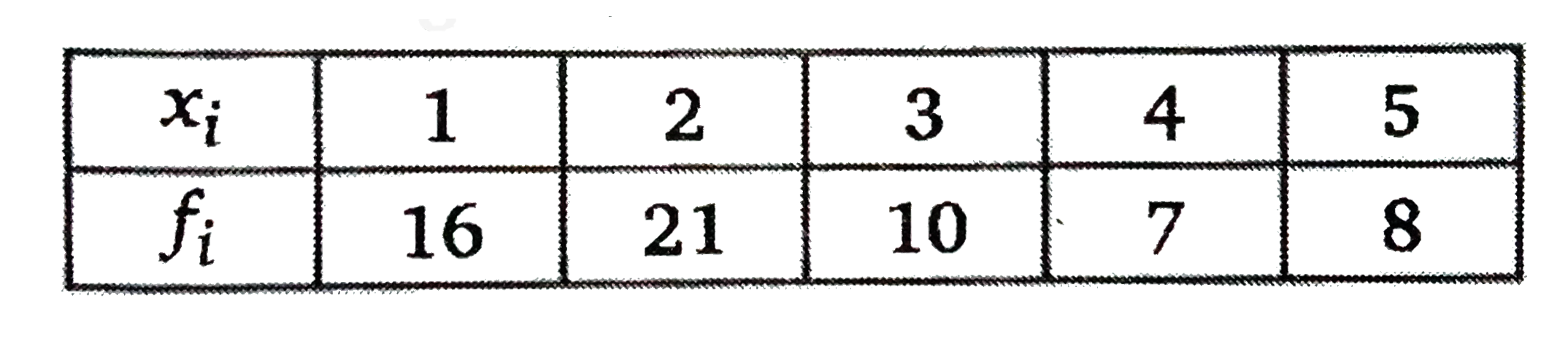 View Solution
View SolutionFind the variance and standard deviation of the following distribution
 View Solution
View Solution

