Find the mean, variance and standard deviation for the following data: 6,7,10,12,13,4,8,12
To find the mean, variance, and standard deviation for the given data set: 6, 7, 10, 12, 13, 4, 8, 12, we will follow these steps:
Step 1: Calculate the Mean
The mean (average) is calculated using the formula:
Mean=1nn∑i=1xi
Where n is the number of observations and xi are the individual data points.
1. Count the number of data points:
- The data points are: 6, 7, 10, 12, 13, 4, 8, 12
- Total number of data points, n=8
2. Calculate the sum of the data points:
Sum=6+7+10+12+13+4+8+12=72
3. Calculate the mean:
Mean=728=9
Step 2: Calculate the Variance
Variance is calculated using the formula:
Variance=1nn∑i=1(xi−¯x)2
Where ¯x is the mean we calculated in Step 1.
1. Calculate (xi−¯x)2 for each data point:
- For x1=6: (6−9)2=(−3)2=9
- For x2=7: (7−9)2=(−2)2=4
- For x3=10: (10−9)2=(1)2=1
- For x4=12: (12−9)2=(3)2=9
- For x5=13: (13−9)2=(4)2=16
- For x6=4: (4−9)2=(−5)2=25
- For x7=8: (8−9)2=(−1)2=1
- For x8=12: (12−9)2=(3)2=9
2. Sum these squared differences:
Sum of squares=9+4+1+9+16+25+1+9=74
3. Calculate the variance:
Variance=748=9.25
Step 3: Calculate the Standard Deviation
Standard deviation is the square root of the variance:
Standard Deviation=√Variance=√9.25≈3.04
Final Results
- Mean: 9
- Variance: 9.25
- Standard Deviation: 3.04
- The data points are: 6, 7, 10, 12, 13, 4, 8, 12
- Total number of data points, n=8
Sum=6+7+10+12+13+4+8+12=72
Mean=728=9
- For x1=6: (6−9)2=(−3)2=9
- For x2=7: (7−9)2=(−2)2=4
- For x3=10: (10−9)2=(1)2=1
- For x4=12: (12−9)2=(3)2=9
- For x5=13: (13−9)2=(4)2=16
- For x6=4: (4−9)2=(−5)2=25
- For x7=8: (8−9)2=(−1)2=1
- For x8=12: (12−9)2=(3)2=9
Sum of squares=9+4+1+9+16+25+1+9=74
Variance=748=9.25
- Variance: 9.25
- Standard Deviation: 3.04
Topper's Solved these Questions
Similar Questions
Find the variance and standard deviation of the following data :
(i) 6,7,10,12,13,4,8,12
(ii) 5,12,3,18,6,8,2,10
(iii) 350,361, 370, 373, 376, 379, 385, 387, 394, 395
View SolutionFind the mean variance and standard deviation for the following data: 2,4,5,6,8,17
View SolutionFind the variance and standard deviation of the following data:

View SolutionFind the variance and standard deviation from the following data:

View SolutionFind the variance and standard deviation for the following data: 4,6,10,12,14,18,20
View SolutionFind the standard deviation for the following data

View SolutionFind the standard deviation for the following data
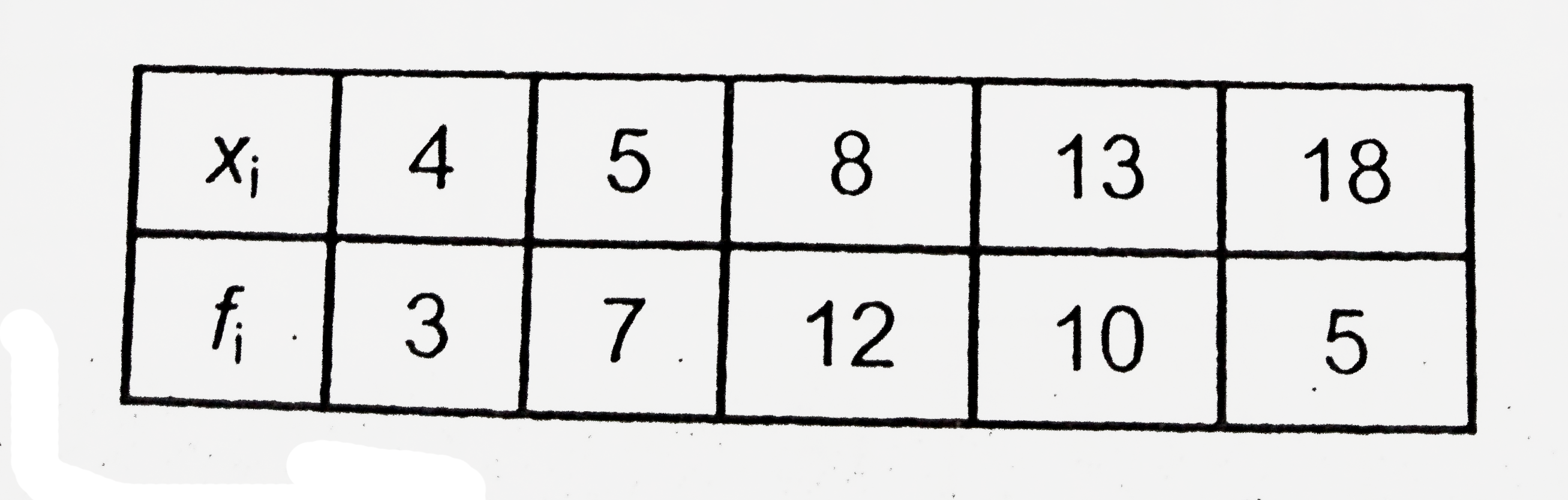
View SolutionCalculate the mean, variance and standard devation for the following data :
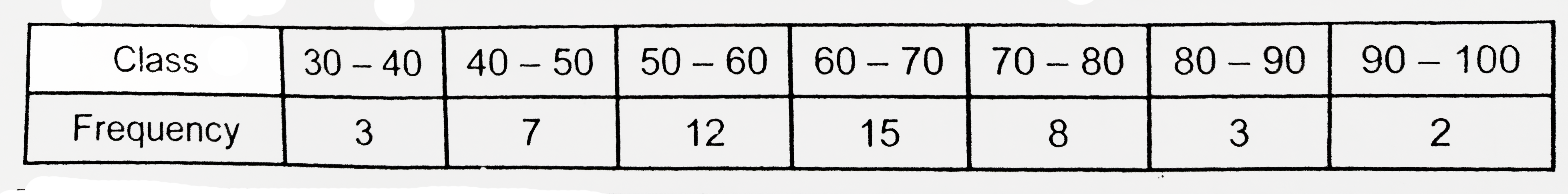
View SolutionFind the standard deviation from the following data:
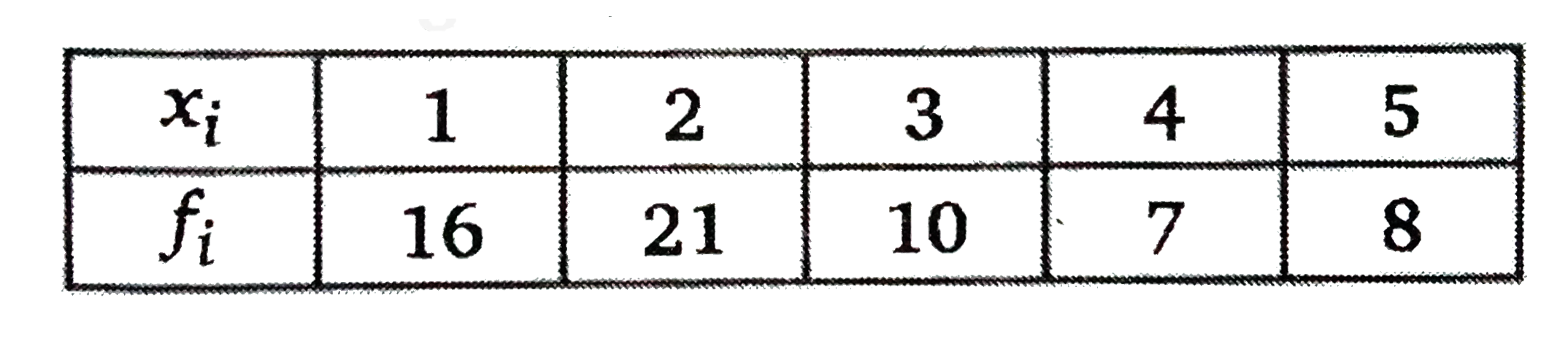
View SolutionFind the mean , mean deviation about the mean , variance and standard deviation for the following data
4,15,14,12,6,8,11
View Solution
