Find the minimum integral value of k for which the equation ex=kx2 has exactly three real distinct solutions.Solution in Bengali
Given equation is ex=kx2.
⇒k=exx2
Now let f(x)=exx2
∴f′(x)=(x−2)exx3
f′(x)=0∴x=2, which is the point of minima.
Also f(2)=e24
limx→∞exx2=∞ (using L' Hospital rule twice)
ex→∞xx2=0
limx→0exx2=∞
Further f(x)>0,∀x∈R.
From this information, the graph of f(x) is as shown in the following figure.
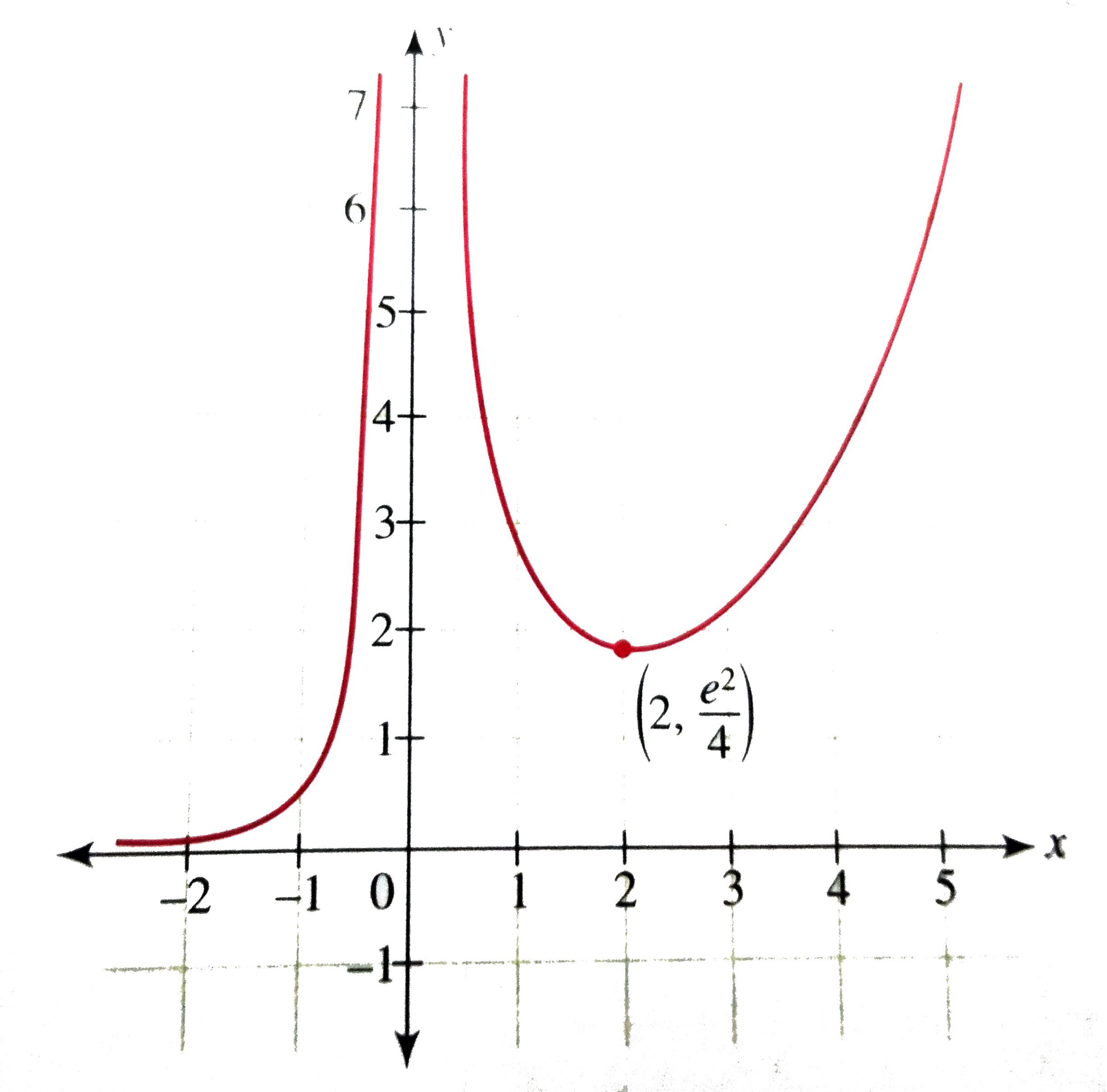
Thus, three real distinct solutions for k<e24,k∈I.
So, kmin=2.
Topper's Solved these Questions
Similar Questions
Find the derivative of y=sin2x−cosx
View SolutionLet →a and →b be two vectors of equal magnitude 5 units. Let →p,→q be vectors such that →p=→a−→b and →q=→a+→b. If |→p×→q|=2{λ−(→a.→b)2}12, then value of λ is
View SolutionLet f be a non-negative function defined on the interval [0,1]. If ∫x0√1−(f′(t))2dt=∫x0f(t)dt,0≤x≤1,and f(0)=0, then
View SolutionLet 'k' be a real number.The minimum number of distinct real roots possible of the equation (3x^(2)+kx+3)(x^(2)+kx-1)=0 is
View SolutionFind the values of a for which the equation x^(4)+(1-2a)x^(2)+a^(2)-1=0(A) has no real solutions (B) has one real solution (C) has two distinct real solutions (D) has three real solutions(E) has four distinct real solutions
View SolutionFind the value of K for which the quadratic equation kx^(2)+2x+1=0, has real and distinct root.
View SolutionFind the values of k for which the given quadratic equation has real and distinct roots: kx2+2x+1=0 (ii) kx2+6x+1=0 (iii) x2−kx+9=0
View SolutionFind the minimum integral value of k for which the equation ex=kx2 has exactly three real distinct solutions.
View SolutionIf the equation sin2x−ksinx−3=0 has exactly two distinct real roots in [0,π], then find the values of k .
View SolutionMinimum integral value of k for which the equation ex=kx2 has exactly three real distinct solution,
View SolutionIf the equation x4+kx2+k=0 has exactly two distinct real roots, then the smallest integral value of |k| is:
View SolutionFind the minimum integral value of k for which the equation ex=kx2 has exactly three real distinct solutions.
View SolutionIf the equation sin2x−ksinx−3=0 has exactly two distinct real roots in [0,π], then find the values of k .
View SolutionMinimum integral value of k for which the equation ex=kx2 has exactly three real distinct solution,
View SolutionLet 'k' be a real number. The minimum number of distinct real roots possible of the equation (3x2+kx+3)(x2+kx−1)=0 is
View SolutionIf the equation x4+kx2+k=0 has exactly two distinct real roots, then the smallest integral value of |k| is:
View Solution
