Find the number of distinct rational numbers x such that o<x<1andx=p/q , where p,q∈{1,2,3,4,5,6} .
As 0<x<1, we havep<q.

Thus, the number of rational number is 5+4+3+2+1=15.
When p and q have a common factor, we get some rational numbers, which are not different from those already counted. Here, there are four such numbers : 2/4,2/6,3/6,4/6. Therefore, the required number of rational numbers is 15-4=11.
Similar Questions
If f(x) is an even function then find the number of distinct real numbers x such that f(x)=f(x+1x+2).
View SolutionIf f(x) is an even function then find the number of distinct real numbers x such that f(x)=f(x+1x+2).
View SolutionFind rational numbers a and b such that .
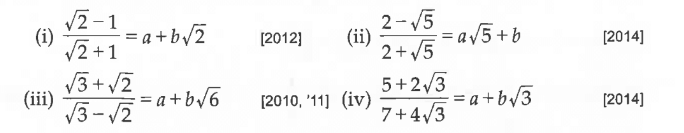
View SolutionIf A={x∣x is prime number and x<30}, find the number of different rational numbers whose numerator and denominator belong to A.
View SolutionIf A={x∣x is prime number and x<30}, find the number of different rational numbers whose numerator and denominator belong to A.
View SolutionFind the number of distinct real solutions of the equation f(f(f(x))=0, where f(x)=x2−1
View SolutionFind the number of distinct solution of secx+tanx=√3
View SolutionState true or false: (i) Between any two distinct integers there is always an integer. (ii) Between any two distinct rational numbers there is always a rational number. (iii) Between any two distinct rational numbers there are infinitely many rational numbers.
View SolutionFind the number of distinct terms in the expansion
(x+y−z)16
View SolutionFind the number of distinct terms in the expansion
(x+y+z)10
View Solution
