Find the ratio in which the line segment joining the points $(3, 10) a n d (6, 8) i s d i v i d e d b y (1, 6)$ .

Video Solution

Text Solution

Verified by Experts

Let the point $A (- 1, 6)$ divide the line joining $B (- 3, 10)$ and $C (6, - 8)$ in the ratio k:1. Then, the co-ordinates of A are $(\frac{6 k - 3}{k + 1}, \frac{- 8 k + 10}{k + 1}) \cdot [∵ internally ratio (\frac{m_{1} x_{2} + m_{2} x_{1}}{m_{1} + m_{2}}, \frac{m_{1} y_{2} + m_{2} y_{1}}{m_{1} + m_{2}})]$
But, the co-ordinates of A are given by (-1, 6).
$∴ \frac{6 k - 3}{k + 1} = - 1 and \frac{- 8 k + 10}{k + 1} = 6$

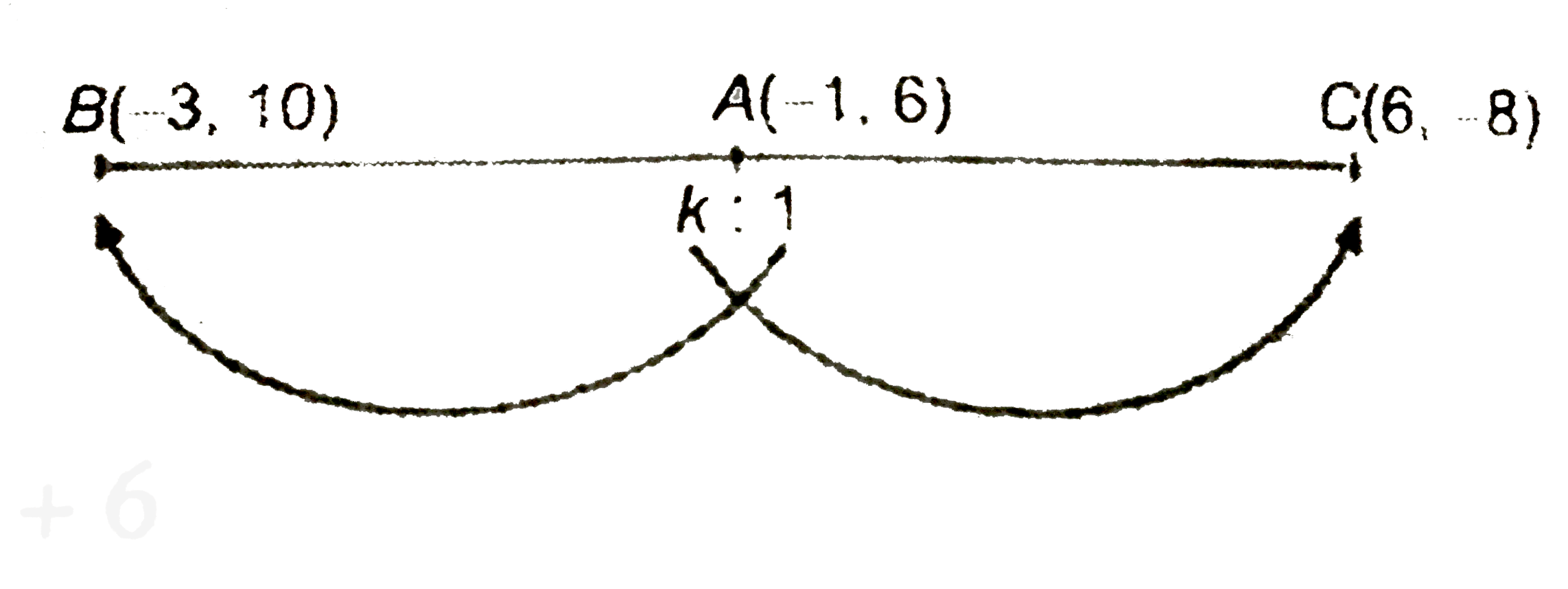
$\Rightarrow 6 k - 3 = - k - 1 and - 8 k + 10 = 6 k + 6$
$\Rightarrow 6 k + k = - 1 + 3 and - 8 k - 6 k = 6 - 10$
$\Rightarrow 7 k = 2 and - 14 k = - 4 \Rightarrow k = \frac{2}{7}$
So, the point A divides BC in the ratio 2 : 7.

|

Updated on:21/07/2023

Class 10 MATHS COORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The ratio in which the line segment joining the point A (4, 8, 10) and B (6, 10,- 8) is divided by yz - plane is
A2 : 3 internally
B2 : 3 externally
C3 : 2 internally
D3 : 2 externally

NAGEEN PRAKASHAN ENGLISH-CO-ORDINATE GEOMETRY-Problems Of NCERT/exemplar

Home
Ask Doubt
Profile