Find the ratio of the area of the semicircle formed on the diagonal of a square of side 14 cm to that of the semicircle forned on the side of the square.
The correct Answer is:2:1
To find the ratio of the area of the semicircle formed on the diagonal of a square of side 14 cm to that of the semicircle formed on the side of the square, we can follow these steps:
Step 1: Calculate the area of the semicircle on the side of the square.
- The side of the square is given as 14 cm.
- The diameter of the semicircle on the side is equal to the side of the square, which is 14 cm.
- The radius r of the semicircle is half of the diameter:
r=142=7 cm
- The area A of a semicircle is given by the formula:
A=12πr2
- Substituting the radius:
A=12π(72)=12π(49)=49π2 cm2
Step 2: Calculate the length of the diagonal of the square.
- The diagonal D of the square can be calculated using the Pythagorean theorem:
D=√(142+142)=√2×142=14√2 cm
Step 3: Calculate the area of the semicircle on the diagonal.
- The diameter of the semicircle on the diagonal is equal to the length of the diagonal, which is 14√2 cm.
- The radius r of this semicircle is:
r=14√22=7√2 cm
- The area A of the semicircle on the diagonal is:
A=12πr2=12π(7√2)2=12π(49×2)=98π2=49π cm2
Step 4: Find the ratio of the areas of the two semicircles.
- The area of the semicircle on the diagonal is 49π cm² and the area of the semicircle on the side is 49π2 cm².
- The ratio of the area of the semicircle on the diagonal to the area of the semicircle on the side is:
Ratio=49π49π2=49π×249π=2:1
Final Answer:
The ratio of the area of the semicircle formed on the diagonal of the square to that of the semicircle formed on the side of the square is 2:1.
---
- The side of the square is given as 14 cm.
- The diameter of the semicircle on the side is equal to the side of the square, which is 14 cm.
- The radius r of the semicircle is half of the diameter:
r=142=7 cm
- The area A of a semicircle is given by the formula:
A=12πr2
- Substituting the radius:
A=12π(72)=12π(49)=49π2 cm2
- The diagonal D of the square can be calculated using the Pythagorean theorem:
D=√(142+142)=√2×142=14√2 cm
- The diameter of the semicircle on the diagonal is equal to the length of the diagonal, which is 14√2 cm.
- The radius r of this semicircle is:
r=14√22=7√2 cm
- The area A of the semicircle on the diagonal is:
A=12πr2=12π(7√2)2=12π(49×2)=98π2=49π cm2
- The area of the semicircle on the diagonal is 49π cm² and the area of the semicircle on the side is 49π2 cm².
- The ratio of the area of the semicircle on the diagonal to the area of the semicircle on the side is:
Ratio=49π49π2=49π×249π=2:1
The ratio of the area of the semicircle formed on the diagonal of the square to that of the semicircle formed on the side of the square is 2:1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A circle is inscribed in a square of side 14 m. The ratio of the area of the circle and that of the square is ______.
Aπ:3Bπ:4Cπ:2Dπ:1 - Question 2 - Select One
If the length of a diagonal of a square is 8 cm. Find the length of the side of the square.
A2 cmB2.8 cmC1.414 cmD5.64 cm
Find the area of a square whose side is 5cm.
View SolutionIf the diagonal of a square is 13 cm ,then find the length of its side .
View SolutionLength of the diagonal of a square is 12 cm. Find the length of each side of the square.
View SolutionFind the area of the shaded region in the given figure, if ABCD is a square of side 14 cm and APD and BPC are semicircles.
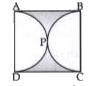 View Solution
View SolutionFind the perimeter of the shaded region in the figure,if ABCD is a square of side 14 cm and APB and CPD are semicircles.
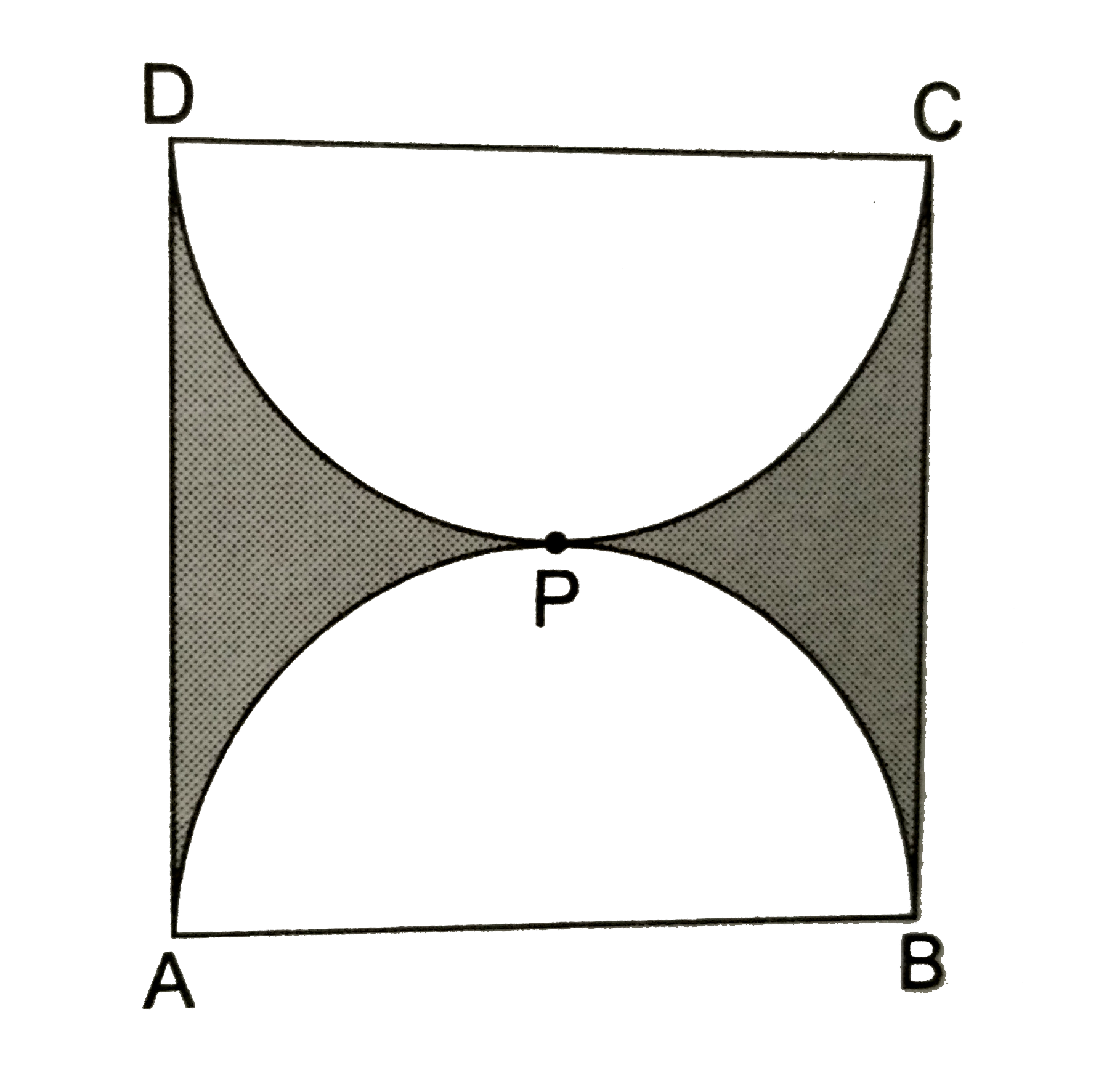 View Solution
View SolutionFind the ratio of area of square and area of square taking diagonal as side.
View Solution
