Find the reference angles corresponding to each of the following angles. It may help if you sketch θ in standard position. θ=−230∘ (ii)31π9 (iii)θ=640∘
To find the reference angles for the given angles, we will follow a systematic approach. The reference angle is defined as the smallest angle that the terminal side of the given angle makes with the x-axis.
3. Calculate the reference angle:
The reference angle in the second quadrant is given by:
Reference Angle=180°−θ
Substituting the value:
Reference Angle=180°−130°=50°
Answer: The reference angle for θ = -230° is 50°.
---
(ii) For θ = (31π)/9:
1. Convert the angle to degrees (optional):
To understand the position of the angle, we can convert it to degrees.
Degrees=(31π9)×(180°π)=31×180°9=620°
2. Find the equivalent angle within 0° to 360°:
Since 620° is greater than 360°, we subtract 360°:
620°−360°=260°
3. Determine the quadrant:
The angle 260° is in the third quadrant.
4. Calculate the reference angle:
The reference angle in the third quadrant is given by:
Reference Angle=θ−180°
Substituting the value:
Reference Angle=260°−180°=80°
Answer: The reference angle for θ = (31π)/9 is 80°.
---
(iii) For θ = 640°:
1. Find the equivalent angle within 0° to 360°:
Since 640° is greater than 360°, we subtract 360°:
640°−360°=280°
2. Determine the quadrant:
The angle 280° is in the fourth quadrant.
3. Calculate the reference angle:
The reference angle in the fourth quadrant is given by:
Reference Angle=360°−θ
Substituting the value:
Reference Angle=360°−280°=80°
Answer: The reference angle for θ = 640° is 80°.
---
Summary of Reference Angles:
1. For θ = -230°, the reference angle is 50°.
2. For θ = (31π)/9, the reference angle is 80°.
3. For θ = 640°, the reference angle is 80°.
The reference angle in the second quadrant is given by:
Reference Angle=180°−θ
Substituting the value:
Reference Angle=180°−130°=50°
1. Convert the angle to degrees (optional):
To understand the position of the angle, we can convert it to degrees.
Degrees=(31π9)×(180°π)=31×180°9=620°
Since 620° is greater than 360°, we subtract 360°:
620°−360°=260°
The angle 260° is in the third quadrant.
The reference angle in the third quadrant is given by:
Reference Angle=θ−180°
Substituting the value:
Reference Angle=260°−180°=80°
1. Find the equivalent angle within 0° to 360°:
Since 640° is greater than 360°, we subtract 360°:
640°−360°=280°
The angle 280° is in the fourth quadrant.
The reference angle in the fourth quadrant is given by:
Reference Angle=360°−θ
Substituting the value:
Reference Angle=360°−280°=80°
1. For θ = -230°, the reference angle is 50°.
2. For θ = (31π)/9, the reference angle is 80°.
3. For θ = 640°, the reference angle is 80°.
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - example
10 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Concept Application Exercises 2.1
8 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Videos
Similar Questions
Knowledge Check
In the figure, which one of the following is the measure of angle θ?
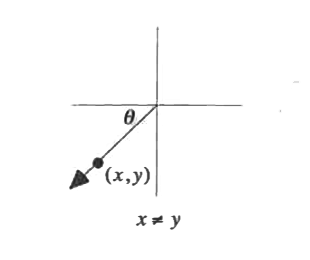 Aθ<45∘Bθ>45∘Cθ=45∘DIt cannot be determined from the information given
Aθ<45∘Bθ>45∘Cθ=45∘DIt cannot be determined from the information given
Evaluate the following limits :
Limθ→π2(secθ−tanθ)
View SolutionFind the general values of θ from the following equations:
tan(π4+θ)+tan(π4−θ)=4
View SolutionFind the general solutions of the following equations: cos3θ=0 (ii) cos(3θ)2=0 (iii) cos23θ=0
View SolutionFind the value of θ in each of the following: (i)2sin2θ=√3 (ii)2cos3θ=1 (iii) √3tan2θ−3=0
View SolutionDerive formulas for the following in terms of functions of 2θand and then of θ.
(i)sin4θ
(ii) cos4θ,
(iii) tan4θ
View SolutionFind the value of θ in each of the following: (i)2sin2θ=√3 (ii)2cos3θ=1
View SolutionFind the general solutions of the following equations: sin2θ=0 (ii) sin(3θ2)=0 (iii) sin22θ=0
View Solution
