Find the sum of first 24 terms of on AP t1,t2,t3,...., if it is known that t1+t5+t10+t15+t20+t24=225.
To find the sum of the first 24 terms of an arithmetic progression (AP) given the condition t1+t5+t10+t15+t20+t24=225, we can follow these steps:
Step 1: Define the terms of the AP
Let the first term of the AP be a and the common difference be d. The n-th term of the AP can be expressed as:
tn=a+(n−1)d
Step 2: Write the terms based on the given condition
From the problem, we need to find:
t1+t5+t10+t15+t20+t24
Substituting the formula for the n-th term:
t1=a
t5=a+4d
t10=a+9d
t15=a+14d
t20=a+19d
t24=a+23d
Step 3: Combine the terms
Now, we can combine these terms:
t1+t5+t10+t15+t20+t24=a+(a+4d)+(a+9d)+(a+14d)+(a+19d)+(a+23d)
This simplifies to:
6a+(4+9+14+19+23)d
Calculating the sum of the coefficients of d:
4+9+14+19+23=69
So we have:
6a+69d=225
Step 4: Simplify the equation
We can simplify this equation:
6a+69d=225
Dividing the entire equation by 3 gives:
2a+23d=75(Equation 1)
Step 5: Find the sum of the first 24 terms
The formula for the sum of the first n terms of an AP is:
Sn=n2×(2a+(n−1)d)
For the first 24 terms:
S24=242×(2a+23d)=12×(2a+23d)
Substituting Equation 1 into this formula:
S24=12×75=900
Final Answer
The sum of the first 24 terms of the AP is:
900
Let the first term of the AP be a and the common difference be d. The n-th term of the AP can be expressed as:
tn=a+(n−1)d
From the problem, we need to find:
t1+t5+t10+t15+t20+t24
Substituting the formula for the n-th term:
t1=a
t5=a+4d
t10=a+9d
t15=a+14d
t20=a+19d
t24=a+23d
Now, we can combine these terms:
t1+t5+t10+t15+t20+t24=a+(a+4d)+(a+9d)+(a+14d)+(a+19d)+(a+23d)
This simplifies to:
6a+(4+9+14+19+23)d
Calculating the sum of the coefficients of d:
4+9+14+19+23=69
So we have:
6a+69d=225
We can simplify this equation:
6a+69d=225
Dividing the entire equation by 3 gives:
2a+23d=75(Equation 1)
The formula for the sum of the first n terms of an AP is:
Sn=n2×(2a+(n−1)d)
For the first 24 terms:
S24=242×(2a+23d)=12×(2a+23d)
Substituting Equation 1 into this formula:
S24=12×75=900
The sum of the first 24 terms of the AP is:
900
Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise For Session 1
5 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise For Session 2
11 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
Similar Questions
Determine the sum of first 35 terms of an A.P. if t2, = 1 and t7 , = -22.
View SolutionStatement :1 If a parabola y2=4ax intersects a circle in three co-normal points then the circle also passes through the vertex of the parabola. Because
Statement : 2 If the parabola intersects circle in four points t1,t2,t3andt4 then t1+t2+t3+t4=0 and for co-normal points t1,t2,t3 we have t1+t2+t3=0.
View Solution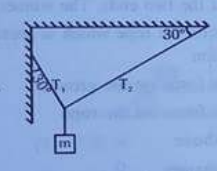
Calculate T1&T2.
View SolutionFind the locus of the point (t2−t+1,t2+t+1),t∈R.
View SolutionLet s1,s2,s3....andt1,t2,t3.... are two arithmetic sequences such that s1=t1≠0,s2=2t2and10∑i=1si=15∑i=1ti. Then the value of s2−s1t2−t1 is
View SolutionFind the locus of the point (t2−t+1,t2+t+1),t∈R.
View SolutionIn a harmonic progression t1,t2,t3,……………., it is given that t5=20andt6=50. If Sn denotes the sum of first n terms of this, then the value of n for which Sn is maximum is
View SolutionSuppose a series of n terms given by Sn=t1+t2+t3+. . . .+tn
then Sn−1=t1+t2+t3+. . . .+tn−1,n≥1 subtracting we get Sn−Sn−1=tn,n≥2 surther if we put n=1 is the first sum then S1=t1 thus w can write tn=Sn−Sn−1,n≥2 and t1=S1
Q. The sum of n terms of a series is a.2n−b. where a and b are constant then the series is
View SolutionSuppose a series of n terms given by Sn=t1+t2+t3+. . ..+tn
then Sn−1=t1+t2+t3+ . . . +tn−1,n≥1 subtracting we get Sn−Sn−1=tn,n≥2 surther if we put n=1 is the first sum then S1=t1 thus w can write tn=Sn−Sn−1,n≥2 and t1=S1
Q. if the sum of n terms of a series a.2n−b then the sum ∞∑r=11tr is
View Solution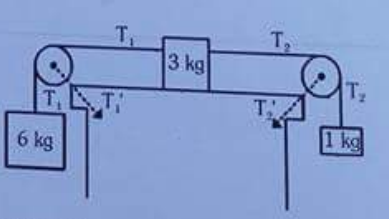
Calculate a, T1,T2,T1'&T2'.
View Solution
