Question

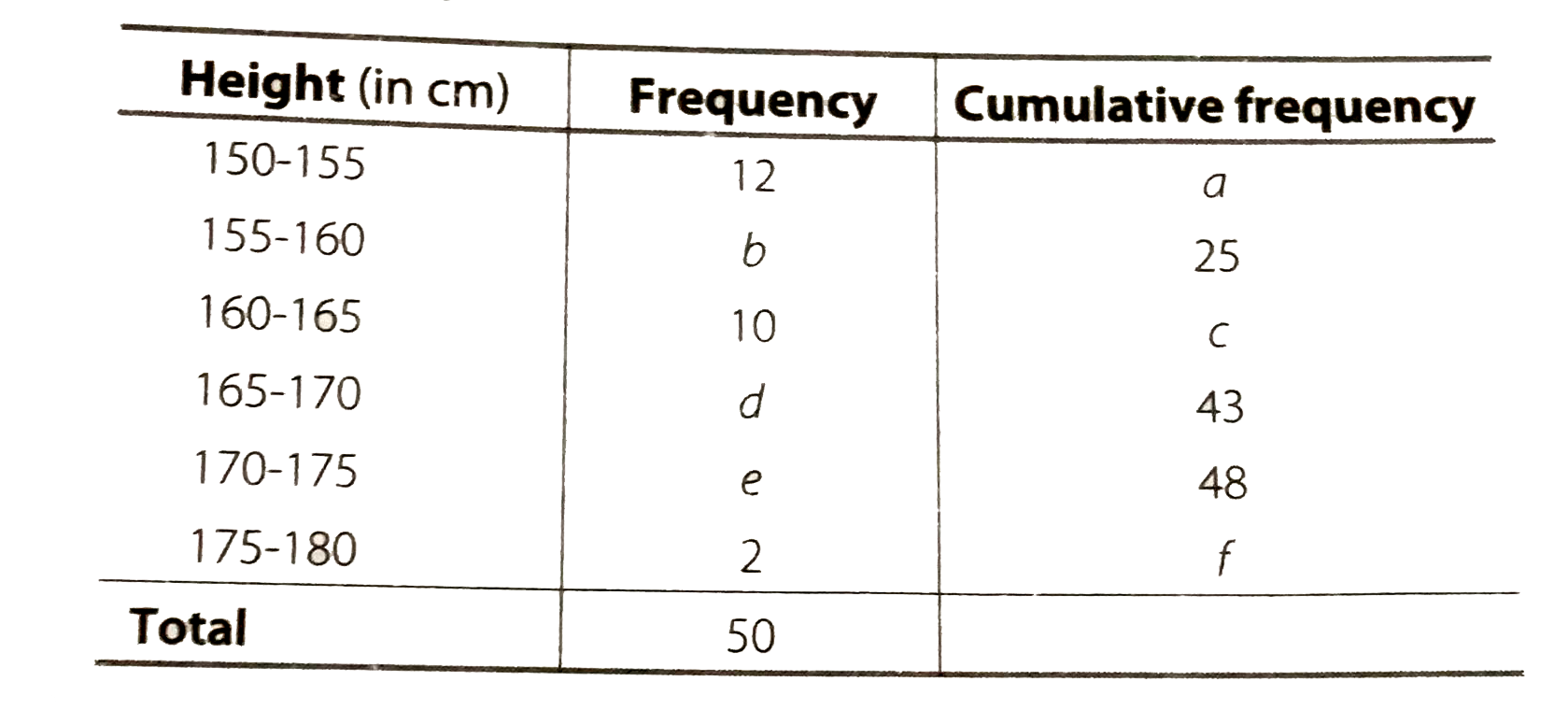
Find the unknown entries (a,b,c,d,e,f,g)
from the following frequency distribution of heights of 50 students in a
class:
Class intervals
(heights in
cm)              Frequency                                      Cumulative
Frequency
150-155
155-160
160-165
165-170
170-175
175-180           12
b
10
d
e
2          a
25
c
43
48
f
                            

Explanation

To find the unknown entries (a, b, c, d, e, f, g) in the frequency distribution of heights of 50 students, we will follow these steps:

Step 1: Identify the first cumulative frequency (a)
The first cumulative frequency is equal to the frequency of the first class interval.
- For the class interval 150-155, the frequency is 12.
- Therefore, \( a = 12 \).

Step 2: Calculate the second cumulative frequency (b)
The second cumulative frequency is the sum of the first cumulative frequency and the frequency of the second class interval.
- The first cumulative frequency \( a = 12 \) and the frequency for the class interval 155-160 is \( b \).
- The cumulative frequency for this interval is given as 25.
- Hence, \( a + b = 25 \) or \( 12 + b = 25 \).
- Solving for \( b \):
\( b = 25 - 12 = 13 \)

Step 3: Calculate the third cumulative frequency (c)
The third cumulative frequency is the sum of the second cumulative frequency and the frequency of the third class interval.
- The second cumulative frequency is \( 25 \) and the frequency for the class interval 160-165 is \( 10 \).
- Therefore, \( c = 25 + 10 = 35 \).

Step 4: Calculate the fourth cumulative frequency (d)
The fourth cumulative frequency is the sum of the third cumulative frequency and the frequency of the fourth class interval.
- The third cumulative frequency \( c = 35 \) and the frequency for the class interval 165-170 is \( d \).
- The cumulative frequency for this interval is given as 43.
- Thus, \( c + d = 43 \) or \( 35 + d = 43 \).
- Solving for \( d \):
\( d = 43 - 35 = 8 \)

Step 5: Calculate the fifth cumulative frequency (e)
The fifth cumulative frequency is the sum of the fourth cumulative frequency and the frequency of the fifth class interval.
- The fourth cumulative frequency \( d = 43 \) and the frequency for the class interval 170-175 is \( e \).
- The cumulative frequency for this interval is given as 48.
- Hence, \( d + e = 48 \) or \( 43 + e = 48 \).
- Solving for \( e \):
\( e = 48 - 43 = 5 \)

Step 6: Calculate the sixth cumulative frequency (f)
The sixth cumulative frequency is the sum of the fifth cumulative frequency and the frequency of the sixth class interval.
- The fifth cumulative frequency \( e = 48 \) and the frequency for the class interval 175-180 is \( 2 \).
- Therefore, \( f = 48 + 2 = 50 \).

Step 7: Determine the total cumulative frequency (g)
The total cumulative frequency for all class intervals should equal the total number of students, which is 50.
- Therefore, \( g = 50 \).

Summary of Values
- \( a = 12 \)
- \( b = 13 \)
- \( c = 35 \)
- \( d = 8 \)
- \( e = 5 \)
- \( f = 50 \)
- \( g = 50 \)