Find the value of (71)3 using the short-cut method.
To find the value of 713 using the shortcut method, we can express 71 as 70+1. We will then apply the formula for the cube of a binomial, which is:
(a+b)3=a3+b3+3a2b+3ab2
1. Identify a and b:
- Let a=70 and b=1.
2. Apply the formula:
(70+1)3=703+13+3(702)(1)+3(70)(12)
3. Calculate 703:
- 703=70×70×70=343000 (since 73=343 and we add three zeros).
4. Calculate 13:
- 13=1.
5. Calculate 3(702)(1):
- First, calculate 702=4900.
- Then, 3(702)(1)=3×4900=14700.
6. Calculate 3(70)(12):
- Since 12=1, we have 3(70)(1)=3×70=210.
7. Combine all the parts:
713=343000+1+14700+210
8. Add the values together:
- First, add 343000+1=343001.
- Then, add 343001+14700=357701.
- Finally, add 357701+210=357911.
Thus, the value of 713 is 357911.
- Let a=70 and b=1.
(70+1)3=703+13+3(702)(1)+3(70)(12)
- 703=70×70×70=343000 (since 73=343 and we add three zeros).
- 13=1.
- First, calculate 702=4900.
- Then, 3(702)(1)=3×4900=14700.
- Since 12=1, we have 3(70)(1)=3×70=210.
713=343000+1+14700+210
- First, add 343000+1=343001.
- Then, add 343001+14700=357701.
- Finally, add 357701+210=357911.
Topper's Solved these Questions
- CUBES AND CUBE ROOTSBOOK - RS AGGARWALCHAPTER - CUBES AND CUBE ROOTSEXERCISE - Exercise 4A
10 Videos
- CUBES AND CUBE ROOTSBOOK - RS AGGARWALCHAPTER - CUBES AND CUBE ROOTSEXERCISE - Exercise 4B
4 Videos
- CONSTRUCTION OF QUADRILATERALS BOOK - RS AGGARWALCHAPTER - CONSTRUCTION OF QUADRILATERALS EXERCISE - Test Paper-17 (E)
1 Video
- DATA HANDLING BOOK - RS AGGARWALCHAPTER - DATA HANDLING EXERCISE - Exercise 21C
11 Videos
Similar Questions
Knowledge Check
cut short
AdeleteBpraiseCinterruptDslice into small piecesFind the value of:
(47)3A103822B103823C103824D103825Find the value using the short-cut method:
(25)3A15625B15635C15645D15655
find the value of (29)3 by short cut method
View SolutionFind the AM of the following data by short-cut method.
Class Intervalf 1−55 6−1010 11−1515 16−2010 21−255
View SolutionFind the cube of 243 by using column method.
View SolutionThe mean of the following distribution is 25 . Find the value of p using direct method :
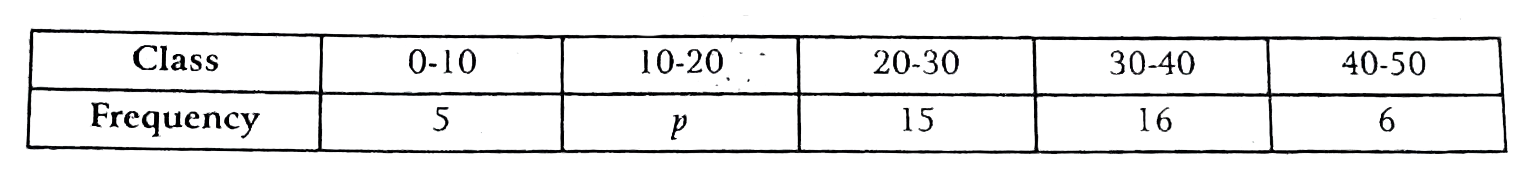
View SolutionThe mean of the following distribution is 54. Find the value of p using direct method :
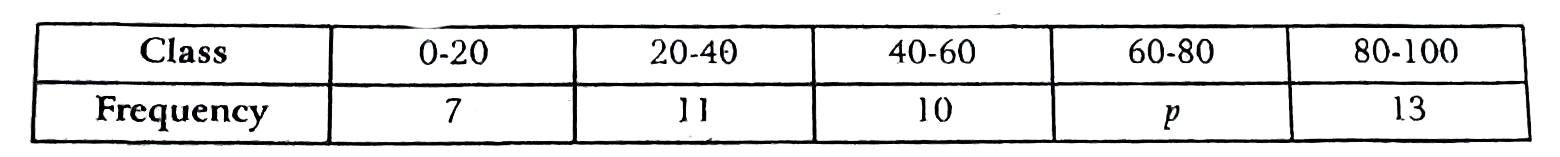
View Solution