For any real number x, let $[x]$ = largest integer less than or equalto x. Let f be a real valued function defined on the interval $[- 10, 10]$ by
Then, the value of ${(\frac{π}{10})}^{2} (\int_{- 10}^{10} f (x) \cos π x d x$ is

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Answer

Step by step video, text & image solution for For any real number x, let [x]= largest integer less than or equalto x. Let f be a real valued function defined on the interval[-10,10] by Then, the value of (pi/10)^2 (int_-10^10 f(x) cos pi x dx is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Knowledge Check

For any real number x, let $[x]$ = largest integer less than or equalto x. Let f be a real valued function defined on the interval $[- 10, 10]$ by
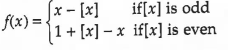Then, the value of ${(\frac{π}{10})}^{2} (\int_{- 10}^{10} f (x) cos π x d x$ is
A4
B5
C6
D1
For any real number x, let [x] denotes the largest integer less than or equal to x.Let f be a real valued function defined on the interval[-10,10] by
$f (x) = {(x - [x]) < b r > (1 + [x] - x)}$
Then, the value of $\frac{π^{2}}{10} \int_{- 10}^{10}$ f(x) cos $π$ x dx is
A2
B4
C0
DNone of these
For a real number x let [x] denote the largest number less than or equal to x. For $x \in R$ let f (x)=[x] $sin π x$ . Then
AIf is differentiable on R.
Bf is symmetric about the line x=0
C $\int_{- 3}^{3} f (x) d x = 0$
DFor each real $α$ , the equation $f (x) - α = 0$ has infinitely many roots.

ML KHANNA-AREA OF CURVES-SELF ASSESSEMENT TEST

Home
Ask Doubt
Profile

For any real number x, let [ x ] = largest integer less than or equalto x. Let f be a real valued function defined on the interval [ - 10 , 10 ] by Then, the value of ( π 10 ) 2 ( ∫ - 10 10 f ( x ) cos π x d x is

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

For any real number x, let [x]= largest integer less than or equalto x. Let f be a real valued function defined on the interval[−10,10] by Then, the value of (π10)2(∫10−10f(x)cosπxdx is

For any real number x, let [x] denotes the largest integer less than or equal to x.Let f be a real valued function defined on the interval[-10,10] by f(x)={(x−[x])<br>(1+[x]−x)} Then, the value of π210∫10−10 f(x) cos π x dx is

For a real number x let [x] denote the largest number less than or equal to x. For x∈R let f (x)=[x] sinπx. Then

ML KHANNA-AREA OF CURVES-SELF ASSESSEMENT TEST

For any real number x, let $[x]$ = largest integer less than or equalto x. Let f be a real valued function defined on the interval $[- 10, 10]$ by
Then, the value of ${(\frac{π}{10})}^{2} (\int_{- 10}^{10} f (x) \cos π x d x$ is

For any real number x, let $[x]$ = largest integer less than or equalto x. Let f be a real valued function defined on the interval $[- 10, 10]$ by
Then, the value of ${(\frac{π}{10})}^{2} (\int_{- 10}^{10} f (x) cos π x d x$ is

For any real number x, let [x] denotes the largest integer less than or equal to x.Let f be a real valued function defined on the interval[-10,10] by
$f (x) = {(x - [x]) < b r > (1 + [x] - x)}$
Then, the value of $\frac{π^{2}}{10} \int_{- 10}^{10}$ f(x) cos $π$ x dx is

For a real number x let [x] denote the largest number less than or equal to x. For $x \in R$ let f (x)=[x] $sin π x$ . Then