For the following, calculate mean deviation and coefficient of mean deviation.
3, 9, 5, 3, 12, 10, 18, 4, 7, 19, 21
The correct Answer is:M.D = 5.27, coefft. of M.D = 0.59
To calculate the mean deviation and the coefficient of mean deviation for the given data set 3,9,5,3,12,10,18,4,7,19,21, we can follow these steps:
Step 1: Calculate the Mean
The mean (average) is calculated using the formula:
Mean(A)=Σxn
where Σx is the sum of all data points and n is the number of data points.
1. Sum the data points:
Σx=3+9+5+3+12+10+18+4+7+19+21=111
2. Count the number of data points:
n=11
3. Calculate the mean:
A=11111=10.09
Step 2: Calculate the Deviation from the Mean
The deviation for each data point is calculated as:
D=|x−A|
where x is each individual data point.
1. Calculate the absolute deviations:
- For 3: |3−10.09|=7.09
- For 9: |9−10.09|=1.09
- For 5: |5−10.09|=5.09
- For 3: |3−10.09|=7.09
- For 12: |12−10.09|=1.91
- For 10: |10−10.09|=0.09
- For 18: |18−10.09|=7.91
- For 4: |4−10.09|=6.09
- For 7: |7−10.09|=3.09
- For 19: |19−10.09|=8.91
- For 21: |21−10.09|=10.91
Step 3: Calculate the Mean Deviation
The mean deviation is calculated using the formula:
Mean Deviation=ΣDn
where ΣD is the sum of the absolute deviations.
1. Sum the absolute deviations:
ΣD=7.09+1.09+5.09+7.09+1.91+0.09+7.91+6.09+3.09+8.91+10.91=59.27
2. Calculate the mean deviation:
Mean Deviation=59.2711≈5.39
Step 4: Calculate the Coefficient of Mean Deviation
The coefficient of mean deviation is calculated using the formula:
Coefficient of Mean Deviation=Mean DeviationMean
1. Calculate the coefficient:
Coefficient of Mean Deviation=5.3910.09≈0.53
Final Results:
- Mean Deviation: ≈5.39
- Coefficient of Mean Deviation: ≈0.53
The mean (average) is calculated using the formula:
Mean(A)=Σxn
where Σx is the sum of all data points and n is the number of data points.
Σx=3+9+5+3+12+10+18+4+7+19+21=111
2. Count the number of data points:
n=11
3. Calculate the mean:
A=11111=10.09
The deviation for each data point is calculated as:
D=|x−A|
where x is each individual data point.
- For 3: |3−10.09|=7.09
- For 9: |9−10.09|=1.09
- For 5: |5−10.09|=5.09
- For 3: |3−10.09|=7.09
- For 12: |12−10.09|=1.91
- For 10: |10−10.09|=0.09
- For 18: |18−10.09|=7.91
- For 4: |4−10.09|=6.09
- For 7: |7−10.09|=3.09
- For 19: |19−10.09|=8.91
- For 21: |21−10.09|=10.91
The mean deviation is calculated using the formula:
Mean Deviation=ΣDn
where ΣD is the sum of the absolute deviations.
ΣD=7.09+1.09+5.09+7.09+1.91+0.09+7.91+6.09+3.09+8.91+10.91=59.27
2. Calculate the mean deviation:
Mean Deviation=59.2711≈5.39
The coefficient of mean deviation is calculated using the formula:
Coefficient of Mean Deviation=Mean DeviationMean
1. Calculate the coefficient:
Coefficient of Mean Deviation=5.3910.09≈0.53
- Mean Deviation: ≈5.39
- Coefficient of Mean Deviation: ≈0.53
Topper's Solved these Questions
- MEASURES OF DISPERSIONBOOK - ICSECHAPTER - MEASURES OF DISPERSIONEXERCISE - EXERCISE 21 (b)
19 Videos
- MEASURES OF DISPERSIONBOOK - ICSECHAPTER - MEASURES OF DISPERSIONEXERCISE - CHAPTER TEST
6 Videos
- MEASURES OF DISPERSIONBOOK - ICSECHAPTER - MEASURES OF DISPERSIONEXERCISE - CHAPTER TEST
6 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - EXERCISE
14 Videos
- MOCK TEST PAPER-2021BOOK - ICSECHAPTER - MOCK TEST PAPER-2021EXERCISE - SECTION - C
10 Videos
Similar Questions
For the following, using median, calculate mean deviation and coefficient of mean deviation.

View SolutionFor the following, using median, calculate mean deviation and coefficient of mean deviation.
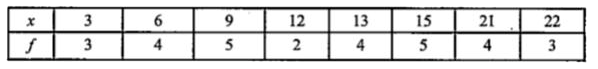
View SolutionFor the following, using median, calculate mean deviation and coefficient of mean deviation.
100, 150, 200, 250, 360, 490, 500, 600, 671
View SolutionFrom the following data, using mean, calculate mean deviation and the coefficient of mean deviation.
15, 17, 19, 25, 30, 35, 48
View SolutionFrom the following data, using mean, calculate mean deviation and the coefficient of mean deviation.
21, 23, 25, 28, 30, 32, 38, 39, 46, 48
View SolutionFrom the following data, using mean, calculate mean deviation and the coefficient of mean deviation.
10, 70, 50, 53, 20, 95, 42, 60, 48, 80
View SolutionFind the mean deviation about the median for the following data:3,9,5,3,12,10,18,4,7,19,21.
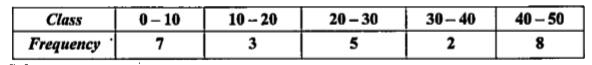
View SolutionCalculate the mean deviation and coefficient of mean deviation for the following distribution:
View SolutionCalculate the mean deviation about mean of the following data.(i) 1, 4, 7, 8, 9,10, 12, 13, 17
View SolutionFind the mean deviation about the mean for the data : 4,7,8,9,10,12,13,17
View Solution
