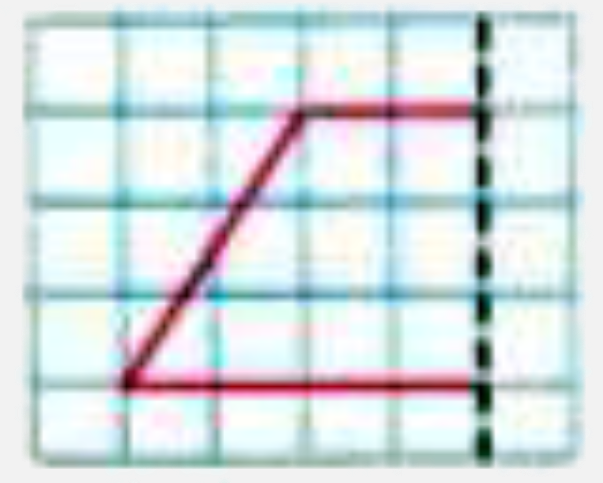
For the given figure, which one is the mirror line, ?
Mirror Line is a line that cuts the figure into symmetrical parts.
If there exists at least one line that divides a figure into two halves such that
one-half is the mirror image of the other half, it is known as reflection
symmetry.
In the following figure, l2 is
the mirror line as it is dividing the figure into two
exactly symmetrical figures
Topper's Solved these Questions
- SYMMETRYBOOK - NCERT ENGLISHCHAPTER - SYMMETRYEXERCISE - EXERCISE 13.2
6 Videos
- SYMMETRYBOOK - NCERT ENGLISHCHAPTER - SYMMETRYEXERCISE - EXERCISE 13.2
6 Videos
- RATIO AND PROPORTIONBOOK - NCERT ENGLISHCHAPTER - RATIO AND PROPORTIONEXERCISE - EXERCISE 12.1
16 Videos
- UNDERSTANDING ELEMENTARY SHAPESBOOK - NCERT ENGLISHCHAPTER - UNDERSTANDING ELEMENTARY SHAPESEXERCISE - EXERCISE 5.5
4 Videos
Similar Questions
Knowledge Check
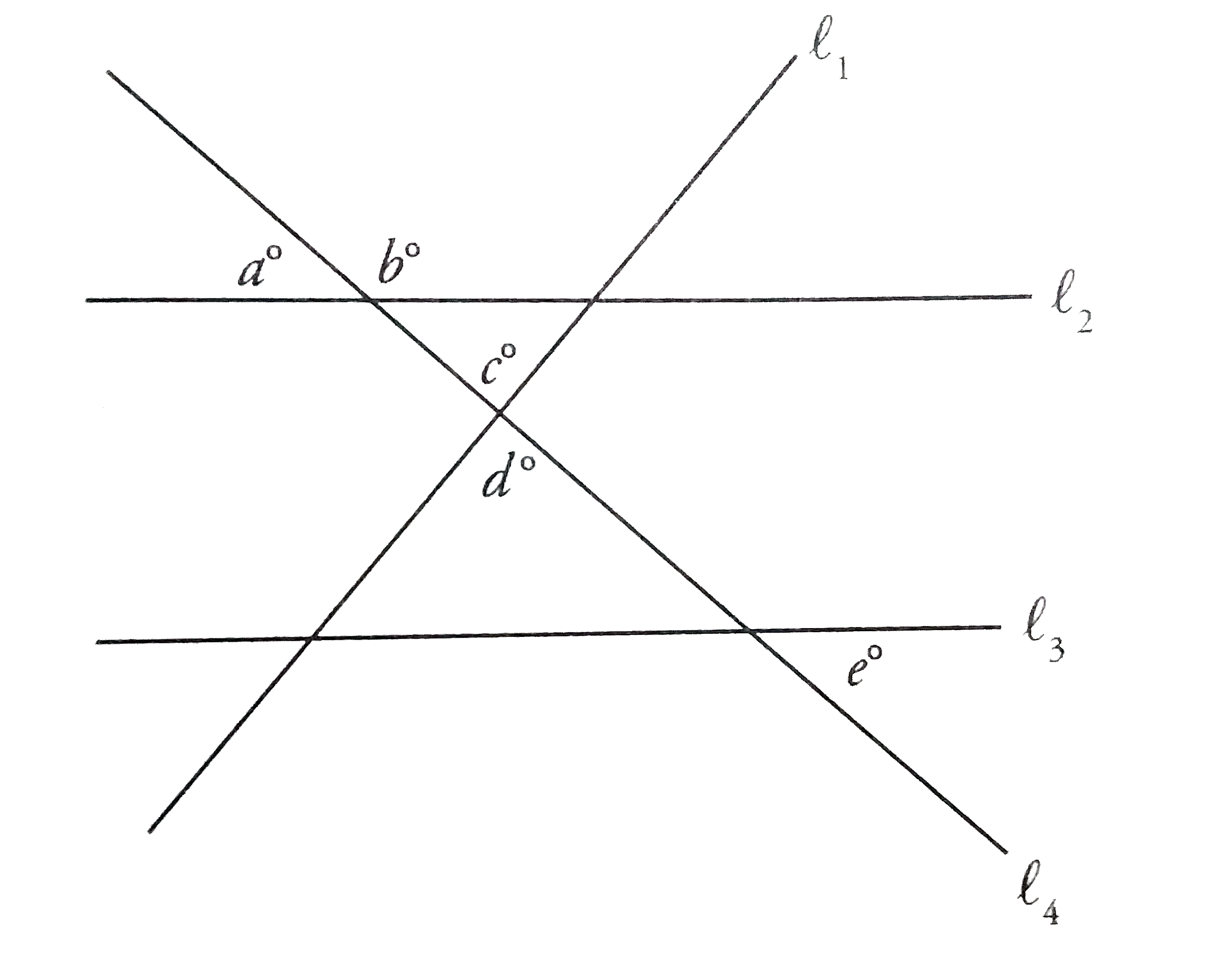
In the figure above l2∣∣l3, which of the following could be false?Aa=eBb+e=180Cl1⊥l4Dc=dIn the figure, lines l and k are parallel. If a is an acute angle, then which one of the following must be true?
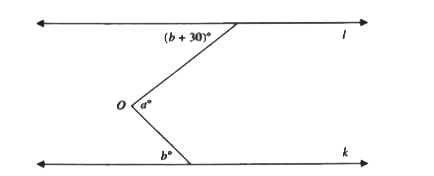 Ab>10Bb>15Cb<20Db<30
Ab>10Bb>15Cb<20Db<30
In the given figure, a transversal n cuts two lines l and m such that ∠2=650 and ∠8=650. Are the lines parallel?
View SolutionIn the given figure, Given equation of line L1 is y = 4.
Write the slope of line L2 if L2 is the bisector of angle O.
View SolutionIf L1 is the line of intersection of the planes 2x−2y+3z−2=0x−y+z+1=0 and L2 is the line of the intersection of the planes x+2y−z−3=0 3x−y+2z−1=0then the distance of the origin from the plane containing the lines L1 and L2 is
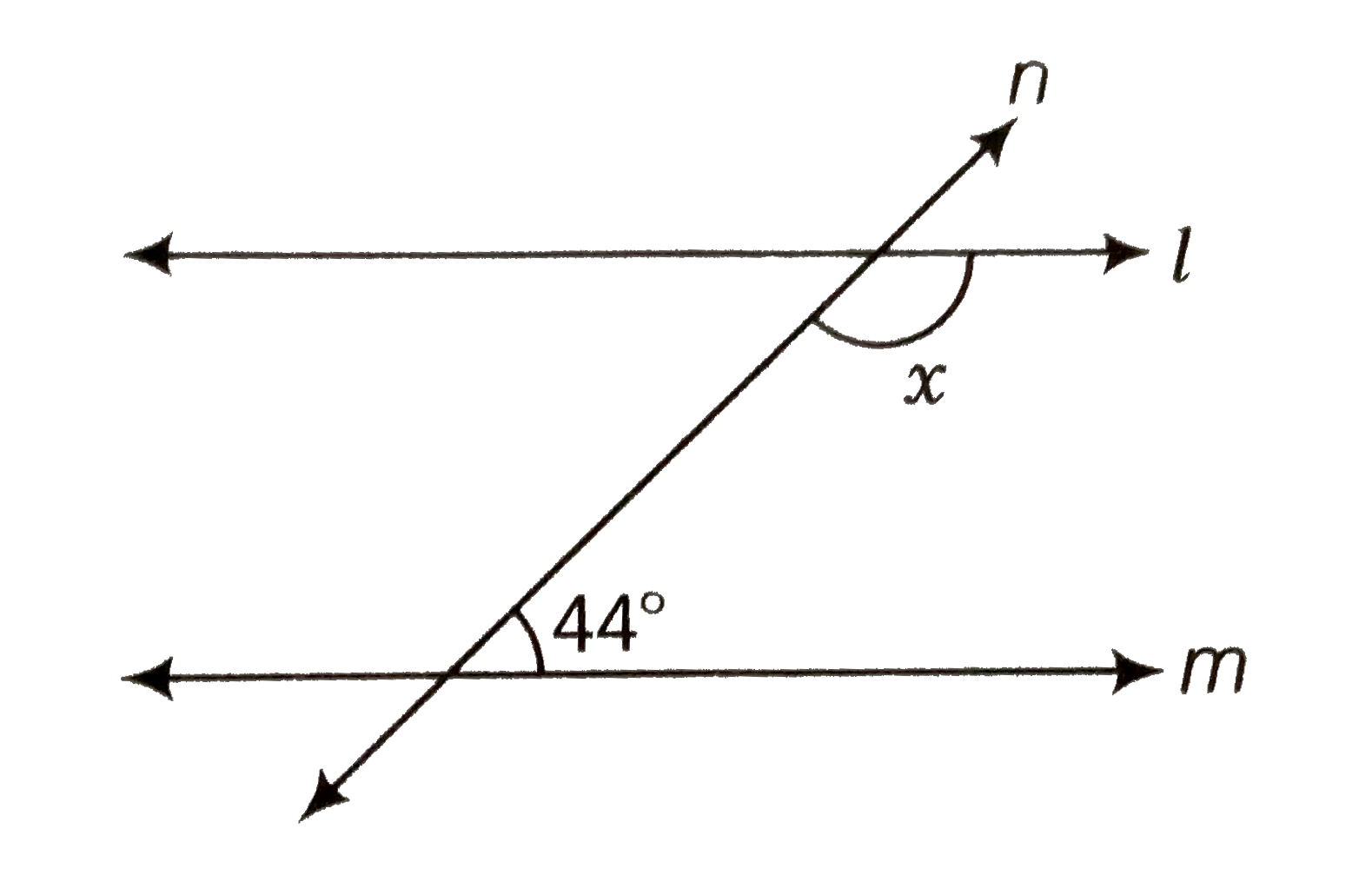
View SolutionIn the figure, find the value of x for which the lines l and m are parallel.
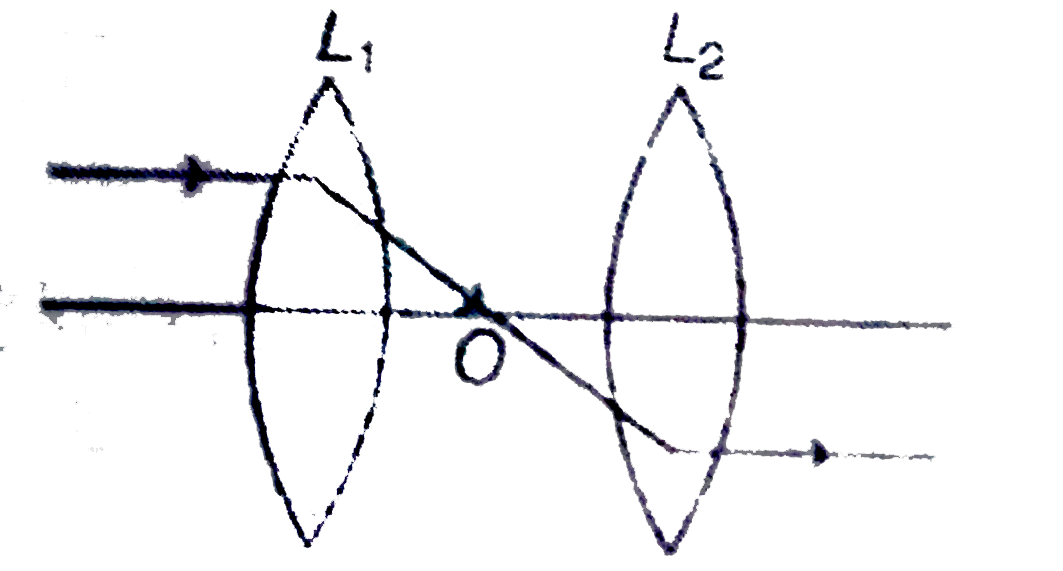
View SolutionIn the figure shown, there are two convex lenses L1 and L2 having focal. Lengths f1 and f2 respectively. The distance between L1 and L2 will be
View SolutionA line L lies in the plane 2x−y−z=4 such that it is perpendicular to the line x−22=y−31=z−45. The line L passes through the point of intersection of the given line and given plane. Which of the following points does not satisfy line L?
View Solution