Form the quadratic equation from the roots given below.
(iv)2−√5,2+√5Solution in Marathi
The correct Answer is:0
Step by step video & image solution for Form the quadratic equation from the roots given below. (iv)2-sqrt(5),2+sqrt(5) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- QUADRATIC EQUATIONSBOOK - BAL BHARTICHAPTER - QUADRATIC EQUATIONSEXERCISE - Practice Set 2.6
8 Videos
- QUADRATIC EQUATIONSBOOK - BAL BHARTICHAPTER - QUADRATIC EQUATIONSEXERCISE - Problem Set - 2 (Choose the correct answers)
8 Videos
- QUADRATIC EQUATIONSBOOK - BAL BHARTICHAPTER - QUADRATIC EQUATIONSEXERCISE - Practice Set 2.4
10 Videos
- PYTHAGORAS THEOREM BOOK - BAL BHARTICHAPTER - PYTHAGORAS THEOREM EXERCISE - PRACTICE SET 2
27 Videos
- SIMILARITY BOOK - BAL BHARTICHAPTER - SIMILARITY EXERCISE - PROBLEM SET 1
12 Videos
Similar Questions
Product of Pragati's age 2 years ago and 3 years hence is 84. Find her present age.
View SolutionWhich one is the quadratic equation?
View SolutionCompare the given quadratic equation to the general form and write values of a, b, c: x2−7x+5=0
View SolutionWhich of the following is a Pythagorean triplet?
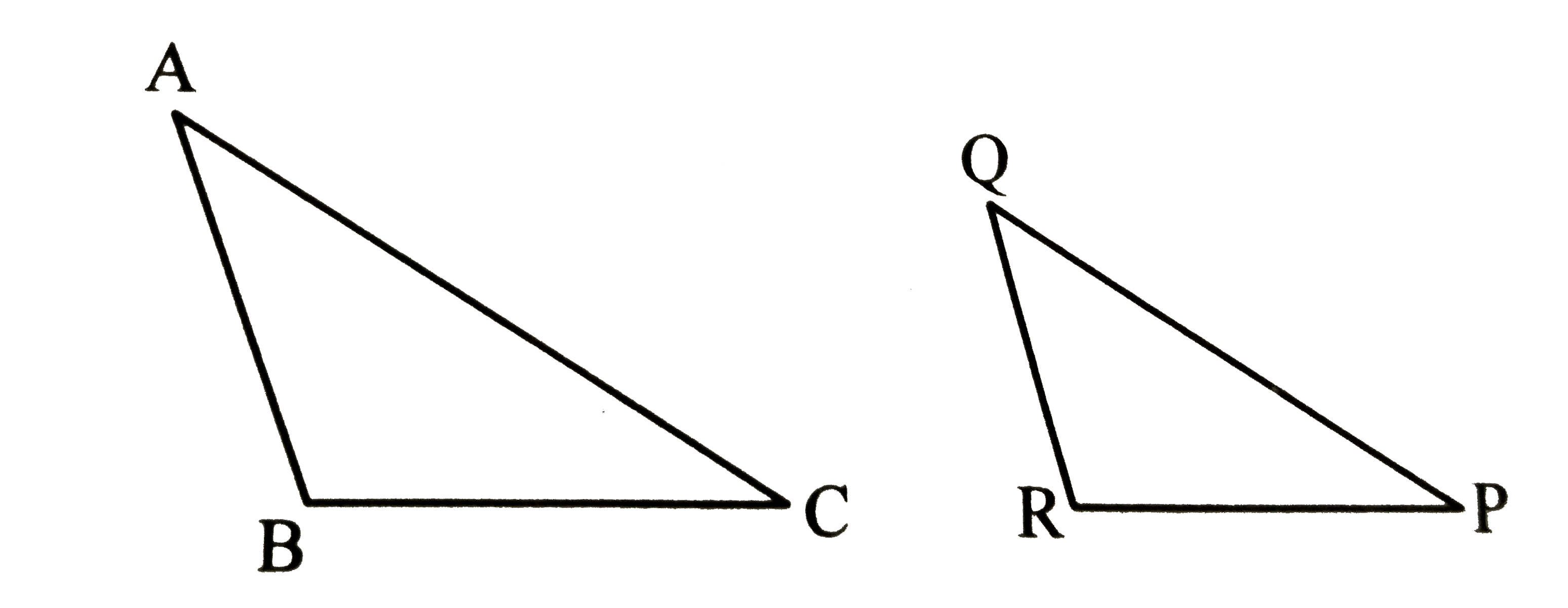
View SolutionIn ΔABCandΔPQR, in a one to one correspondence.
ABQR=BCPR=CAPQ, then
View SolutionConstruct the quadratic equation with following given roots (7+sqrt(5))/(7) and (7-sqrt(5))/(7)
View SolutionIf one root is 3+sqrt(5), then quadratic equation will be:
View SolutionSolve the equation by using the general expression for roots of a quadratic equation: √5x2+x+√5=0
View Solutionवे द्विघात बहुपद ज्ञात कीजिये जिनके मूल निम्न है :
(i) 3−√35,3+√35
(ii) 5+√25−√2,5−√25+√2
View SolutionFind the quadratic equation, if one of the roots is √5−√3 and other is √5+√3.
View SolutionForm the quadratic equation if its root are 2−√5,2+√5
View SolutionForm the quadratic equation from the roots given below.
2−√5 and 2+√5
View SolutionThe product of the roots of the quadratic equation√2x2−3x+5√2=0
View SolutionThe quadratic equation whose one root is 3+√52−√5 is
View SolutionWrite the quadratic equation if the roots are: 2−√5and2+√5
View SolutionForm the quadratic equation from the roots given below.
2−√5 and 2+√5
View Solution
