From a point P, 10cm away from the centre of a circle, a tangent PT of length 8cm is drawn. Find the radius of the circle.
To solve the problem, we will use the Pythagorean theorem. Let's break down the solution step by step:
Step 1: Understand the Geometry
We have a circle with center O, a point P outside the circle, and a tangent PT drawn from point P to the circle. The length of the tangent PT is given as 8 cm, and the distance OP from point P to the center O is given as 10 cm. We need to find the radius OT of the circle.
Step 2: Identify the Right Triangle
In triangle OTP, we can identify:
- OP as the hypotenuse (10 cm)
- PT as one leg of the triangle (8 cm)
- OT as the other leg, which is the radius we need to find.
Step 3: Apply the Pythagorean Theorem
According to the Pythagorean theorem:
OP2=OT2+PT2
Substituting the known values:
102=OT2+82
Step 4: Calculate the Squares
Calculating the squares:
100=OT2+64
Step 5: Solve for OT^2
Now, we isolate OT^2:
OT2=100−64
OT2=36
Step 6: Find OT
To find OT, we take the square root of both sides:
OT=√36
OT=6 cm
Conclusion
The radius of the circle is 6 cm.
---
We have a circle with center O, a point P outside the circle, and a tangent PT drawn from point P to the circle. The length of the tangent PT is given as 8 cm, and the distance OP from point P to the center O is given as 10 cm. We need to find the radius OT of the circle.
In triangle OTP, we can identify:
- OP as the hypotenuse (10 cm)
- PT as one leg of the triangle (8 cm)
- OT as the other leg, which is the radius we need to find.
According to the Pythagorean theorem:
OP2=OT2+PT2
Substituting the known values:
102=OT2+82
Calculating the squares:
100=OT2+64
Now, we isolate OT^2:
OT2=100−64
OT2=36
To find OT, we take the square root of both sides:
OT=√36
OT=6 cm
The radius of the circle is 6 cm.
Topper's Solved these Questions
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8A
16 Videos
- CIRCLESBOOK - RS AGGARWALCHAPTER - CIRCLESEXERCISE - Exercise 8B
15 Videos
- ARITHMETIC PROGRESSIONBOOK - RS AGGARWALCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Multiple Choice Questions (Mcq)
30 Videos
- CONSTRUCTIONSBOOK - RS AGGARWALCHAPTER - CONSTRUCTIONSEXERCISE - Test Yourself
10 Videos
Similar Questions
Knowledge Check
A point P is 25 cm from the centre of the circle. The length of the tangent drawn from P to the circle is 24 cm. Find the radius of the circle.
A5 cmB7 cmC6 cmD8 cmCalculate the distance (in cm) of the chord from the centre of the circle, if the chord of length 48 cm is drawn in the circle of radius 25 cm.
A7B14C49D56
A point P is 13cm from the centre of the circle. The length of the tangent drawn from P to the circle is 12 cm. Find the radius of the circle.
View SolutionA point is 26 cm away from the centre O of a circle and the length PT of the tangent drawn from P to the circle is 10 cm. Find the radius of the circle.
View SolutionType I: A point P is 13cm from the center of the circle. The length of the tangent drawn from P to the circle is 12 cm . Find the radius of the circle ?
View SolutionIf a point P is 17 cm from the centre of a circle of radius 8 cm, then find the length of the tangent drawn to the circle from point P.
View Solution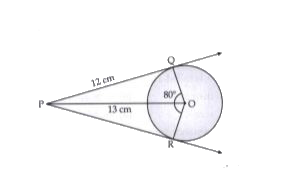
A point P is 13 cm from the centre of the circle. The two tangent PQ and PR are drawn from the point P, The length of the tangent drawn from P to the circle is 12 cm.
Find the radius of the circle.
View SolutionDraw a circle of radius 6 cm. From a point 10 cm away from its centre, construct the pair of tangents to the circle and measure their lengths.
View Solution
