From the bottom of a pole of height h, the angle of elevation of the top of a tower is α. The pole subtends an angle β at the top of the tower. find the height of the tower.
hcot(α−β)cot(α−β)−cotα
htan(α−β)tan(α−β)−tanα
cot(α−β)cot(α−β)−cotα
None of these
To find the height of the tower given the height of the pole and the angles of elevation, we can follow these steps:
Step 1: Understand the Geometry
We have a pole of height H and a tower. The angle of elevation from the bottom of the pole to the top of the tower is α. The pole subtends an angle β at the top of the tower.
Step 2: Set Up the Diagram
Let:
- O be the bottom of the pole.
- P be the top of the pole.
- Q be the top of the tower.
- A be the point directly below Q on the ground.
Step 3: Identify Angles
From the geometry:
- The angle ∠OQP=α (angle of elevation to the tower).
- The angle ∠PAQ=β (the angle subtended by the pole at the top of the tower).
- The angle ∠OAP=90∘ (the angle at point A).
Step 4: Calculate Angles in Triangle OQP
Using the angle sum property in triangle OQP:
∠OQP+∠PAQ+∠OAP=180∘
This gives:
α+β+90∘=180∘
Thus:
∠OQA=90∘−α
Step 5: Use Trigonometric Ratios
In triangle OQP:
tan(α)=PQOA
Let OA=x. Then:
PQ=xtan(α)
Step 6: Express PQ in Terms of H and QR
Since PQ=H+QR (where QR is the height of the tower above the pole), we can write:
H+QR=xtan(α)
Step 7: Analyze Triangle ARQ
In triangle ARQ:
tan(α−β)=QRx
Thus:
QR=xtan(α−β)
Step 8: Substitute QR Back
Substituting QR into the equation from Step 6:
H+xtan(α−β)=xtan(α)
Rearranging gives:
QR=xtan(α)−H
Step 9: Solve for x
From the equation tan(α−β)=QRx:
Substituting QR:
tan(α−β)=xtan(α)−Hx
Rearranging gives:
xtan(α−β)=xtan(α)−H
Thus:
x(tan(α)−tan(α−β))=H
So:
x=Htan(α)−tan(α−β)
Step 10: Substitute x Back to Find PQ
Now substituting x back into the equation for PQ:
PQ=Htan(α)−tan(α−β)tan(α)
Thus:
PQ=Htan(α)tan(α)−tan(α−β)
Final Expression for the Height of the Tower
The height of the tower QR can be expressed as:
QR=Htan(α)−tan(α−β)tan(α)−tan(α−β)
Final Answer
The height of the tower QR is:
QR=Htan(α)tan(α)−tan(α−β)
We have a pole of height H and a tower. The angle of elevation from the bottom of the pole to the top of the tower is α. The pole subtends an angle β at the top of the tower.
Let:
- O be the bottom of the pole.
- P be the top of the pole.
- Q be the top of the tower.
- A be the point directly below Q on the ground.
From the geometry:
- The angle ∠OQP=α (angle of elevation to the tower).
- The angle ∠PAQ=β (the angle subtended by the pole at the top of the tower).
- The angle ∠OAP=90∘ (the angle at point A).
Using the angle sum property in triangle OQP:
∠OQP+∠PAQ+∠OAP=180∘
This gives:
α+β+90∘=180∘
Thus:
∠OQA=90∘−α
In triangle OQP:
tan(α)=PQOA
Let OA=x. Then:
PQ=xtan(α)
Since PQ=H+QR (where QR is the height of the tower above the pole), we can write:
H+QR=xtan(α)
In triangle ARQ:
tan(α−β)=QRx
Thus:
QR=xtan(α−β)
Substituting QR into the equation from Step 6:
H+xtan(α−β)=xtan(α)
Rearranging gives:
QR=xtan(α)−H
From the equation tan(α−β)=QRx:
Substituting QR:
tan(α−β)=xtan(α)−Hx
Rearranging gives:
xtan(α−β)=xtan(α)−H
Thus:
x(tan(α)−tan(α−β))=H
So:
x=Htan(α)−tan(α−β)
Now substituting x back into the equation for PQ:
PQ=Htan(α)−tan(α−β)tan(α)
Thus:
PQ=Htan(α)tan(α)−tan(α−β)
The height of the tower QR can be expressed as:
QR=Htan(α)−tan(α−β)tan(α)−tan(α−β)
The height of the tower QR is:
QR=Htan(α)tan(α)−tan(α−β)
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives3videos
HIGHT AND DISTANCE
- BOOK - CENGAGE ENGLISHCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives3videos
HIGHT AND DISTANCE
- BOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - HYPERBOLAEXERCISE - COMPREHENSION TYPE2videos
HYPERBOLA
Similar Questions
From the top of a 7 m high building, the angle of elevation of the top of a tower is 60° and the angle of depression of the foot of the tower is 30°. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution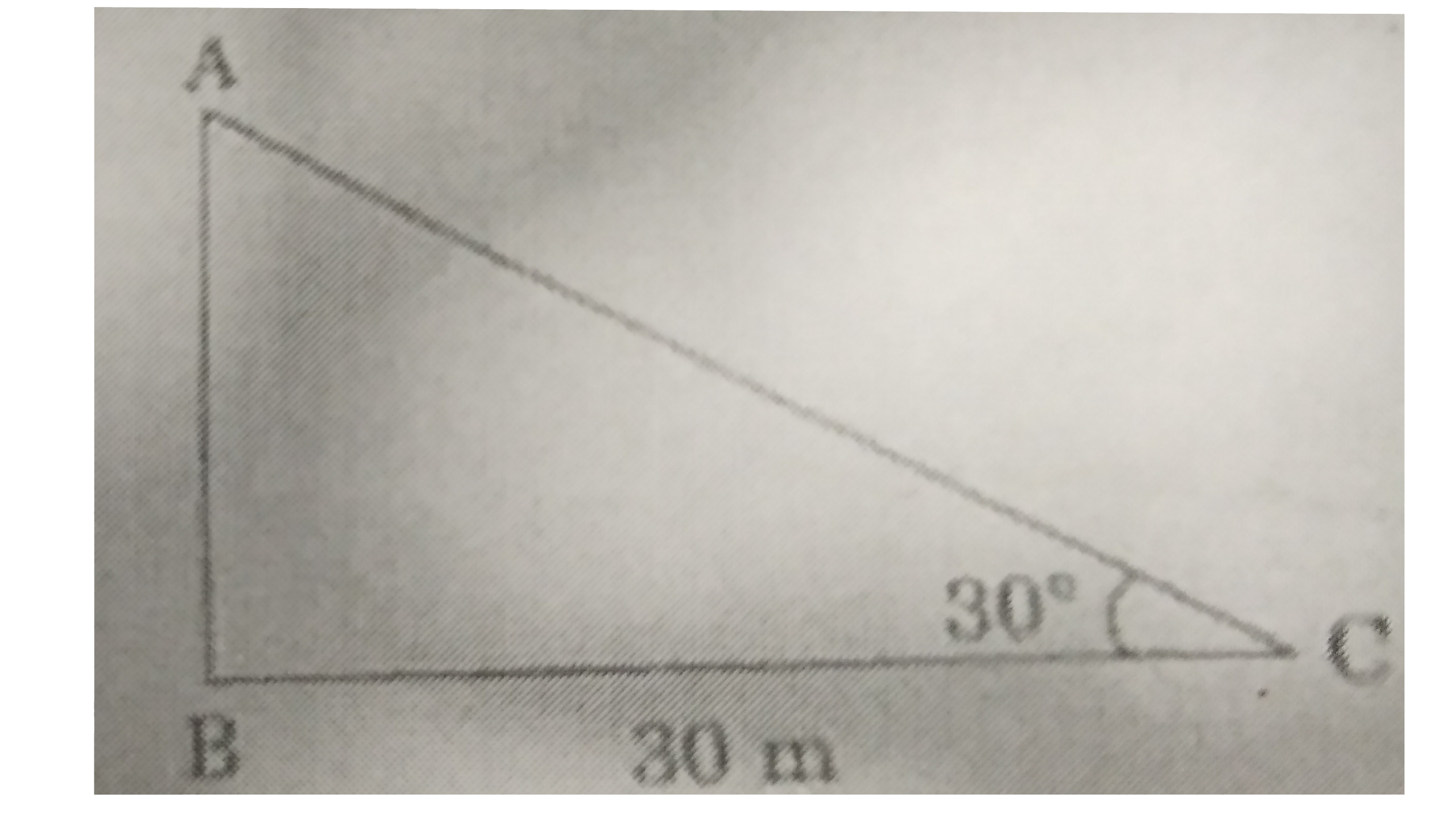
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.View SolutionA vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole the angle of elevation of the top of the tower is 60∘ and the angle of depression of the bottom of the tower is 30∘ . Find :
the height of the tower, it the height of the pole is 20 m ,View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.
 View Solution
View SolutionA vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole the angle of elevation of the top of the tower is 60∘ and the angle of depression of the bottom of the tower is 30∘ . Find :
the height of the pole, if the height of the tower is 75 mView Solution

