From the variable point A on circle x2+y2=2a2, two tangents are drawn to the circle x2+y2=a2 which meet the curve at BandC. Find the locus of the circumcenter of ABC.
The correct Answer is:x2+y2=a22
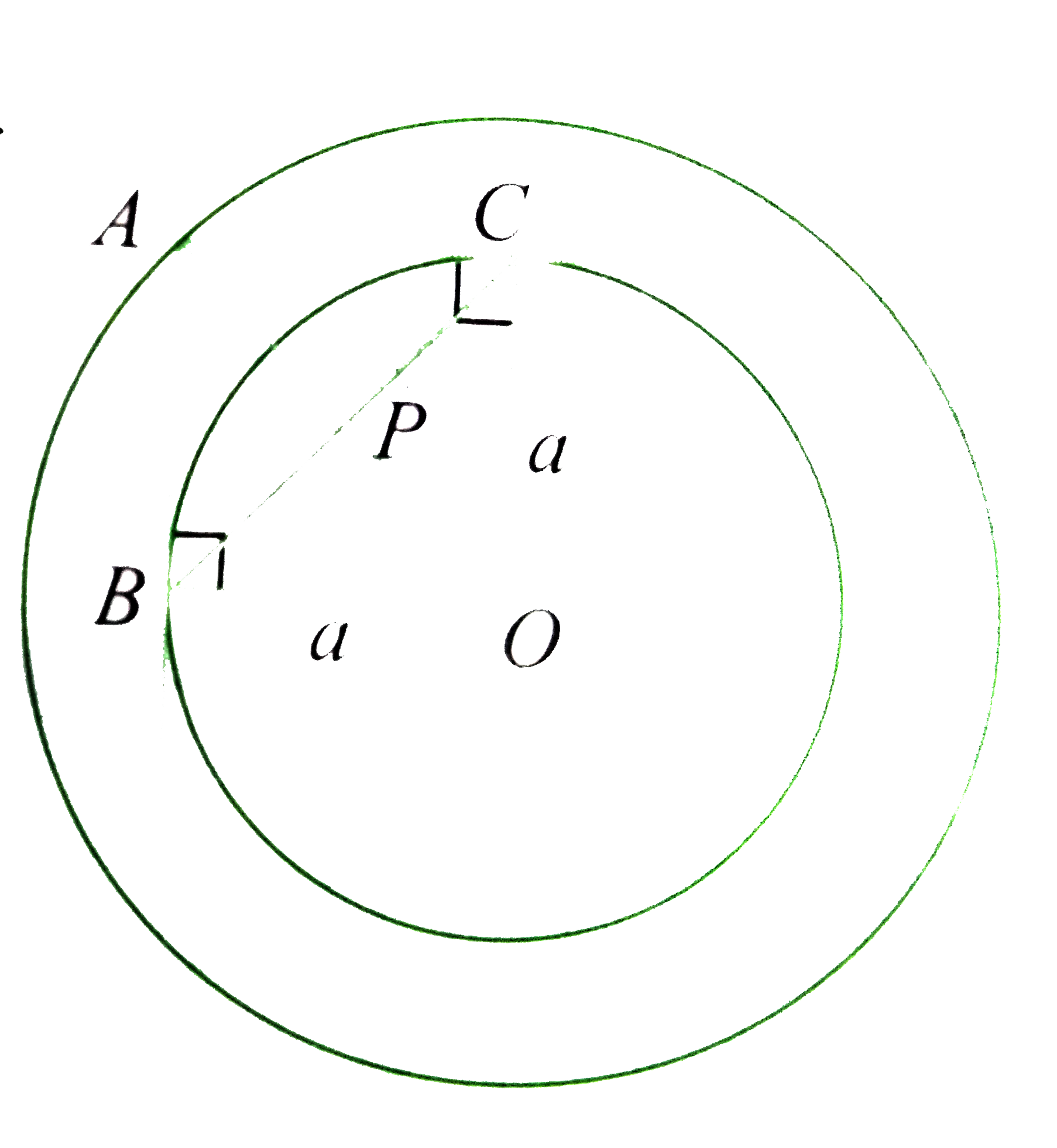
Clearly, x2+y2=2a2 is director circle of the circle x2+y2=a2.
Hence, in the diagram , ABOC is a square and circumcenter P(h,k) of ΔABC is the midpoint of OA.
Hence,
√h2+k2=√2a2
or the locus is
x2+y2=a22
Topper's Solved these Questions
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:Exercise 4.5
Explore 12 Videos
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:Exercise 4.6
Explore 5 Videos
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:Exercise 4.3
Explore 18 Videos
- BINOMIAL THEORMBook:CENGAGEChapter:BINOMIAL THEORMExercise:Question Bank
Explore 31 Videos
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Question Bank
Explore 32 Videos
Similar Questions
From the variable point A on circle x2+y2=2a2, two tangents are drawn to the circle x2+y2=a2 which meet the curve at BandC. Find the locus of the circumcenter of ABC.
View SolutionThe tangent at any point P on the circle x2+y2=4 meets the coordinate axes at AandB . Then find the locus of the midpoint of AB.
View Solution
Knowledge Check
From points on the circle x2+y2=a2 tangents are drawn to the hyperbola x2−y2=a2. Then, the locus of mid-points of the chord of contact of tangents is:
A(x2−y2)2=a2(x2+y2)B(x2−y2)=a2(x2+y2)C(x2−y2)=a2(x2+y2)2DNone of theseLocus of a point from which perpendicular tangents can be drawn to the circle x2+y2=a2 is
Acircle throụgh originBcircle of radius 2aCconcentric circle of radius a√2Dnone
From an arbitrary point P on the circle x2+y2=9 , tangents are drawn to the circle x2+y2=1 , which meet x2+y2=9 at AandB . The locus of the point of intersection of tangents at AandB to the circle x2+y2=9 is x2+y2=(277)2 (b) x2−y2(277)2 y2−x2=(277)2 (d) none of these
View SolutionIf from any point on the circle x2+y2=a2 tangents are drawn to the circle x2+y2=b2,(a>b) then the angle between tangents is
View SolutionThe locus of the point of intersection of the two tangents drawn to the circle x2+y2=a2 which include are angle α is
View SolutionFrom the points on the circle x2+y2=a2, tangents are drawn to the hyperbola x2−y2=a2: prove that the locus of the middle-points (x2−y2)2=a2(x2+y2)
View SolutionTwo perpendicular tangents to the circle x2+y2=a2 meet at P. Then the locus of P has the equation
View SolutionTwo perpendicular tangents to the circle x2+y2=a2 meet at P. Then the locus of P has the equation
View Solution