Give the venn diagram for the truth of the following statement "Equivalent triangles are isosceles triangles.
To solve the problem of creating a Venn diagram for the statement "Equivalent triangles are isosceles triangles," we can follow these steps:
Step 1: Define the Sets
- Let Set A represent the set of all Equilateral Triangles. An equilateral triangle is defined as a triangle where all three sides are equal.
- Let Set B represent the set of all Isosceles Triangles. An isosceles triangle is defined as a triangle with at least two sides equal.
Step 2: Analyze the Relationship
- All equilateral triangles are also isosceles triangles because if all three sides are equal, then at least two sides are equal. Therefore, Set A (Equilateral Triangles) is a subset of Set B (Isosceles Triangles).
- However, not all isosceles triangles are equilateral, as isosceles triangles can have two sides equal and the third side different.
Step 3: Draw the Venn Diagram
1. Draw two overlapping circles.
2. Label the left circle as Set A (Equilateral Triangles).
3. Label the right circle as Set B (Isosceles Triangles).
4. The area where Set A overlaps with Set B represents the equilateral triangles, which are also isosceles triangles.
5. The remaining area of Set B (Isosceles Triangles) represents those isosceles triangles that are not equilateral.
Step 4: Conclusion
- The Venn diagram visually represents that all equilateral triangles are included within the isosceles triangles, confirming the statement "Equivalent triangles are isosceles triangles."
- Let Set A represent the set of all Equilateral Triangles. An equilateral triangle is defined as a triangle where all three sides are equal.
- Let Set B represent the set of all Isosceles Triangles. An isosceles triangle is defined as a triangle with at least two sides equal.
- All equilateral triangles are also isosceles triangles because if all three sides are equal, then at least two sides are equal. Therefore, Set A (Equilateral Triangles) is a subset of Set B (Isosceles Triangles).
- However, not all isosceles triangles are equilateral, as isosceles triangles can have two sides equal and the third side different.
1. Draw two overlapping circles.
2. Label the left circle as Set A (Equilateral Triangles).
3. Label the right circle as Set B (Isosceles Triangles).
4. The area where Set A overlaps with Set B represents the equilateral triangles, which are also isosceles triangles.
5. The remaining area of Set B (Isosceles Triangles) represents those isosceles triangles that are not equilateral.
- The Venn diagram visually represents that all equilateral triangles are included within the isosceles triangles, confirming the statement "Equivalent triangles are isosceles triangles."
Topper's Solved these Questions
- MATHEMATICAL REASONINGBook:ML KHANNAChapter:MATHEMATICAL REASONINGExercise:PROBLEM SET (1)(MULTIPLE CHOICE QUESTIONS)
Explore 29 Videos
- MATHEMATICAL REASONINGBook:ML KHANNAChapter:MATHEMATICAL REASONINGExercise:PROBLEM SET (1)(FILL IN THE BLANKS)
Explore 22 Videos
- LOGARITHMS AND SURDSBook:ML KHANNAChapter:LOGARITHMS AND SURDSExercise:Miscellaneous exercise
Explore 3 Videos
- MATRICESBook:ML KHANNAChapter:MATRICESExercise:COMPREHENSION
Explore 3 Videos
Similar Questions
Knowledge Check
Which of the following cannot be the measures of an isosceles triangle ABC?
AAngle A is 50∘ and angle B is 80∘BAngle A is 65∘ and angle B is 50∘CAngle A is 60∘ and angle B is 70∘DAll of theseNumber of line of symmetry in a right angled isosceles triangle is
A0B1C2D3
Symmetry of an isosceles triangle
View SolutionIn the figure given below, ABC is a triangle with AB = BC and D is an interior point of the triangle ABC such that ∠DAC=∠DCA.
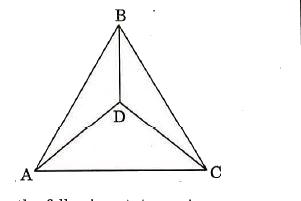
Consider the following statements :
1. Triangle ADC is an isosceles triangle.
2. Dis the centroid of the triangle ABC.
3. Triangle ABD is congruent to the triangle CBD.
Which of the above statements are correct ?
View SolutionLines of symmetry of an isosceles triangle
View SolutionLines of symmetry of an isosceles triangle
View SolutionIf one angle of a triangle is equal to the sum of the other two angles, then the triangle is an isosceles triangle (b) an obtuse triangle an equilateral triangle (d) a right triangle
View SolutionDraw the appropriate Venn diagram for the following:
(A∩B)'
View Solution
