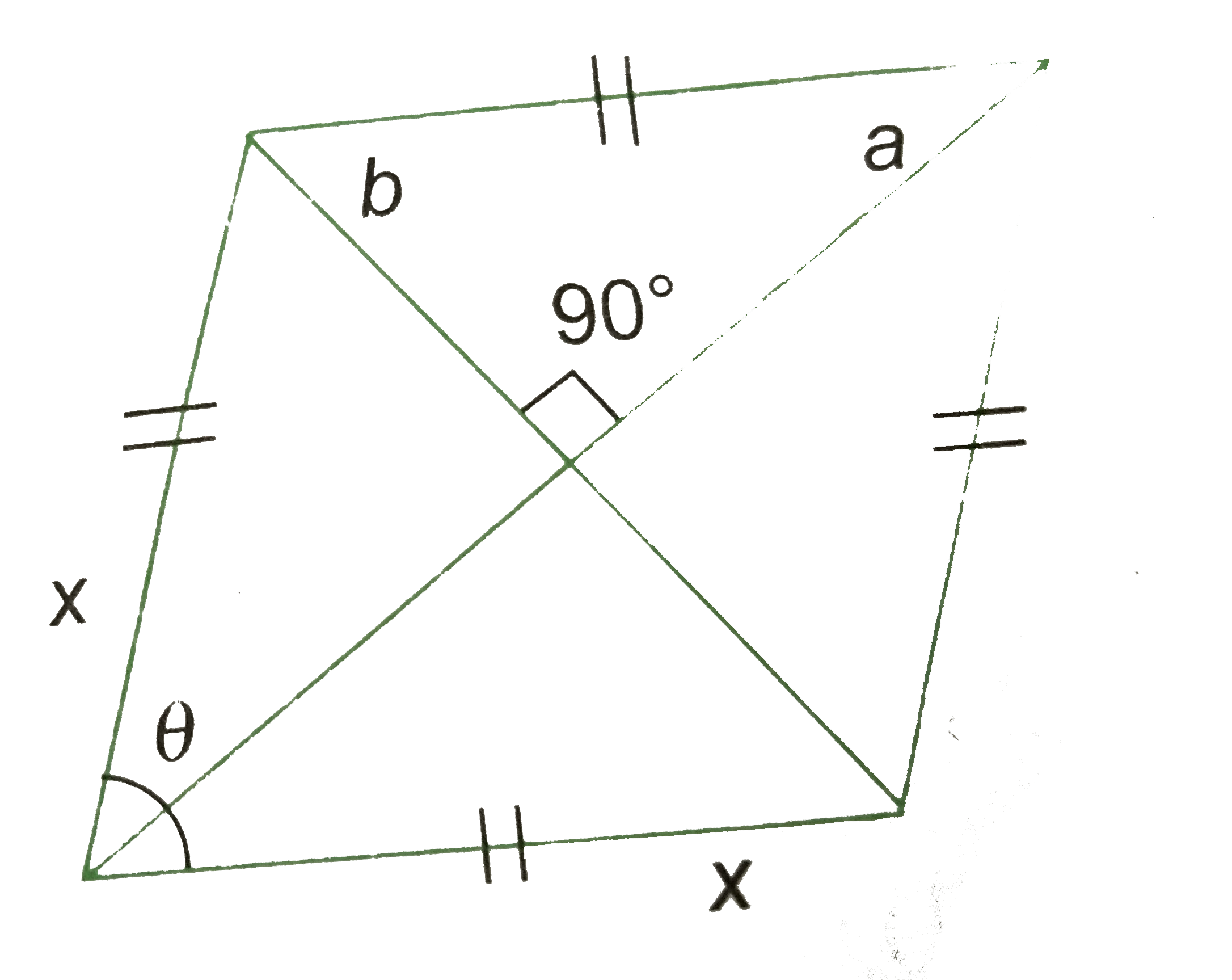
Given that the side length of a rhombus is the geometric mean of the length of its diagonals. The degree measure of the acute angle of the rhombus is 150 (b) 300 (c) 450 (d) 600Solution in Tamil
15∘
30∘
45∘
60∘
The correct Answer is:B
Area of rhomubs is given by
2×12x2sinθ=ab2 (Equating area of a rhombus)
Given x2=ab (given)
⇒sintη=12
orθ=30∘
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise (Multiple)17videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise (Comprehension)11videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Exercise 2.69videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)1videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGECHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type1videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
Similar Questions
If 0≤θ≤πand81sin2θ+81cos2θ=30 is
02:38View SolutionLet us consider the equation cos4xa+sin4xb=1a+b,x∈[0,π2],a,b>0
The value of sin2x in terms of a and b is02:56View SolutionProve that: tan7200−cos2700−s∈1500cos1200=14
07:05View SolutionFind the derivative of y=tan−12x3
07:56View SolutionRefer to the following diagram :

Column IColumn IIa.Collinear vectorsp.→ab.Coinitial vectorsq.→bc.Equal vectorsr.→cd.Unlike vectors (same initial point)s.→d04:43View SolutionGiven that the side length of a rhombus is the geometric mean of the length of its diagonals. The degree measure of the acute angle of the rhombus is (a)150 (b) 300 (c) 450 (d) 600
03:20View SolutionThe angle between sides of a rhombus whose v2xx sides is mean of its two diagonal,is equal to: a)30^(@)(b)45^(@)(c)60^(@)(d)90^(@)
01:32View SolutionThe acute angle of a rhombus whose side is a mean proportional between its diagonals is
13:07View SolutionThe area of the rhombus ABCD is 24. The equation of the diagonal BD is 4x + 3y + 2=0 and A (3,2) The length of the side of the rhombus is
02:45View SolutionGiven that the side length of a rhombus is the geometric mean of the length of its diagonals. The degree measure of the acute angle of the rhombus is (a)150 (b) 300 (c) 450 (d) 600
01:33View SolutionIf the hypotenuse of a right-angled triangle is four times the length of the perpendicular drawn from the opposite vertex to it, then the difference of the two acute angles will be 600 (b) 150 (c) 750 (d) 300
03:11View SolutionGiven that the side length of a rhombus is the geometric mean of the length of its diagonals. The degree measure of the acute angle of the rhombus is 150 (b) 300 (c) 450 (d) 600
04:57View SolutionThe acute angle of a rhombus whose side is a mean proportional between its diagonals is
08:03View SolutionA rhombus of side 20cm has two angles of 600 each. Find the length of the diagonals.
View SolutionIf two acute angles of a right triangle are equal, then each acute is equal to (a)300 (b) 450 (c) 600 (d) 900
01:30View SolutionGiven that the side length of a rhombus is the geometric mean of the lengths of its diagonals. The degree measure of the acute angle of the rhombus is
02:54View Solution

