How you would find the surface area of an open-top box that is shaped like a rectangular prism.
To find the surface area of an open-top box shaped like a rectangular prism (cuboid), follow these steps:
1. Identify the Dimensions:
- Let the length of the box be l.
- Let the breadth (width) of the box be b.
- Let the height of the box be h.
2. Understand the Faces of the Box:
- An open-top box has five faces:
- Two rectangular faces on the front and back (each with dimensions b×h).
- Two rectangular faces on the left and right (each with dimensions h×l).
- One rectangular face on the bottom (with dimensions l×b).
- The top face is open, so it is not included in the surface area calculation.
3. Calculate the Area of Each Face:
- Front and Back Faces:
- Area of one face = b×h
- Total area for both faces = 2×(b×h)=2bh
- Left and Right Faces:
- Area of one face = h×l
- Total area for both faces = 2×(h×l)=2hl
- Bottom Face:
- Area of the bottom face = l×b
4. Combine the Areas:
- Total surface area of the open-top box = Area of front and back + Area of left and right + Area of bottom
- Therefore, the total surface area SA can be expressed as:
SA=2bh+2hl+lb
5. Final Formula:
- The formula for the surface area of an open-top rectangular prism (box) is:
SA=2bh+2hl+lb
- Let the length of the box be l.
- Let the breadth (width) of the box be b.
- Let the height of the box be h.
- An open-top box has five faces:
- Two rectangular faces on the front and back (each with dimensions b×h).
- Two rectangular faces on the left and right (each with dimensions h×l).
- One rectangular face on the bottom (with dimensions l×b).
- The top face is open, so it is not included in the surface area calculation.
- Front and Back Faces:
- Area of one face = b×h
- Total area for both faces = 2×(b×h)=2bh
- Left and Right Faces:
- Area of one face = h×l
- Total area for both faces = 2×(h×l)=2hl
- Bottom Face:
- Area of the bottom face = l×b
- Total surface area of the open-top box = Area of front and back + Area of left and right + Area of bottom
- Therefore, the total surface area SA can be expressed as:
SA=2bh+2hl+lb
- The formula for the surface area of an open-top rectangular prism (box) is:
SA=2bh+2hl+lb
Topper's Solved these Questions
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - TRY THIS
5 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
Similar Questions
Knowledge Check
What is the formula for finding the surface area of an open cylinder with radius of base 'r' and height 'h'?
A2πrhBπr2hCπr(2h+r)D2πr(h+r)If the box is opened from the top, then the surface area of the open box with maximum volume is:
A32009cm2B35009cm2C46009cm2D16009cm2The average revenues of 11 consecutive years of a company is rs.77 lakhs. if the average of first 6 years is rs.72 lakhs and that of last 6 years is rs.84 lakhs. What is the revenue for the sisth year.
Aa. 91lakhsBb. 87lakhsCc. 89lakhsDd.none of these
The total surface area of open cuboidal box is ___________.
View SolutionThe adjacent figure shows a rectangular prism of length l units, width w units and height h units.
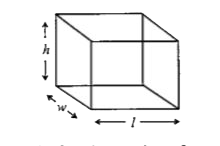
The formula for the total surface area of the rectangular prism is
View SolutionRead the following text and answer the following questions on the basis of the same :
Rohan went to a cricket stadium which is in rectangular shape. A circular green ground for playing cricket is inscribed in a rectangular shaped stadium of breadth 100 m and length 250 m

Find the area of rectangular shaped stadium
View SolutionExplain how the surface area of a box is changed if each dimension is multiplied by a factor of 3.
View SolutionA box contains 420 coins in 1 rupee, 50 paisa and 20 paisa coins, the ratio of their rupee values being 13: 11 : 7. The number of 50 paisa coins is
View Solution
