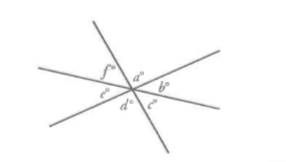
If 2s−e=32and2e−d=10, what is the average of d and e?
The correct Answer is:21
To solve the problem, we have the following two equations:
1. 2d−e=32 (Equation 1)
2. 2e−d=10 (Equation 2)
We need to find the average of d and e, which is given by the formula:
Average=d+e2
Step 1: Solve the equations simultaneously
Let's add the two equations together to eliminate one of the variables.
From Equation 1:
2d−e=32
From Equation 2:
2e−d=10
Adding these two equations:
(2d−e)+(2e−d)=32+10
Step 2: Simplify the left side
Combine like terms:
2d−d+2e−e=32+10
This simplifies to:
d+e=42
Step 3: Find the average of d and e
Now that we have d+e=42, we can find the average:
Average=d+e2=422=21
Final Answer
The average of d and e is 21.
---
2. 2e−d=10 (Equation 2)
2d−e=32
2e−d=10
(2d−e)+(2e−d)=32+10
2d−d+2e−e=32+10
d+e=42
Average=d+e2=422=21
Topper's Solved these Questions
Similar Questions
Knowledge Check
The average of a, b, c, d, and e is 28. If the average of a, c, and e is 24, what is the average of b and d?
A31B32C33D34Suppose that the average (arithmetic mean) of a, b and c is h, the average of b, c, and d is j, and the average of d and e is k.
What is the average of a and e?Ah−j+kB3h+3j−2k2C3h−3j+2k2D3h−3j+2k5
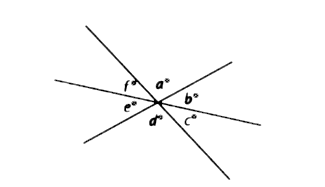
If a is 95, what is b+d−e ?
View SolutionIn Figure, DE∣∣BC, if AD=x,DB=x−2,AE=x+2 and EC=x−1, find the value of x.
View SolutionIn Fig 31, ABCD is a rectangle with dimensions 32 m by 18 m. ADE is a triangle such that EF⊥AD and EF=14 cm. Calculate the area of the shaded region.
View SolutionIf sinθ=a2−b2a2+b2, find the value of tanθ, secθ and cosecθ
View SolutionIf A is the area and 2s is the sum of the sides of a triangle, then A≤s24 (b) A≤s23√3 2RsinAsinBsinC (d) noneofthese
View SolutionIf a,b,c,d,e are +ve real numbers such that a+b+c+d+e=8anda2+b2+c2+d2+e2=16, then the range of 'e' is
View Solution