If 4m+8, 2m2+3m+6,3m2+4m+4 are three consecutive terms of an A.P. Find m.
To solve the problem, we need to find the value of m such that the expressions 4m+8, 2m2+3m+6, and 3m2+4m+4 are three consecutive terms of an Arithmetic Progression (A.P.).
1. Understanding the Condition for A.P.:
For three terms a1,a2,a3 to be in A.P., the condition is:
a2−a1=a3−a2
This means the difference between the second and first term should equal the difference between the third and second term.
2. Assign the Terms:
Let:
- a1=4m+8
- a2=2m2+3m+6
- a3=3m2+4m+4
3. Set Up the Equation:
According to the A.P. condition:
a2−a1=a3−a2
Substituting the values of a1,a2, and a3:
(2m2+3m+6)−(4m+8)=(3m2+4m+4)−(2m2+3m+6)
4. Simplify the Left Side:
2m2+3m+6−4m−8=2m2−m−2
5. Simplify the Right Side:
3m2+4m+4−2m2−3m−6=m2+m−2
6. Set the Two Sides Equal:
Now we have:
2m2−m−2=m2+m−2
7. Rearranging the Equation:
Move all terms to one side:
2m2−m−2−m2−m+2=0
This simplifies to:
m2−2m=0
8. Factoring the Equation:
Factor out m:
m(m−2)=0
9. Finding the Values of m:
Setting each factor to zero gives:
m=0orm=2
10. Verification:
- For m=0:
- a1=4(0)+8=8
- a2=2(0)2+3(0)+6=6
- a3=3(0)2+4(0)+4=4
- The terms are 8,6,4 which do not form an A.P.
- For m=2:
- a1=4(2)+8=16
- a2=2(2)2+3(2)+6=16
- a3=3(2)2+4(2)+4=16
- The terms are 16,16,16 which do form an A.P.
Final Answer:
The values of m that satisfy the condition are m=0 and m=2, but only m=2 gives valid consecutive terms in A.P.
For three terms a1,a2,a3 to be in A.P., the condition is:
a2−a1=a3−a2
This means the difference between the second and first term should equal the difference between the third and second term.
Let:
- a1=4m+8
- a2=2m2+3m+6
- a3=3m2+4m+4
According to the A.P. condition:
a2−a1=a3−a2
Substituting the values of a1,a2, and a3:
(2m2+3m+6)−(4m+8)=(3m2+4m+4)−(2m2+3m+6)
2m2+3m+6−4m−8=2m2−m−2
3m2+4m+4−2m2−3m−6=m2+m−2
Now we have:
2m2−m−2=m2+m−2
Move all terms to one side:
2m2−m−2−m2−m+2=0
This simplifies to:
m2−2m=0
Factor out m:
m(m−2)=0
Setting each factor to zero gives:
m=0orm=2
- For m=0:
- a1=4(0)+8=8
- a2=2(0)2+3(0)+6=6
- a3=3(0)2+4(0)+4=4
- The terms are 8,6,4 which do not form an A.P.
- For m=2:
- a1=4(2)+8=16
- a2=2(2)2+3(2)+6=16
- a3=3(2)2+4(2)+4=16
- The terms are 16,16,16 which do form an A.P.
The values of m that satisfy the condition are m=0 and m=2, but only m=2 gives valid consecutive terms in A.P.
Topper's Solved these Questions
- ARITHMETIC PROGRESSION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ARITHMETIC PROGRESSION EXERCISE - SHORT ANSWER TYPE QUESTIONS-II
17 Videos
- ARITHMETIC PROGRESSION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ARITHMETIC PROGRESSION EXERCISE - LONG ANSWER TYPE QUESTIONS
14 Videos
- ARITHMETIC PROGRESSION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ARITHMETIC PROGRESSION EXERCISE - STATE TRUE/FALSE AND JUSTIFY
5 Videos
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - PRACTICE-TEST ( AREAS RELATED TO CIRCLES ) SECTION-D
1 Videos
- CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - CIRCLESEXERCISE - PRACTICE - TEST (SECTION - D)
1 Videos
Similar Questions
Knowledge Check
Masses m,2m, 3m, 4m,………,12m are kept on the peripheri of a clock having a circular dial. The masses are kept such that m is kept at 1,2miskeptat2,3mat3………12mat12.vec g is the gravitational field intensity at the centre of dial.
AAt 9:30 the hour hand is in the direction of →gBAt 3:30 the hour hand is in the direction of →gCthere will be no component of →g along hour hand at 6:30DThere will be no component of →g along hour hand at 12:30Subtract the sum of (8m+7n+6p2) and (−3m−4n−p2) from the sum of (2m+4n−3p2) and (−m−n−p2).
A4m+9p2B4m−6n+9p2C−4m+6n−9p2D4m−9p2
Three point masses m1=2Kg,m2=4kgandm3=6kg are kept at the three corners of an equilateral triangle of side 1 m. Find the locationo of their center of mass.
View SolutionIf m+1m−2=4, find
m2+m+1=?
View SolutionIf m+1m+2=−4 , find
m2+m+1=?
View SolutionIf in an A.P., Sn=n2p and Sm=m2p , where Sr denotes the sum of r terms of the A.P., then Sp is equal to 12p3 (b) mn p (c) p3 (d) (m+n)p2
View SolutionThe four particles of masses m,3m,2m and 4m are placed on the vertices of a sqare of side L. Locate the center of mass
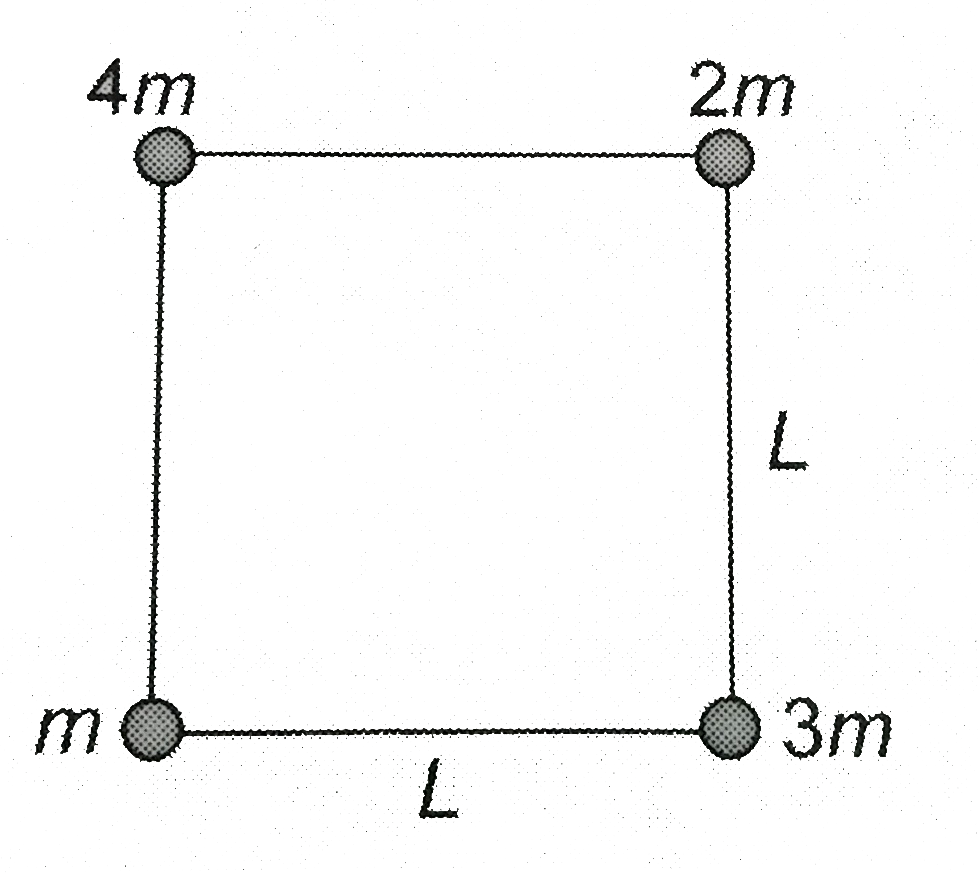
View SolutionFour particles of masses m,2m,3m,4m are placed at corners of a square of side 'a' as shown in fig. Find out coordinateds of centre of mass.
View Solution
