If A and B are two independent events such that P(¯¯¯A∩B)=2/15 and P(A∩¯¯¯B)=1/6, then P(B), is
15 or ,45
16 or,56
45 or,16
56 or,15
The correct Answer is:C
To solve the problem step by step, we need to find the probability of event B, given the probabilities of the intersections of events A and B.
Step 1: Define the probabilities
Let:
- P(A)=x
- P(B)=y
Since A and B are independent events, we have:
- P(A∩B)=P(A)⋅P(B)=x⋅y
Step 2: Use the given information
We are given:
1. P(¯¯¯¯A∩B)=215
2. P(A∩¯¯¯¯B)=16
Using the properties of probabilities, we can express these in terms of x and y:
- P(¯¯¯¯A)=1−P(A)=1−x
- P(¯¯¯¯B)=1−P(B)=1−y
Thus, we can write:
1. P(¯¯¯¯A∩B)=P(¯¯¯¯A)⋅P(B)=(1−x)⋅y=215
2. P(A∩¯¯¯¯B)=P(A)⋅P(¯¯¯¯B)=x⋅(1−y)=16
Step 3: Set up the equations
From the above expressions, we can set up the following equations:
1. (1−x)⋅y=215 (Equation 1)
2. x⋅(1−y)=16 (Equation 2)
Step 4: Solve Equation 1 for y
From Equation 1:
y−xy=215
Rearranging gives:
y(1−x)=215
Thus,
y=215(1−x)(1)
Step 5: Substitute y in Equation 2
Now substitute y from Equation (1) into Equation 2:
x⋅(1−215(1−x))=16
Simplifying the left side:
x⋅(15(1−x)−215(1−x))=16
This simplifies to:
x⋅15−15x−215(1−x)=16
x⋅13−15x15(1−x)=16
Step 6: Cross-multiply and simplify
Cross-multiplying gives:
6x(13−15x)=15(1−x)
Expanding both sides:
78x−90x2=15−15x
Rearranging gives:
90x2−93x+15=0
Step 7: Solve the quadratic equation
Using the quadratic formula x=−b±√b2−4ac2a:
Here, a=90,b=−93,c=15:
x=93±√(−93)2−4⋅90⋅152⋅90
Calculating the discriminant:
=8649−5400=3249
x=93±57180
Calculating the two possible values for x:
1. x=150180=56
2. x=36180=15
Step 8: Find y for both values of x
Using y=215(1−x):
1. For x=56:
y=215(1−56)=215⋅16=2⋅615=1215=45
2. For x=15:
y=215(1−15)=215⋅45=2⋅515⋅4=1060=16
Conclusion
Thus, the possible values for P(B) are 45 and 16.
Let:
- P(A)=x
- P(B)=y
- P(A∩B)=P(A)⋅P(B)=x⋅y
We are given:
1. P(¯¯¯¯A∩B)=215
2. P(A∩¯¯¯¯B)=16
- P(¯¯¯¯A)=1−P(A)=1−x
- P(¯¯¯¯B)=1−P(B)=1−y
1. P(¯¯¯¯A∩B)=P(¯¯¯¯A)⋅P(B)=(1−x)⋅y=215
2. P(A∩¯¯¯¯B)=P(A)⋅P(¯¯¯¯B)=x⋅(1−y)=16
From the above expressions, we can set up the following equations:
1. (1−x)⋅y=215 (Equation 1)
2. x⋅(1−y)=16 (Equation 2)
From Equation 1:
y−xy=215
Rearranging gives:
y(1−x)=215
Thus,
y=215(1−x)(1)
Now substitute y from Equation (1) into Equation 2:
x⋅(1−215(1−x))=16
Simplifying the left side:
x⋅(15(1−x)−215(1−x))=16
This simplifies to:
x⋅15−15x−215(1−x)=16
x⋅13−15x15(1−x)=16
Cross-multiplying gives:
6x(13−15x)=15(1−x)
Expanding both sides:
78x−90x2=15−15x
Rearranging gives:
90x2−93x+15=0
Using the quadratic formula x=−b±√b2−4ac2a:
Here, a=90,b=−93,c=15:
x=93±√(−93)2−4⋅90⋅152⋅90
Calculating the discriminant:
=8649−5400=3249
x=93±57180
Calculating the two possible values for x:
1. x=150180=56
2. x=36180=15
Using y=215(1−x):
1. For x=56:
y=215(1−56)=215⋅16=2⋅615=1215=45
2. For x=15:
y=215(1−15)=215⋅45=2⋅515⋅4=1060=16
Thus, the possible values for P(B) are 45 and 16.
Topper's Solved these Questions
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Section- II (Assertion -Reason Types MCQs)
15 Videos
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Section I - Mcqs
89 Videos
- PROBABILITY BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PROBABILITY EXERCISE - Chapter Test
45 Videos
- PERMUTATIONS AND COMBINATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Chapter Test
60 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test
50 Videos
Similar Questions
If A and B are two independent events such that P (A) =1/2,P(B)=1/5, then
View SolutionA and B are two independent events such that P(A)=15,P(A∪B)=710. Then ,P(¯¯¯B)=
View SolutionIf A and B are two independent events such that P(¯¯¯A∩B)=2/15 and P(A∩¯¯¯B)=1/6, then P(B) is
View SolutionIf A and B are two independent events, such that P(A)=13andP(B)=14.
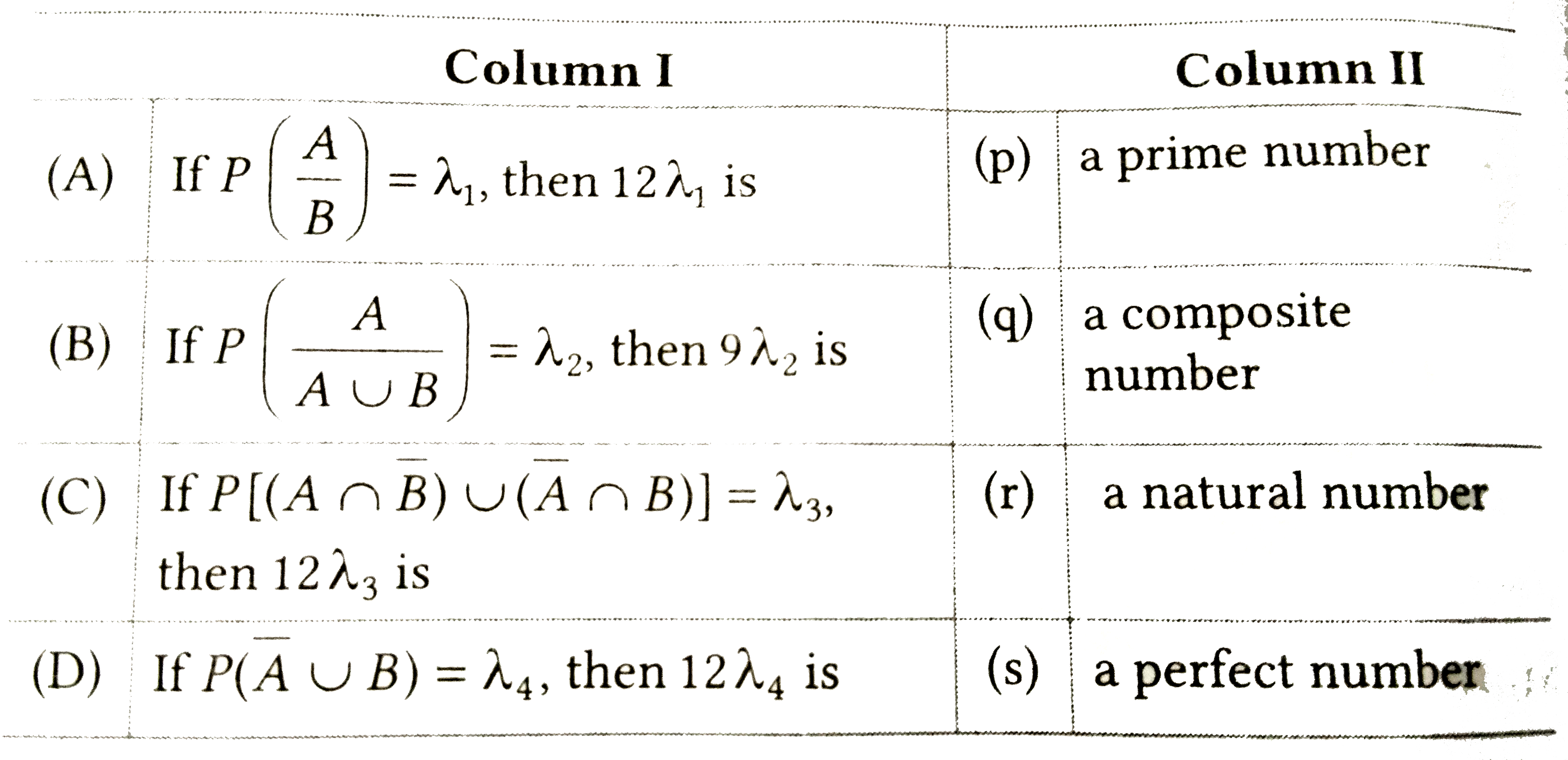
View SolutionIf A and B are two independent events such that P(¯¯¯A∩B)=215andP(A∩¯¯¯B)=16 then P(B)=
View SolutionIf A and B are two independent events such that P(A∪B)=2/15 and P(A∪B)=1/6 , then find P(B).
View SolutionIf A and B are two independent events, then P( A and B)=P(A)⋅P(B)
View SolutionIf AandB are two independent events such that P(B)=27,,P(A∪B)=0.8 then P(A)=
View SolutionIf A and B are two independent events such that P(¯¯¯A)=710,P(¯¯¯B)=α andP(A∪B)=810, then α, is
View SolutionA and B are two independent events such that P(A) = 0.8 and P(A∩→B)=0.3 Find P(B)
View Solution
