If ABCD is a rhombus with ∠ABC=56∘, find the measure of ∠ACD
To solve the problem, we need to find the measure of angle ∠ACD in the rhombus ABCD given that ∠ABC=56∘.
1. Understand the Properties of a Rhombus:
- In a rhombus, opposite angles are equal, and adjacent angles are supplementary (they add up to 180∘).
- Thus, if ∠ABC=56∘, then ∠ADC=56∘ as well.
Hint: Remember that in a rhombus, opposite angles are equal.
2. Find the Measure of Angle ∠BCD:
- Since adjacent angles in a rhombus are supplementary, we can find ∠BCD using:
∠ABC+∠BCD=180∘
Substituting the known value:
56∘+∠BCD=180∘
∠BCD=180∘−56∘=124∘
Hint: Use the property of supplementary angles to find the adjacent angle.
3. Identify the Relationship Between Angles:
- In triangle ABC, since AB∥CD and AC is a transversal, we can say:
∠ACD=∠ABC
- This is because alternate interior angles are equal when a transversal crosses parallel lines.
Hint: Recall the alternate interior angles theorem when dealing with parallel lines.
4. Conclusion:
- Therefore, we have:
∠ACD=∠ABC=56∘
Final Answer:
The measure of ∠ACD is 62∘.
- In a rhombus, opposite angles are equal, and adjacent angles are supplementary (they add up to 180∘).
- Thus, if ∠ABC=56∘, then ∠ADC=56∘ as well.
- Since adjacent angles in a rhombus are supplementary, we can find ∠BCD using:
∠ABC+∠BCD=180∘
Substituting the known value:
56∘+∠BCD=180∘
∠BCD=180∘−56∘=124∘
- In triangle ABC, since AB∥CD and AC is a transversal, we can say:
∠ACD=∠ABC
- This is because alternate interior angles are equal when a transversal crosses parallel lines.
- Therefore, we have:
∠ACD=∠ABC=56∘
The measure of ∠ACD is 62∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
In a triangle ABC, if ∠A>∠B>∠C and the measures of ∠A,∠B and ∠C in degrees are integers, then the least possible value of ∠A is
A70∘B65∘C60∘D61∘ - Question 2 - Select One
In a cyclic □ ABCD, twice the measure of ∠A is thrice the measure of ∠C . Find the measure of ∠C.
A36∘B72∘C90∘D108∘
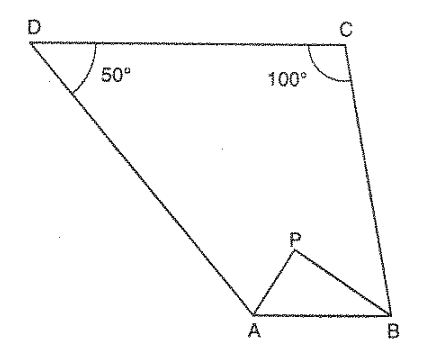
In Figure, the bisectors of ∠A and ∠B meet at a point P. If ∠C=1000 and ∠D=500, find the measure of ∠APB
 View Solution
View SolutionIn a parallelogram ABCD, if ∠D=115∘, then write the measure of ∠A
View SolutionIn a quadrilateral ABCD, bisectors of angles A and B intersect at O such that ∠AOB=75∘, then write the value of ∠C+ ∠D
View SolutionIn a parallelogram ABCD,∠D=115∘, determine the measure of ∠A and ∠B.
View SolutionIn a parallelogram ABCD, ∠D=1350, determine the measure of ∠A and ∠B.
View SolutionIn a parallelogram ABCD,∠D=135∘, determine the measures of ∠Aand∠B
View Solution

