If a vertex of a triangle is $(1, 1)$ , and the middle points of two sides passing through it are $- 2, 3)$ and $(5, 2),$ then find the centroid and the incenter of the triangle.

Video Solution

Text Solution

Verified by Experts

Let E and F be the midpoints of AB and AC. Let the coordinates of B and C be $(α, β)$ and $(γ, δ)$ respectively. Then $- 2 = \frac{1 + α}{2}, 3 = \frac{1 + β}{2}$ ,
$5 = \frac{1 + γ}{2}, 2 = \frac{1 + δ}{2}$
$∴ α = - 5, β = 5, γ = 9, δ = 3$

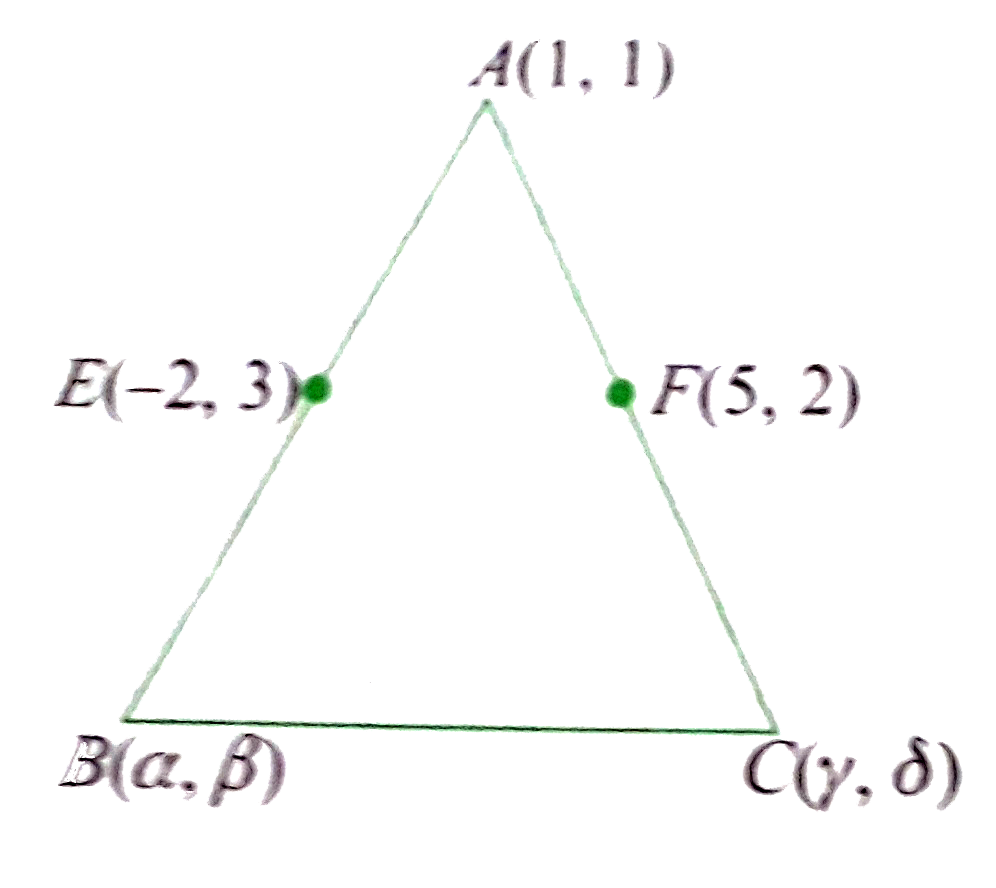
Therefore, the coordinates of B and C are $(- 5, 5)$ and $(9, 3)$ , respectively.
Then the centroid is
$(\frac{1 - 5 + 9}{3}, \frac{1 + 5 + 9}{3}) \equiv (\frac{5}{3}, 3)$
and $a = B C = \sqrt{{(- 5 - 9)}^{2} + {(5 - 3)}^{2}} = 10 \sqrt{2}$
$b = C A = \sqrt{{(9 - 1)}^{2} + {(3 - 1)}^{2}} = 2 \sqrt{17}$
$c = A B = \sqrt{{(1 + 5)}^{2} + {(1 - 5)}^{2}} = 2 \sqrt{13}$
Then, the incenter is
$(\frac{10 \sqrt{2} (1) + 2 \sqrt{17} (- 5) + 2 \sqrt{13} (9)}{10 \sqrt{2} + 2 \sqrt{17} + 2 \sqrt{13}}, \frac{10 \sqrt{2} (1) + 2 \sqrt{17} (5) + 2 \sqrt{13} (3)}{10 \sqrt{2} + 2 \sqrt{17} + 2 \sqrt{13}})$
i.e., $(\frac{5 \sqrt{2} - 5 \sqrt{17} + 9 \sqrt{13}}{5 \sqrt{2} + \sqrt{17} + \sqrt{13}}, \frac{5 \sqrt{2} + 5 \sqrt{17} + 3 \sqrt{13}}{5 \sqrt{2} + \sqrt{17} + \sqrt{13}})$

Updated on:21/07/2023