If a,b,c are in AP, then show that r1,r2,r3 are in HP.
To show that if a,b,c are in Arithmetic Progression (AP), then the reciprocals r1,r2,r3 are in Harmonic Progression (HP), we can follow these steps:
Step 1: Understanding AP
Given that a,b,c are in AP, we know that:
b−a=c−b
This can be rearranged to:
2b=a+c
Step 2: Expressing in terms of S
Let’s introduce a variable S such that:
S=a+b+c
From the property of AP, we can express S in terms of a,b,c:
S−a=b+c
S−b=a+c
S−c=a+b
Step 3: Forming new terms
Now, we can form the terms S−a,S−b,S−c:
- S−a=b+c
- S−b=a+c
- S−c=a+b
Step 4: Showing the new terms are in AP
Since a,b,c are in AP, the terms S−a,S−b,S−c are also in AP. This means:
(S−b)−(S−a)=(S−c)−(S−b)
This simplifies to:
b−a=c−b
Step 5: Relating to R1, R2, R3
Next, we express the reciprocals in terms of R1,R2,R3:
Let:
R1=1S−a,R2=1S−b,R3=1S−c
Thus, we have:
1R1=S−a,1R2=S−b,1R3=S−c
Step 6: Showing the reciprocals are in HP
Since S−a,S−b,S−c are in AP, it follows that:
1R1,1R2,1R3 are in AP
This implies that:
R1,R2,R3 are in HP
Conclusion
Thus, we have shown that if a,b,c are in AP, then R1,R2,R3 are in HP.
Given that a,b,c are in AP, we know that:
b−a=c−b
This can be rearranged to:
2b=a+c
Let’s introduce a variable S such that:
S=a+b+c
From the property of AP, we can express S in terms of a,b,c:
S−a=b+c
S−b=a+c
S−c=a+b
Now, we can form the terms S−a,S−b,S−c:
- S−a=b+c
- S−b=a+c
- S−c=a+b
Since a,b,c are in AP, the terms S−a,S−b,S−c are also in AP. This means:
(S−b)−(S−a)=(S−c)−(S−b)
This simplifies to:
b−a=c−b
Next, we express the reciprocals in terms of R1,R2,R3:
Let:
R1=1S−a,R2=1S−b,R3=1S−c
Thus, we have:
1R1=S−a,1R2=S−b,1R3=S−c
Since S−a,S−b,S−c are in AP, it follows that:
1R1,1R2,1R3 are in AP
This implies that:
R1,R2,R3 are in HP
Thus, we have shown that if a,b,c are in AP, then R1,R2,R3 are in HP.
Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 4
10 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 5
7 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 2
20 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
If in ΔABC,(a−b)(s−c)=(b−c)(s−a), prove that r1,r2,r3 are in A.P.
View SolutionIn a ΔABC, show that r1⋅r2⋅r3⋅r=Δ2.
View SolutionIn a triangle ABC if sides a = 13, b =14 and c = 15, then reciprocals of r1,r2,r3 are in the ratio
View SolutionAssertion: a2,b2,c2 are in A.P., Reason: 1b+c,1c+a,1a+b are in A.P. (A) Both A and R are true and R is the correct explanation of A (B) Both A and R are true R is not te correct explanation of A (C) A is true but R is false. (D) A is false but R is true.
View SolutionShow that r1+r2=ccot(C2)
View SolutionIf the numbers p,q,r are in A.P. then m7p,m7q,m7r(m>0) are in (A) A.P. (B) G.P. (C) H.P. (D) none of these
View SolutionStatement I In a ΔABC,ifa<b<c and r is inradius and r1,r2,r3 are the exradii opposite to angle A,B,C respectively, then r<r1<r2<r3.
Statement II For, ΔABCr1r2+r2r3+r3r1=r1r2r3r
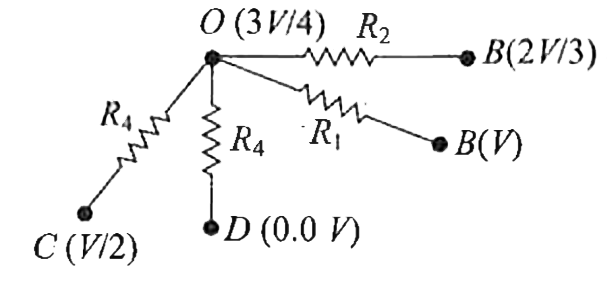
View SolutionThe circuit is shown in the following figure. The potential at points A, B, C, D and O are given. The currents in the resistance R1,R2 and R3 are in the ratio of 4:2:1. What is the ratio of resistance R1,R2,R3 and R4 ?
View SolutionShow that 16R2rr1r2r3=a2b2c2
View SolutionProve that
r21+r22+r33+r2=16R2−a2−b2−c2.
where r= in radius, R = circumradius,, r1,r2,r3 are ex-radii.
View Solution